pdf file

The composition is concatenation of tangles, followed by a rescaling to the interval [0, 1].
The identity tangles are given by parallel lines.
2. We endow T with a monoidal structure. On objects, we define k⊗l := k+l; on morphisms,
we take juxtaposition of tangles. The tensor unit is 0 ∈ Z ≥0 .
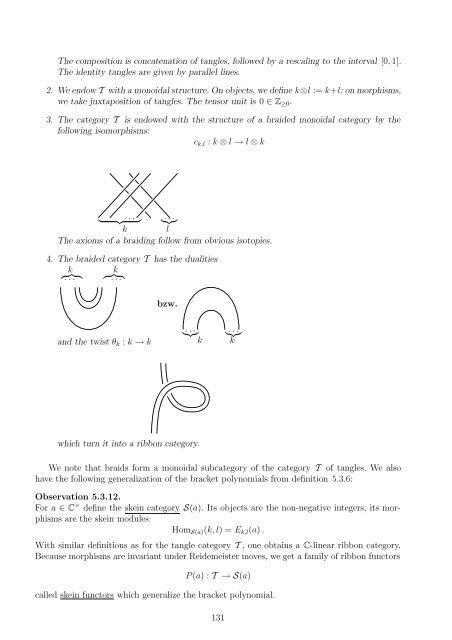
3. The category T is endowed with the structure of a braided monoidal category by the
following isomorphisms:
c k,l : k ⊗ l → l ⊗ k
} {{. . . } }{{} . . .
k l
The axioms of a braiding follow from obvious isotopies.
4. The braided category T has the dualities
{ }}
k
. . . { { }}
k
. . .{
bzw.
and the twist θ k : k → k
}{{} . . . }{{} . . .
k k
which turn it into a ribbon category.
We note that braids form a monoidal subcategory of the category T of tangles. We also
have the following generalization of the bracket polynomials from definition 5.3.6:
Observation 5.3.12.
For a ∈ C × define the skein category S(a). Its objects are the non-negative integers, its morphisms
are the skein modules
Hom S(a) (k, l) = E k,l (a) .
With similar definitions as for the tangle category T , one obtains a C-linear ribbon category.
Because morphisms are invariant under Reidemeister moves, we get a family of ribbon functors
P (a) : T → S(a)
called skein functors which generalize the bracket polynomial.
131