pdf file

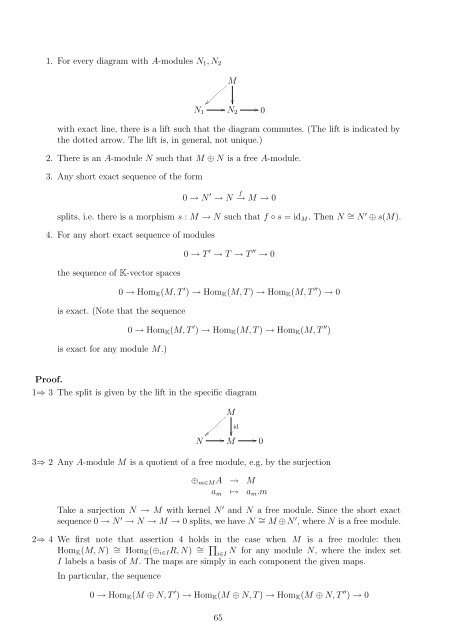
1. For every diagram with A-modules N 1 , N 2
M
N 1 N 2 0
with exact line, there is a lift such that the diagram commutes. (The lift is indicated by
the dotted arrow. The lift is, in general, not unique.)
2. There is an A-module N such that M ⊕ N is a free A-module.
3. Any short exact sequence of the form
0 → N ′ → N f → M → 0
splits, i.e. there is a morphism s : M → N such that f ◦ s = id M . Then N ∼ = N ′ ⊕ s(M).
4. For any short exact sequence of modules
the sequence of K-vector spaces
0 → T ′ → T → T ′′ → 0
0 → Hom K (M, T ′ ) → Hom K (M, T ) → Hom K (M, T ′′ ) → 0
is exact. (Note that the sequence
is exact for any module M.)
0 → Hom K (M, T ′ ) → Hom K (M, T ) → Hom K (M, T ′′ )
Proof.
1⇒ 3 The split is given by the lift in the specific diagram
M
id
N M 0
3⇒ 2 Any A-module M is a quotient of a free module, e.g. by the surjection
⊕ m∈M A → M
a m ↦→ a m .m
Take a surjection N → M with kernel N ′ and N a free module. Since the short exact
sequence 0 → N ′ → N → M → 0 splits, we have N ∼ = M ⊕ N ′ , where N is a free module.
2⇒ 4 We first note that assertion 4 holds in the case when M is a free module: then
Hom K (M, N) ∼ = Hom K (⊕ i∈I R, N) ∼ = ∏ i∈I
N for any module N, where the index set
I labels a basis of M. The maps are simply in each component the given maps.
In particular, the sequence
0 → Hom K (M ⊕ N, T ′ ) → Hom K (M ⊕ N, T ) → Hom K (M ⊕ N, T ′′ ) → 0
65