CHAPTER 4. THERMODYNAMICS: THE FIRST LAW

4-1
CHAPTER 4. THERMODYNAMICS: THE FIRST LAW
A. Introduction
Thermodynamics deals with the macroscopic observable properties of matter. It is a subject
that is broadly applicable to all energetic processes involving large aggregates of atoms and
molecules.
Thermodynamics is founded upon three fundamental laws of nature. The first of these laws
is the familiar law of conservation of energy, which in general terms states that the energy of the
universe is constant. As with other natural laws, the validity and generality of the laws of
thermodynamics have been firmly established by experimental observation. One of the major
strengths of thermodynamics is that it does not rely on any assumptions regarding the fundamental
structure of matter.
Thermodynamics plays a major role in chemistry. It provides the theoretical basis for
equilibrium as well as for most discussions of the energetics of chemical reactions. One of the
primary applications of thermodynamics to chemistry is in providing the criteria for predicting
whether a particular chemical reaction will proceed spontaneously.
In the study of thermodynamics, it is necessary to divide the universe into the particular
region in which we are interested, called the system, and everything else, called the surroundings.
Usually, the system is separated from the surroundings by some kind of physical boundary. If this
boundary is such that both matter and energy may be transferred between the system and the
surroundings, the system is called an open system. If the boundary prevents the transfer of matter,
but allows the transfer of energy, the system is called a closed system. A system for which neither
the transfer of matter or energy is possible is called an isolated system. For example, a thermos
bottle with the top screwed on is an isolated system because it is thermally insulated from the
surroundings.
B. State functions
In order to specify the macroscopic state of a system, it is necessary to know the values of
various parameters called state functions, such as temperature, pressure, volume, etc. When a
system changes from one state to another, the final values of the state functions do not depend on

4-2
how the change of state was carried out. Therefore, the changes in the values of the state functions
are determined only by the initial and final states of the system. For example, consider the four states
of a system composed of an ideal gas, as depicted in Fig. 4-1.
path 1
State 2
P 2
= 2 atm
V 2
= 22 L
T 2
= 546 K
State 1
P 1
= 1 atm
V 1
= 22 L
T 1
= 273 K
path 3
State 4
P 4
= 2 atm
V 4
= 11 L
T 4
= 273 K
path 2
State 3
P 3
= 1 atm
V 3
= 11 L
T 3
= 136 K
Figure 4-1. Transformation of a system from state 1 to state 4 via three different paths.
Three different paths lead from state 1 to state 4. However, the changes in all three of the state
functions listed (P, V, and T) are the same for each path. Going from state 1 to state 4 via path 1,
path 2, or path 3 results in P = 1 atm, V = 11 L, and T = 0 K. In other words, the final value
of a state function does not depend on the path taken between the initial and final state. On
the other hand, suppose that instead of different states of a system, the above (state) labels
represented different cities on a map and the three paths represented different routes between them.
It is obvious that distance is not a state function since the distance between city 1 and city 4 depends
on the route taken. In the city analogy, is altitude a state function? Of course distance is not a
thermodynamic variable, but two very important thermodynamic variables that are not state
functions are work and heat.
C. Work and heat

is
=
4-3
Mechanical work is defined as a force applied in the direction of motion times the distance
moved (displacement). Specifically,
dw ' PF@ Pds ' Fdscosθ
,
where θ
the angle between the force vector and the displacement vector. An example of the
application of this formula is the calculation of the work done in raising a mass m to a height h
against the force of gravity:
F = ma = mg
dw = Fds cos θ
mgds
w '
h
0
mgds ' mgh.
A particularly important form of work in chemical thermodynamics is the work associated
with a pressure-volume change. The diagram in Fig. 4-2 shows a cross sectional view of a piston
undergoing a compression.
Figure 4-2. A piston system undergoing a compression.
The applied force can be expressed as an external pressure times the cross sectional area of the
piston; F = P A. Therefore,
ex
but
dw = Fds = P Ads, ex

4-4
and so
Ads = dV,
dw = P dV. ex
The thermodynamic sign convention is that work done on the system is positive. To conform to this
convention, the above expression for P-V work must include a negative sign since, work was done
on the system, but because V < V , dV was negative. Therefore, the general expression for
2 1
differential P/V work is
.
If an expansion (compression) is carried out with a constant external pressure, then the work
done by (on) the system is
V 2
dw ' !
V 1
P ex
dV ' !P ex
(V 2
! V 1
) ' !P ex
V
.
Another way of performing a compression would be to keep the temperature constant and make the
external pressure infinitesimally greater than the internal pressure throughout the process. This is
called an isothermal reversible compression. The term reversible is derived from the fact that the
process could be reversed by applying an infinitesimal change in the opposite direction. Assuming
ideal behavior of the gas inside the system, the external pressure in this case is
P = P = nRT/V,
ex
(i.e., neglecting the infinitesimal difference, the external pressure is equal to the internal pressure for
a reversible compression or expansion). Therefore, the work done on the system is
dw ' !
V 2
V 1
P ex
dV ' !nRT
V 2
dV
V ' !nRTln V 2
V
V 1
1
.
P-V diagrams are useful for analyzing processes involving P-V work. A P-V diagram for two

expansion processes which start at P , V and end at P , V are shown in Fig. 4-3.
1 1 2 2
4-5
P 1
a
path 1
b
dw = -PdV
P
path 2
c
P 2
V 1 V
V 2
Figure 4-3.
A P-V diagram for two expansion processes between the same initial and final values
of P and V.
Path 1 consists of a constant pressure expansion from point a to point b followed by a reduction in
pressure at constant volume from point b to point c. Path 2 is an isothermal reversible expansion (P in
= P ) from point a to point c (shown by the hyperbolic curve or isotherm). As indicated by the
ex
shaded region labeled P dV, the differential work is represented by the area of a rectangle having
1
length P and width dV. Therefore, the total work associated with path 1 is equal to the area of the
rectangle having length P and width V ! V , while the work associated with path 2 is equal to the
1 2 1
area under the hyperbolic curve. Clearly these two areas are not the same even though both
processes involved the same changes in pressure and volume. One must conclude, therefore, that
work is not a state function.
Another way in which energy can be exchanged between systems is by heat flow. A system
at a higher temperature may exchange energy with a system at a lower temperature, thereby lowering
its own temperature and raising the temperature of the other system in the process. Since the same
change in state can be achieved by either heat or work, one must conclude that like work, heat is not
a state function.

4-6
Exercise:
Calculate the work for each of the following constant temperature processes;
a). Expansion due to a sudden pressure change from P ex to P ex/4.
b). Expansion in two steps- from P to P /2, then from P /2 to P /4.
ex ex ex ex
c). Reversible expansion from P ex to P ex/4.
What do you conclude about a reversible expansion?
a). w = ! P V; V = V (P /P ) = 4V so V = 3V and
2 1 1 2 1 1
w = ! (P /4)(3V ) = !0.75P V .
1 1 1 1
b). w = ! 0.5P V = !0.5P V (V = 2V )
1 1 1 1 2 1
w = ! 0.25P V = !0.5P V (V = 4V )
2 1 1 1 2 1
w = w + w = ! P V .
1 2 1 1
c). w = ! nRT ln (V /V ) = ! P V ln 4 = !1.39P V .
2 1 1 1 1 1
As can be seen from this exercise, the reversible work is the maximum possible work
that can be done by the system or the surroundings in a given process.
D. The first law of thermodynamics
Since heat added to a system must result in a change of the internal energy content of the
system, or it must produce work, or both, one is led to expect that these quantities must be intimately
related. The first law of thermodynamics expresses the fact that (a) heat and/or work are involved
in the transfer of internal energy from one system to another, and (b) the total internal energy of a
system must be conserved;
The first Law.
In the first law equation above, the quantity U represents the internal energy, which consists of the
kinetic and potential energies of all the atoms and molecules of the system. Unlike heat (q) and work
(w), internal energy is a state function. If this were not true, it would be possible to construct a
cyclic process going from state a to state b that released U and then returned back to state a using
1
U , such that | U | < | U |. This is the condition for a perpetual motion machine. It is important
2 2 1
to understand the thermodynamic sign conventions as they apply to the first law. Namely,

4-7
when heat is added to a system, q is positive,
when work is done on a system, w is positive.
According to the first law, when heat is transferred to or from a closed system in a process
that does no work, the heat involved is equal to the internal energy change. Specifically, for a
constant volume process
where
but
and so
dU = dq + dw,
dw = ! P dV, ext
dV = 0
at constant volume.
In general, when only P-V work is involved,
dq = dU + P dV. ext
Therefore, it is useful to define a new quantity (H), called enthalpy, such that
,
where P is the pressure of the system. Since U, P, and V are all state functions, H is a state function
also. A differential change in H may be expressed as
When P is constant,
dH = dU + PdV + VdP.
dH = dU + PdV,
and if P = P (i.e., when the system is in mechanical equilibrium with the surroundings),
ext

4-8
Therefore,
dH = dU + P dV. ext
at constant pressure.
For liquids and solids, (PV) is usually very small (typically - 0.1% of U), and hence H
. U. For ideal gases,
H = U + PV = U + nRT,
so
H = U + nRT
at constant temperature. It should be noted that n is the change in the number of moles of gas.
Exercise:
A total of 885.7 kJ of heat is evolved in the reaction
CH + 2O ! CO + 2H O
4(g) 2(g) 2(g) 2 (l)
o
Carried out at fixed volume and 25 C. Calculate U and H for this reaction.
U = q = !885.7 kJ
H = U + (PV) = U + nRT; n = 1 ! 3 = !2
!3
H = !885.7 ! 2(8.314)(298)x10 = !890.6 kJ
Exercise:
A 10.0 g sample of benzene at its boiling temperature (353.2 K) is placed in contact
with a resistance heater. It evaporates when 3942 J of heat have been produced by
the heater. Calculate the molar enthalpy change and the the molar internal energy
change. (Neglect the volume of the liquid).
This is a constant pressure process in which H = U + (PV) = q.
n = 10.0 g C H x 1 mol C H /78 g C H = 0.128 mol
6 6 6 6 6 6
H = 3942 J/0.128 mol = 30797 J/mol = 30.8 kJ/mol
m
U = H ! (PV ! PV )/n = H ! RT
m m gas liq m

4-9
!3
= 30.8 kJ/mol - (8.314x10 kJ/mol-K)(353.2 K) = 27.9 kJ/mol.
E. Heat capacity
The amount of heat released or absorbed in a process may be determined by measuring the
change in temperature of a mass in thermal contact with the process. The heat capacity is defined
as the change in heat divided by the change in temperature, C = dq/dT. However, this definition is
not very useful unless a path is specified, since the value of dq depends on how the process is carried
out. In the laboratory, there are two convenient paths; the constant volume path, where a reaction
is carried out in a closed container so that the volume cannot change, and the constant pressure path,
where a reaction is carried out in an open container so that the pressure remains constant at the
pressure of the surrounding atmosphere. Consider a process carried out at constant volume. Then
dw = 0 and so dq = dU, therefore, the heat capacity (per mole) at constant volume is
and
,
.
For a process carried out at constant pressure, dq = dH, therefore, the heat capacity (per mole) at
constant pressure is
,
and
.
Physically, the difference between C V and C P is related to the fact that when the volume is

4-10
held constant, all of the heat goes into producing a corresponding change in temperature (i.e., it all
goes into changing the internal energy), whereas when the pressure is held constant, some of the heat
goes into work of expansion or contraction. Therefore, it is to be expected that
since a given q results in a smaller T.
P
C P > CV
When a monatomic ideal gas is heated at constant volume, all of the heat goes into increasing
the translational kinetic energy of the atoms. However, in the case of molecules, there are other
energy modes available in addition to kinetic energy. Specifically, molecules may have rotational
and vibrational energy. Therefore, when a molecular gas is heated, the resulting energy increase is
distributed among all three energy modes. Statistically, it may be shown that the amount of energy
per mole that goes into each energy mode is
U m = N m(½RT),
where N is related to the number of degrees of freedom and the number of different types of energy
m
(e.g., kinetic and potential) associated with the energy mode, and R is the gas constant. This result,
which assumes that all energy modes have continuous energy distributions, is known as the
equipartition theorem. The number of degrees of freedom for a molecule, D , is related to the
m
number of coordinates required to specify the position of each atom. The degrees of freedom
associated with the various energy modes of a molecule and the corresponding values of N are m
summarized below;
N = D , N = D , N = 2D
tran tran rot rot vib vib
D = 3n tot
D = 3 tran
D = 2 (linear molecule) or 3 (nonlinear)
rot
D = 3n !5 (linear molecule) or 3n ! 6 (nonlinear)
vib
The equipartition theorem may be used to estimate the heat capacities of gas-phase molecules at high
temperatures.
Example: Estimate the value of C for CO .
V 2

4-11
In this case, n = 3 and so D = 9. This molecule is linear; therefore D = 2 and D
tot rot vib
= 9 ! 5 = 4. Hence U = U tran + U rot + U vib = 3(½RT) + 2(½RT) + 8(½RT) =
13/2 RT. The estimated heat capacity is therefore C V
' dU
dT ' 13 R ' 6.5 R. The
2
measured value at 298 K is 3.46 R.
Exercise: Estimate the value of C for CH .
V 4
In this case, n = 5 and so D tot = 15. This molecule is nonlinear; therefore D rot = 3 and
D vib = 15 ! 6 = 9. Hence U = U tran + U rot + U vib = 3(½RT) + 3(½RT) + 18(½RT) =
12RT. The estimated heat capacity is therefore C V
' dU ' 12 R. The measured
dT
value at 298 K is 3.25 R.
Because the energies of rotation and vibration are not really continuous, the values of C obtained
V
for molecules from the equipartition theorem are reached only at very high temperatures.
The relationship between C V and C P can be derived for an ideal gas as follows:
H = U + PV
MH
' MU % P MV
MT P
MT P
MT
P
,
but for an ideal gas, the internal energy only depends on temperature U = f(T) (recall that U = trans
(3/2)RT), and so
MU
' MU
MT P
MT
' C V
.
V
Also
V m
' RT
P , so MV m
MT
' R . Therefore
P
P
.
Exercise:
Determine the values of C V and C P for Ar assuming it to be an ideal gas.
U = (3/2)RT so C V = dU/dT = (3/2)R and C P = (5/2)R.

4-12
Exercise:
Draw a P-V diagram for one mole of a monatomic ideal gas initially at a pressure of
2.5 atm and a volume of 5.0 L undergoing the following: (a) the pressure is reduced
suddenly to 1.0 atm while the volume is held constant, (b) then, the volume expands
to 12.5 L while the pressure is held constant. Calculate U, H, q, and w for both
steps and for the isothermal, reversible expansion from the initial to the final state.
T = P V /R = 5(2.5)/0.08206 =152.3K
1 1 1
T = 5(1.0)/0.08206 = 60.9 K
2
T = T = 152.3 K
3 1
P (atm)
3.0
2.5
2.0
1.5
1.0
0.5
(5.0, 2.5)
A
C
B
(5.0, 1.0) (12.5, 1.0)
Step a:
U = C T = (3/2)(8.314)(!91.4)
V
= !1139.8 J
0.0
4 6 8 10 12 14
V (L)
H = U + (PV) = !1139.8 +[(1.0)(5.0)!(2.5)(5.0)] x 8.314/0.08206
= !1899.7 J Also H = C T = 5/2(8.314)(!91.4) = !1899.7 J (state func.)
P
w = 0, q = U
Step b:
H = C T = (5/2)(8.314)(91.9) = 1899.7 J
Step c:
q = H
P
w = !P V = !(1.0)(12.5 ! 5.0) x 8.314/0.08206 = !759.9 J
U = q + w = 1899.7 ! 759.9 = 1139.8 J Also U = C T = 3/2(8.314)(91.4)
V
U = 0
(T is constant)
H = U + (PV) = 0 (P V = P V )
1 1 2 2
w = !RT ln V /V = !(8,314)(152.3) ln (12.5/5.0) = !1160.2 J
2 1
q = !w
= 1139.8 J (state function)
(Note that both U and H are zero for the closed cycle 16 2 6 1).

F. Thermochemistry
4-13
A very important application of thermodynamics to chemistry is the study of the heat released
or absorbed in a chemical reaction. Chemical reactions are most commonly carried out at constant
pressure (i.e., in an open vessel) and for this specified path, the heat changes are the same as the
enthalpy changes. Since enthalpy is a state function, it is possible to calculate the change in enthalpy
accompanying a chemical reaction simply from a knowledge of the enthalpies of the reactants and
the enthalpies of the products. In actual, practice, enthalpies for chemical reactions may be
o
determined from tabulated standard enthalpy changes, denoted by the symbol H . The term
standard means that the enthalpy change is for a reaction in which the reactants and products are in
their so-called standard states.
Standard state:
the pure substance at a specified
temperature and a pressure of 1 bar.
Although enthalpy changes may be tabulated for any temperature, the most common tabulations are
for 298 K.
1. Enthalpy of formation
The most useful thermochemical tabulations give standard enthalpies of formation denoted
o
by the symbol fH .
Standard enthalpy of formation:
the enthalpy change accompanying the formation of a
substance in its standard state from the most stable forms of
the constituent elements of the substance in their standard
states.
For example, the standard enthalpy of formation for benzene is the enthalpy change for the reaction
6C + 3H ! C H .
(s,gr) 2(g) 6 6(l)

is
=
=
=
4-14
At 298 K and 1 bar, where the solid (graphite) is the most stable form of carbon, the diatomic gas
is the most stable form of hydrogen, and the liquid is the most stable form of benzene, the standard
o
o
enthalpy of formation of benzene is H (C H ) = 49.0 kJ/mol. Note that H is a molar quantity
f 6 6 f
and the formation reaction is always balanced such that one mole of product is formed. The
enthalpies of formation of all pure elements in their standard states are taken to be zero.
of formation:
o
Now consider the task of calculating H for the following reaction using standard enthalpies
(1) 2HN + 2NO ! H O + 4N .
3(l) (g) 2 2(l) 2(g)
We begin by writing the formation reactions for each of the (nonelemental) reactants and products
and looking up the standard enthalpies of formation;
o
(2) 3/2 N 2(g) + 1/2 H 2(g) ! HN 3(l) fH (HN 3) = 264.0 kJ/mol
o
(3) 1/2 N 2(g) + 1/2 O 2(g) ! NO (g) fH (NO) = 90.25 kJ/mol
o
(4) H 2(g) + O 2(g) ! H2O 2(l) fH (H2O 2) = !187.78 kJ/mol
Next, we rearrange the reactions in such a way that they add up to reaction (1);
Therefore,
reaction (1) = !2 x reaction (2) ! 2 x reaction (3) + reaction (4) .
o o o o
H = fH (H2O 2) ! 2 fH (HN 3) !2 fH (NO) = !896.28 kJ/mol.
This is an illustration of Hess's Law. The above procedure may be generalized and stated in a more
concise form by representing each of the stoichiometric coefficients of the net reaction by the symbol
ν, where i represents the reaction species and the sign of taken to be positive for products and
ν
i
negative for reactants. (For example, in reaction 1 above,
ν
i
1,
ν
!2, and
ν
1 2 3
!2). Then
.

4-15
Applying this formula directly to reaction (1) above gives
o o o o
H = fH (H2O 2) ! 2 fH (HN 3) !2 fH (NO) = !896.28 kJ/mol,
which is exactly the same as we obtained before.
Hess's law is a direct consequence of the fact that enthalpy is a state function; the enthalpy
change for a reaction is the same for any path that connects the reactants and products.
Exercise:
Construct a diagram for the above example showing the relationship between the
direct reaction path and the path involving the formation reactions.
2HN + 2NO !
H O + 4N
3 2 2 2
9 9 8
(3N + H ) + (N + O ) ! (4N + H + O ) .
2 2 2 2 2 2 2
Exercise:
Use the tabulated enthalpies of formation in your textbook to calculate the enthalpy
of combustion for phenol (C H OH).
6 5
C H OH + 7O ! 6CO + 3H O
6 5 (s) 2(g) 2(g) 2 (l)
o o o o
cH (C6H5OH) = 6 fH (CO 2) + 3 fH (H2O) ! fH (C6H5OH)
2. Enthalpies of physical change
= 6(!393.51) + 3(!285.83) ! (!165.0) = !3054 kJ/mol.
Other enthalpy changes of importance in chemistry are those that accompany transitions from
one physical form to another. The transitions of particular interest are defined below.
Transition Physical change Symbol for enthalpy change
Fusion solid to liquid H fus
Vaporization liquid to gas H vap
Sublimation solid to gas H sub
Hydration gaseous ions to ions H hyd
in solution
Solution solute to solution H sol

4-16
Ionization gaseous atom to H ion
gaseous positive ion
Electron gain gaseous atom to H eg
Gaseous negative ion
Bond dissociation gaseous molecule to H dis
dissociated gaseous species
G. Bond Enthalpies
One consequence of the fact that bond formation in many molecules is highly localized
between neighboring atoms is that bond enthalpies are fairly insensitive to the other (non-neighbor)
atoms of the molecule. As a result of this, average bond enthalpies that have been determined for
bonds between various atoms may be used to estimate enthalpies of reactions. Average bond
enthalpies for bonds of H, C, N, and O atoms with other common elements are given in Table 4-1.
Table 4-1. Bond enthalpies of common elements (kJ/mol)
H! C! C= C/ N! N= N/ O! O=
H 436 413 391 463
C 413 348 615 812 292 615 891 351 728
N 391 292 615 891 161 418 946
O 463 351 728 139 485
F 563 441 270 185
Si 295 290 369
P 320
S 339 259 477
Cl 432 328 200 203
Br 366 276
I 299 240
Bond enthalpies, such as those given in the above table, may be determined from enthalpies
of formation. For example, to obtain the C!H bond enthalpy, One can use the following information
for the CH molecule: CH ! C + 4H
4 4(g) (g) (g)
o
H (CH
f
4(g)
) = !74.85 kJ/mol

orbitals
orbitals
4-17
o
H (C ) = 718.38 kJ/mol
f
(g)
o
H (H ) = 217.94 kJ/mol.
f
o
Then H = 718.38 + 4(217.94)+ 74.85 = 1665.0 kJ,
and therefore the average C!H bond energy is 1665.0/4 = 416.0 kJ/mol.
(g)
As an illustration of how bond enthalpies may be used to estimate enthalpies of formation,
consider the case of benzene;
6C + 3H ! C H .
(gr) 2(g) 6 6(g)
Using the tabulated C=C, C!C, and C!H bond enthalpies, the
formation of benzene from the gaseous atoms as follows:
o
H can be estimated for the
Form 6 C!H bonds = 6 x !413 = !2478 kJ (Note: When bonds
3 C!C bonds = 3 x !348 = !1044 kJ are formed, H is
3 C=C bonds = 3 x !615 = !1845 kJ negative).
Total bond formation = !5367 kJ.
Then, to obtain the enthalpy of formation, we must add the enthalpy changes for the additional two
steps;
o
o
6C 6 6C , H = 6 H (C ) = 6 x 718.38 = 4308 kJ/mol
(gr) (g) f (g)
o
o
3H 6 6H , H = 6 H (H ) = 6 x 217.94 = 1308 kJ/mol.
2(g) (g) f (g)
o
Therefore, fH (C6H 6(g) ) = !5367 + 4308 + 1308 = 249 kJ/mol. Actually, this turns out to be a rather
poor estimate (the experimental value is 83 kJ/mol) because the double bonds in benzene are best
2
described in terms of sp hybridized σ
and delocalized
π
that extend around the
whole molecule. The delocalization causes the bond enthalpy to be considerably larger than that
expected for single and double bonds. This reduction in the bond enthalpy is referred to as
resonance stabilization.
H. Temperature dependence of enthalpy
It is often necessary to know reaction enthalpies at temperatures other than 298 K.
Consider the cycle shown below involving the same reaction at two temperatures T and T .
1 2

4-18
Since H is a state function
H(T ) 2
aA + bB ! cC T 2
H 9 H 9 8 H
1 2 3
aA + bB ! cC T 1
H(T ) 1
H(T ) = H + H + H(T ) + H .
2 1 2 1 3
For a reaction at constant pressure, the
substance may be calculated from the relation
H associated with changing the temperature of each
T 2
H ' n C P
dT.
T 1
Therefore, T 1
T 1
T 2
H(T 2) = H(T 1) + a C P
(A)dT % b C P
(B)dT % c C P
(C)dT.
T 2
T 2
T 1
Over a restricted range of temperatures, the heat capacities may be treated as constants, in which case
the above formula reduces to
where
H(T ) = H(T ) + C (T ! T ),
2 1 P 2 1
C j C P (i)
P = cC P(C) ! aC P(A) ! bC P(B) = .
iνi
I. Work of adiabatic expansion
The work associated with the expansion of a gas is given by
V 2
w ' !
V 1
P ex
dV
,
and for the isothermal expansion of an ideal gas, we found
a). w = !P V
ex
when P = constant
ex

). w = !nRT ln (V /V )
2 1
4-19
when process is reversible.
Also, for an ideal gas, since U = 0 when T = 0,
q = !w.
Now we shall explore the properties of an adiabatic expansion. For an adiabatic expansion,
q = 0, and so dU = dw. Therefore,
w ' dU ,
which means that when a gas expands adiabatically, the work done on the surroundings is
accompanied by a decrease in the internal energy of the system. Hence, the temperature of an ideal
gas will decrease in an adiabatic expansion. Since U is a state function, we may obtain the work for
an adiabatic expansion of n moles of an ideal gas from
T 2
w ' U ' n C V
dT ' nC V
T,
T 1
where the last expression assumes C is constant over the temperature range. The above equation
V
applies to both reversible and irreversible processes.
Lets consider the important case of a reversible adiabatic expansion of an ideal gas. The
initial state of the system is specified by P , V , and T , while the final state of the system is specified
1 1 1
by P , V , and T .
2 2 2
so
Therefore,
and
a). Determine the relationship between V and T.
where c = C /R. V
Thus
dw = !PexdV = !(nRT/V)dV = nCVdT,
ln V 1
V 2
T 2
C V
dT
T
T 1
V 2
RdV
' ! .
V
V 1
C V
ln T 2
T 1
' Rln V 1
V 2
' C V
R ln T 2
T 1
' ln T 2
T 1
,
c
,

=
>
=
4-20
.
b). Determine the relationship between P and V.
P 1
V 1
T 1
' P 2 V 2
T 2
so
Now
P 1
V 1
' T 1
' V 1
2 c
P 2
V 2
T 2
V 1
1% 1 c
P 1
V1 ' P 2
V
1% 1 c
1 % 1 c ' 1 % R C V
' C V % R
2
.
C V
Let γ
C P/C V, then
' C P
C V
.
.
Note that γ
1 for all gases and γ
5/3 for a monatomic ideal gas.
' T 1
Exercise: Show that ,
P 2
where
P 1
T 2
c )
c’ = C /R. P
P 1
V 1
P 2
V 2
' T 1
T 2
P 1
P 2
T 2
T 1
c
' T 1
T 2
P 1
P 2
' T 1
T 2
1%c
' T 1
T 2
c )
.

4-21
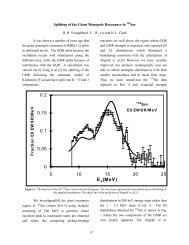
An adiabatic expansion is shown schematically on the PVT surface in Fig. 4-4, and an
adiabat (graph of P versus V for an adiabatic process) is compared with an isotherm (graph of P
versus V for an isothermal process) in Fig. 4-5. It should be noted in Fig. 4-4 that the pressure
decreases more in an adiabatic expansion than in an isothermal expansion between the same initial
and final volumes.
Figure 4.4. Depiction of an isothermal and an adiabatic expansion on the PVT surface (From
th
Physical Chemistry, 5 Edition, Peter Atkins, W. H. Freeman and Co., New York,
1994).

4-22
1.2
1.1
1.0
0.9
P (atm)
0.8
0.7
0.6
Isotherm
0.5
0.4
0.3
Adiabat
0.2
20 25 30 35 40 45 50
V (L)
Figure 4-5. An isotherm and an adiabat for the expansion of one mole of a monatomic ideal gas.

4-23
Review Questions
1. Define open, closed, and isolated thermodynamic systems.
2. What is a state function? List some state functions. List some non-state functions.
3. Define a reversible process.
4. Calculate the work involved in each of the following:
a).
b).
A balloon containing 1 mole of O at 298 K is suddenly ejected into outer space from the
2
space shuttle. (Neglect the elastic force of the balloon).
A bubble in a bottle of champagne expands when the bottle is uncorked. Assume the
bubble contains 0.3 mg of CO at 1.5 atm and 5 C, and that the outside pressure is 1 atm).
2
c). The expansion in part b is carried out reversibly.
5. Draw a PV diagram for parts (b) and (c) of problem 4.
6. A sufficient amount of heat is transferred to 25.0 g of carbon tetrachloride at its boiling
temperature (76.1 C) to cause it to evaporate inside a container with a frictionless piston. The
external pressure is 1 atm.
a).
b).
c).
d).
Does all the heat get changed into internal energy?
If your answer to (a) was no, explain where the rest goes.
The heat of vaporization of CCl is 30.00 kJ/mol. Calculate the internal energy change for
4
this process. (Neglect the volume of the liquid).
After the CCl has evaporated, the outside pressure is reduced to 0.5 atm while the
4
temperature is kept constant. Calculate the change in the internal energy and the change in
enthalpy associated with the resulting expansion.
7. Derive the relationship between C P and C V for an ideal gas.
8. Estimate the high temperature value of C for water vapor.
V
9. How much heat must be transferred to 3 mole of Ar to raise its temperature from 25 C to 100 C
(a) at constant volume, and (b) at constant pressure?
10. The standard heat of combustion of 1-hexene is -4034 kJ/mol. Calculate its standard heat of
formation.
11. Give a physical explanation of why C P is larger than C V.

4-24
12. Write reactions to define each of the following enthalpies:
(a).
sublimation
(b). solution
(c).
hydration
(d). ionization
(e).
lattice
13. Use tabulated bond enthalpies to estimate the enthalpy for the reaction
C H + H ! C H .
2 4(g) 2(g) 2 6(g)
14. Calculate H for the freezing of 2.000 mol of supercooled liquid water at -15.00 C at a pressure
of 1.00 atm, given that the standard enthalpy of fusion (T = 0 C) is 6.008 kJ/mol. Assume the
heat capacities of liquid water (76.1 J/mol-K) and ice (37.15 J/mol-K) are constant.
15. A 28.0 g sample of Kr at 25 atm and 300 K expands reversibly and adiabatically to a final
volume of 10.0 L. Calculate the following assuming Kr is an ideal gas:
(a).
(b). U
(c).
The final temperature
q
(d). w
(e). H .
16. A 28.0 g sample of Kr at 25 atm and 300 K expands reversibly and adiabatically to a final
pressure of 2.00 atm. Calculate the following assuming Kr is an ideal gas:
(a).
(b). U
(c).
The final temperature
q
(d). w
(e). H .

Answers:
4-25
4. (a) w = 0, (b) w = -0.0053 J, (c) w = -0.0064 J
rev
6. (c) U = 4.40 kJ, (d) H = 0.24 kJ
8. 6R
9. (a) q =2.806 kJ, (b) q = 4.677 kJ
o
10. fH (C6H 12) = -42 kJ/mol
o
13. H = !123 kJ/mol
o
14. fusH (258) = !10.848 kJ
15. a) T = 30.8 K, (b) U = -1121 J, (c) q = 0, (d) w = -1121 J, (e) H = -1869 J
2
16. a) T = 109 K , (b) U = -794.6 J, (c) q = 0, (d) w = -794.6 J, (e) H = -1325 J
2

4-26
FORMULAS YOU SHOULD KNOW
First law of thermodynamics:
U ' q % w
Differential work of expansion:
Work for isothermal reversible
expansion of an ideal gas:
Enthalpy:
Heat capacity
w ' !
C V
' dq V
dT '
C P
' dq P
dT '
dw ' !P ex
dV
V 2
V 1
P int
dV ' !nRT
H ' U % PV
constant volume: ;
Constant pressure: ;
V 2
MU U ' n
MT V
MH H ' n
MT P
dV
V ' !nRT ln V 2
V
V 1
1
T 2
T 1
C V
dT . nC V
T
T 2
T 1
C P
dT . nC P
T
Relationship between C V and CP
for an ideal gas:
C P
' C V
% R
Hess’s law:
H o ' jiνi f H o (i)
Reversible adiabatic expansion of an ideal gas:
w ' n
V 1
V 2
' T 2
T 1
T 2
T 1
C V
dT ' nC V
T
c
or
T 2
' V 1
1 c
T 1
V 2

=
4-27
where
where
c = C /R. V
P 1
P 2
' T 1
T 2
c )
c’ = C /R
,
P
,
P 1
Vγ1 ' P 2Vγ2 ' constant
where γ
C P/C V.