H-Matrix approximation for the operator exponential with applications

86 I. P. Gavrilyuk et al.
η
Γ 0
Γ δ
ξ
δ 1
γ 0
γ 1
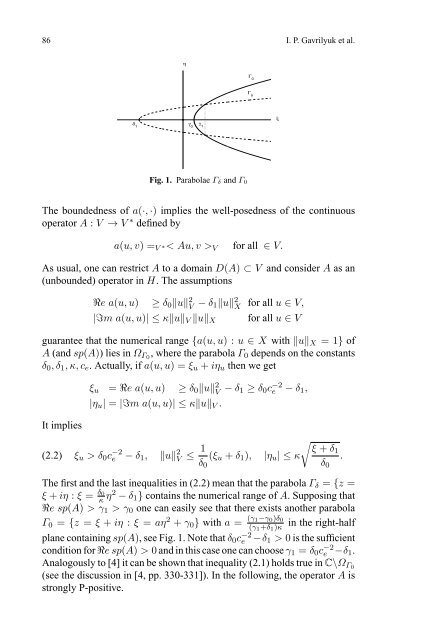
Fig. 1. Parabolae Γ δ and Γ 0
The boundedness of a(·, ·) implies the well-posedness of the continuous
operator A : V → V ∗ defined by
a(u, v) = V ∗< Au, v > V for all ∈ V.
As usual, one can restrict A to a domain D(A) ⊂ V and consider A as an
(unbounded) operator in H. The assumptions
Re a(u, u) ≥ δ 0 ‖u‖ 2 V − δ 1‖u‖ 2 X
|Im a(u, u)| ≤κ‖u‖ V ‖u‖ X
for all u ∈ V,
for all u ∈ V
guarantee that the numerical range {a(u, u) :u ∈ X with ‖u‖ X =1} of
A (and sp(A)) lies in Ω Γ0 , where the parabola Γ 0 depends on the constants
δ 0 ,δ 1 ,κ,c e . Actually, if a(u, u) =ξ u + iη u then we get
ξ u = Re a(u, u) ≥ δ 0 ‖u‖ 2 V − δ 1 ≥ δ 0 c −2
e − δ 1 ,
|η u | = |Im a(u, u)| ≤κ‖u‖ V .
It implies
(2.2) ξ u >δ 0 c −2
e − δ 1 , ‖u‖ 2 V ≤ 1 √
ξ + δ1
(ξ u + δ 1 ), |η u |≤κ .
δ 0 δ 0
The first and the last inequalities in (2.2) mean that the parabola Γ δ = {z =
ξ + iη : ξ = δ 0
κ
η 2 − δ 1 } contains the numerical range of A. Supposing that
Re sp(A) >γ 1 >γ 0 one can easily see that there exists another parabola
Γ 0 = {z = ξ + iη : ξ = aη 2 + γ 0 } with a = (γ 1−γ 0 )δ 0
(γ 1 +δ 1 )κ
in the right-half
plane containing sp(A), see Fig. 1. Note that δ 0 c −2
e −δ 1 > 0 is the sufficient
condition for Resp(A) > 0 and in this case one can choose γ 1 = δ 0 c −2
e −δ 1 .
Analogously to [4] it can be shown that inequality (2.1) holds true in C\Ω Γ0
(see the discussion in [4, pp. 330-331]). In the following, the operator A is
strongly P-positive.