New metrics for rigid body motion interpolation - helix

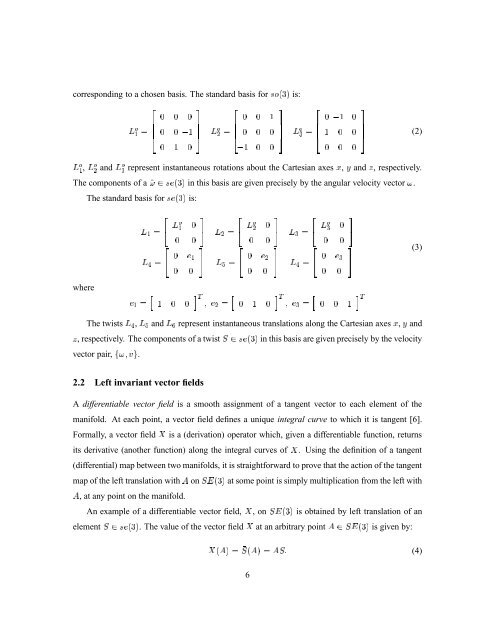
corresponding to a chosen basis. The standard basis for so(3) is:
2 3
3
0 0 0
0 0 1
6
7
6
7
L o 1 = 0 0 ,1
0 0 0
6
4
2
7
5 Lo 2 = 6
4
0 1 0
,1 0 0
2
7
5 Lo 3 = 6
4
0 ,1 0
1 0 0
0 0 0
3
7
5
(2)
L o, 1 Lo and 2 Lo 3
represent instantaneous rotations about the Cartesian axes x, y and z, respectively.
The components of a ^! 2 se(3) in this basis are given precisely by the angular velocity vector !.
The standard basis for se(3) is:
2
3
2
L 1 = 4 Lo 1
0
5 L2 = 4 Lo 2
0
5 L3 = 4 Lo 3
0
0 0
0 0
0 0
L 4 =
2
4 0 e 1
0 0
3
5 L 5 =
2
4 0 e 2
0 0
3
3
5 L 4 =
2
2
4 0 e 3
0 0
3
5
3
5
(3)
where
e 1 =
h
1 0 0
i T
; e2 =
h
0 1 0
i T
; e3 =
h
0 0 1
i T
The twists L 4 , L 5 and L 6 represent instantaneous translations along the Cartesian axes x, y and
z, respectively. The components of a twist S 2 se(3) in this basis are given precisely by the velocity
vector pair, f!; vg.
2.2 Left invariant vector fields
A differentiable vector field is a smooth assignment of a tangent vector to each element of the
manifold. At each point, a vector field defines a unique integral curve to which it is tangent [6].
Formally, a vector field X is a (derivation) operator which, given a differentiable function, returns
its derivative (another function) along the integral curves of X. Using the definition of a tangent
(differential) map between two manifolds, it is straightforward to prove that the action of the tangent
map of the left translation with A on SE(3) at some point is simply multiplication from the left with
A, at any point on the manifold.
An example of a differentiable vector field, X, onSE(3) is obtained by left translation of an
element S 2 se(3). The value of the vector field X at an arbitrary point A 2 SE(3) is given by:
X(A) = S(A) =AS: (4)
6