Sirgue, Laurent, 2003. Inversion de la forme d'onde dans le ...

More documents

Recommendations

Info

$Fayek & Kyser 2000 uraninite fractionations.pdf - Queen's University$

16 CHAPTER 2. INVERSE THEORY Unknowns/Equations G −1 exists if G has Solution n m < n d linearly independent columns At most one n m = n d linearly independent columns Only one n m > n d n d linearly independent columns More than one Table 2.1: Condition of existence of G −1 in equation 2.5 (p. 48 of Scales et al. (2001)). 2.2.1 Singular value decomposition The Singular Value Decomposition (SVD) method was introduced by Lanczos (1961). I briefly review in this section the principle results of the SVD. Further details on the method may be found in Aki and Richards (1980); Menke (1989); Scales et al. (2001). The singular value decomposition of any matrix G of dimension (n d × n m ) is expressed as G = UΛV t (2.7) where the columns of U (dimension (n d × n d )) are the data space eigenvectors of GG t , and the column of V (dimension (n m × n m )) are the model space eigenvectors of G t G. The matrix Λ of dimension (n d × n m ) is diagonal where the diagonal elements are called singular values. There are min(n d , n m ) number of singular values which are defined as the square roots of the first min(n d , n m ) eigenvalues of G t Gv i = λ 2 i v i i = 1, n m GG t u i = λ 2 i u i i = 1, n d (2.8) Each data eigenvector u i and model eigenvector v i are columns of U and V respectively such as U and V form a basis of orthonormal vectors. Any vector of the data or model space can thus be expressed as a linear combination of eigenvectors and we have U t U = UU t = I nd (2.9) V t V = VV t = I nm (2.10) Note that the eigenvalues λ 2 i defined in equation (2.8) may be positive or zero real numbers so that the diagonal terms of Λ may contain zeros. If n m ≠ n d , the number of eigenvectors of the data and model space are different. If p ≤ min(n d , n m ) is the number of non-zero singular values, the data and model space have p eigenvectors with associated non-zero eigenvalues.
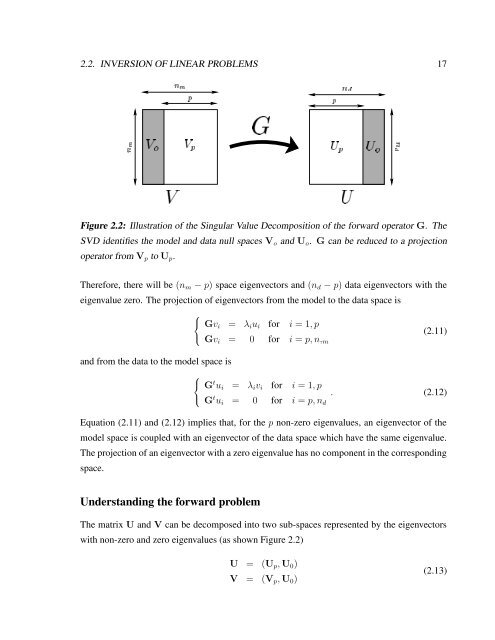
£ 2.2. INVERSION OF LINEAR PROBLEMS 17 ¤¦¥ §©¨ ¡ ¢ Figure 2.2: Illustration of the Singular Value Decomposition of the forward operator G. The SVD identifies the model and data null spaces V o and U o . G can be reduced to a projection operator from V p to U p . Therefore, there will be (n m − p) space eigenvectors and (n d − p) data eigenvectors with the eigenvalue zero. The projection of eigenvectors from the model to the data space is ⎧ ⎨ and from the data to the model space is ⎩ ⎧ ⎨ ⎩ Gv i = λ i u i for i = 1, p Gv i = 0 for i = p, n, m (2.11) G t u i = λ i v i for i = 1, p G t u i = 0 for i = p, n d . (2.12) Equation (2.11) and (2.12) implies that, for the p non-zero eigenvalues, an eigenvector of the model space is coupled with an eigenvector of the data space which have the same eigenvalue. The projection of an eigenvector with a zero eigenvalue has no component in the corresponding space. Understanding the forward problem The matrix U and V can be decomposed into two sub-spaces represented by the eigenvectors with non-zero and zero eigenvalues (as shown Figure 2.2) U = (U p , U 0 ) V = (V p , U 0 ) (2.13)
Page 1 and 2: Thèse de Doctorat de l’Universit
Page 3 and 4: Abstract The standard imaging appro
Page 5 and 6: Résumé de thèse L’approche sta
Page 7 and 8: vii l’inversion de la forme d’o
Page 9: Ackowledgments First and foremost,
Page 12 and 13: xii CONTENTS 3 A strategy for selec
Page 14 and 15: xiv CONTENTS 6 Conclusions 155 6.1
Page 16 and 17: xvi LIST OF FIGURES 3.11 Imapact of
Page 18 and 19: xviii LIST OF FIGURES
Page 20 and 21: xx LIST OF TABLES
Page 22 and 23: 2 CHAPTER 1. INTRODUCTION is made t
Page 24 and 25: 4 CHAPTER 1. INTRODUCTION the data
Page 26 and 27: 6 CHAPTER 1. INTRODUCTION quantify
Page 28 and 29: 8 CHAPTER 1. INTRODUCTION technique
Page 30 and 31: 10 CHAPTER 1. INTRODUCTION 1.8 Summ
Page 32 and 33: 12 CHAPTER 1. INTRODUCTION
Page 34 and 35: 14 CHAPTER 2. INVERSE THEORY Data M
Page 38 and 39: 18 CHAPTER 2. INVERSE THEORY where
Page 40 and 41: 20 CHAPTER 2. INVERSE THEORY Where
Page 42 and 43: 22 CHAPTER 2. INVERSE THEORY The mi
Page 44 and 45: ‰ 24 CHAPTER 2. INVERSE THEORY St
Page 46 and 47: œS œ *ž œ7Ÿ• ›&œ žu¡[¢
Page 48 and 49: 28 CHAPTER 2. INVERSE THEORY Adding
Page 50 and 51: 30 CHAPTER 2. INVERSE THEORY a) b)
Page 52 and 53: 32 CHAPTER 2. INVERSE THEORY Misfit
Page 54 and 55: 34 CHAPTER 2. INVERSE THEORY Gauss-
Page 56 and 57: O P 36 CHAPTER 2. INVERSE THEORY St
Page 58 and 59: 38 CHAPTER 2. INVERSE THEORY a) YZ
Page 60 and 61: 40 CHAPTER 2. INVERSE THEORY a) b)
Page 62 and 63: € 42 CHAPTER 2. INVERSE THEORY 10
Page 64 and 65: 44 CHAPTER 2. INVERSE THEORY The so
Page 66 and 67: 46 CHAPTER 2. INVERSE THEORY For a
Page 68 and 69: 48 CHAPTER 2. INVERSE THEORY The gr
Page 70 and 71: 50 CHAPTER 2. INVERSE THEORY using
Page 72 and 73: 52 CHAPTER 2. INVERSE THEORY
Page 74 and 75: 54 CHAPTER 3. STRATEGY FOR SELECTIN
Page 78 and 79: ë ¸ · 0 0.1 0.2 0.3 0.4 0.5 0.6
Page 84 and 85: „ 64 CHAPTER 3. STRATEGY FOR SELE
Page 86 and 87:
66 CHAPTER 3. STRATEGY FOR SELECTIN
Page 88 and 89:
Page 90 and 91:
Page 92 and 93:
a) Depth (Km) Depth (Km) 0 0.5 1.0
Page 94 and 95:
Page 96 and 97:
Page 98 and 99:
Page 100 and 101:
Page 102 and 103:
Page 104 and 105:
Page 106 and 107:
Page 108 and 109:
Page 110 and 111:
Page 112 and 113:
92 CHAPTER 4. STARTING FROM REALIST
Page 114 and 115:
Page 116 and 117:
Page 118 and 119:
a) b) Depth (km) 0 0.5 1.0 1.5 2.0
Page 120 and 121:
100 CHAPTER 4. STARTING FROM REALIS
Page 122 and 123:
a) b) Depth (km) 0 0.5 1.0 1.5 2.0
Page 124 and 125:
Page 126 and 127:
a) b) Depth (km) 0 0.5 1.0 1.5 2.0
Page 128 and 129:
Page 130 and 131:
Page 132 and 133:
Page 134 and 135:
Page 136 and 137:
Page 138 and 139:
Page 140 and 141:
Page 142 and 143:
Page 144 and 145:
Page 146 and 147:
Page 148 and 149:
Page 150 and 151:
Page 152 and 153:
Page 154 and 155:
Page 156 and 157:
136 CHAPTER 5. WAVEFORM INVERSION A
Page 158 and 159:
Page 160 and 161:
Page 162 and 163:
Page 164 and 165:
Page 166 and 167:
Page 168 and 169:
Page 170 and 171:
Page 172 and 173:
Page 174 and 175:
Page 176 and 177:
156 CHAPTER 6. CONCLUSIONS The wave
Page 178 and 179:
158 CHAPTER 6. CONCLUSIONS 6.3 Wave
Page 180 and 181:
160 CHAPTER 6. CONCLUSIONS
Page 182 and 183:
162 APPENDIX A. IMAGE STRETCH AND N
Page 184 and 185:
164 BIBLIOGRAPHY Bleistein, N., 198
Page 186 and 187:
166 BIBLIOGRAPHY Forgues, E., Scala
Page 188 and 189:
168 BIBLIOGRAPHY Lafond, C., Kaculi
Page 190 and 191:
170 BIBLIOGRAPHY Pratt, R. G., and
Page 192 and 193:
172 BIBLIOGRAPHY Tarantola, A., 198
show all

Sirgue, Laurent, 2003. Inversion de la forme d'onde dans le ...

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?