Linear Differential Game With Two Pursuers and One Evader

The 2x1 game only makes a change in the evader optimal command. The presence of a second pursuer
doesn’t change the pursuers behaviour. Indeed, the optimal controls for each pursuer in the 2x1 game
are the same as the ones in their respective 1x1 game.
When considering two pursuers and one evader (in the framework of DGL representations), some
cases are easy to solve. If E is “above” (or symmetrically “below”) the two pursuers (in ZEM, Tgo
frame), the optimal evasion respect to each pursuer (considered alone) and according to both pursuers
together is to turn right (to turn left when below) with maximum acceleration. In these cases (E
“above” or “below”), there are no changes in evasion trajectory (optimal strategies) by adding a
pursuer.
ZEM
P1
E
P1E Reference
P2
P2E Reference
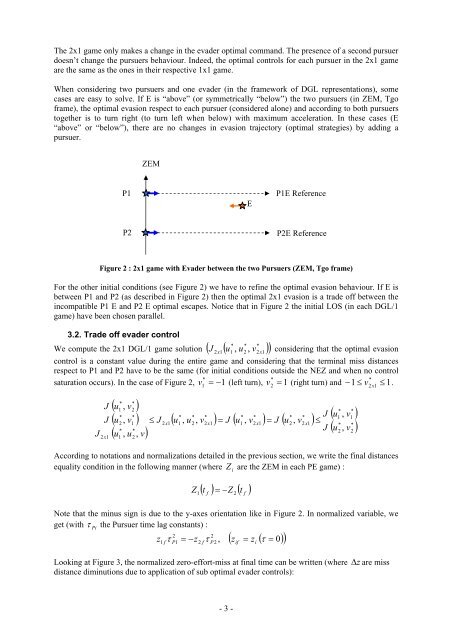
Figure 2 : 2x1 game with Evader between the two Pursuers (ZEM, Tgo frame)
For the other initial conditions (see Figure 2) we have to refine the optimal evasion behaviour. If E is
between P1 and P2 (as described in Figure 2) then the optimal 2x1 evasion is a trade off between the
incompatible P1 E and P2 E optimal escapes. Notice that in Figure 2 the initial LOS (in each DGL/1
game) have been chosen parallel.
3.2. Trade off evader control
* * *
We compute the 2x1 DGL/1 game solution ( ( u u v
)
J
2 x1
1
,
2
,
2x1
considering that the optimal evasion
control is a constant value during the entire game and considering that the terminal miss distances
respect to P1 and P2 have to be the same (for initial conditions outside the NEZ and when no control
*
*
*
saturation occurs). In the case of Figure 2, v = 1 (left turn), v 1 (right turn) and −1
≤ v 1.
J
J
J
2x1
* *
( u1
, v2
)
* *
( u2
, v1
)
* *
( u , u , v)
1
2
≤ J
2x1
1
−
2
=
* * *
* *
* *
( u , u , v ) = J ( u , v ) = J ( u , v )
1
2
2x1
1
2x1
2
2x1
J
≤
J
* *
( u1
, v1
)
* *
( u , v )
2
2
2x1
≤
According to notations and normalizations detailed in the previous section, we write the final distances
equality condition in the following manner (where Z are the ZEM in each PE game) :
( t ) = Z ( t )
Z1 f
−
2
Note that the minus sign is due to the y-axes orientation like in Figure 2. In normalized variable, we
get (with τ
Pi
the Pursuer time lag constants) :
2
2
z τ = −z
τ , z = z τ 0
i
f
( ( ))
1 f P1
2 f P2
if i
=
Looking at Figure 3, the normalized zero-effort-miss at final time can be written (where
distance diminutions due to application of sub optimal evader controls):
Δz
are miss
- 3 -