Equalization of Audio Systems using Kautz Filters ... - TKK Acoustics

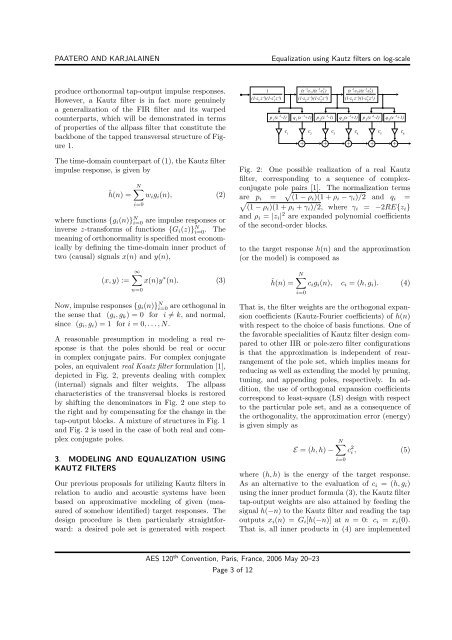
PAATERO AND KARJALAINENEqualization using Kautz filters on log-scaleproduce orthonormal tap-output impulse responses.However, a Kautz filter is in fact more genuinelya generalization of the FIR filter and its warpedcounterparts, which will be demonstrated in termsof properties of the allpass filter that constitute thebackbone of the tapped transversal structure of Figure1.The time-domain counterpart of (1), the Kautz filterimpulse response, is given byĥ(n) =N∑w i g i (n), (2)i=0where functions {g i (n)} N i=0 are impulse responses orinverse z-transforms of functions {G i (z)} N i=0 . Themeaning of orthonormality is specified most economicallyby defining the time-domain inner product oftwo (causal) signals x(n) and y(n),(x, y) :=∞∑x(n)y ∗ (n). (3)n=0Now, impulse responses {g i (n)} N i=0 are orthogonal inthe sense that (g i , g k ) = 0 for i ≠ k, and normal,since (g i , g i ) = 1 for i = 0, . . . , N.A reasonable presumption in modeling a real responseis that the poles should be real or occurin complex conjugate pairs. For complex conjugatepoles, an equivalent real Kautz filter formulation [1],depicted in Fig. 2, prevents dealing with complex(internal) signals and filter weights. The allpasscharacteristics of the transversal blocks is restoredby shifting the denominators in Fig. 2 one step tothe right and by compensating for the change in thetap-output blocks. A mixture of structures in Fig. 1and Fig. 2 is used in the case of both real and complexconjugate poles.3. MODELING AND EQUALIZATION USINGKAUTZ FILTERSOur previous proposals for utilizing Kautz filters inrelation to audio and acoustic systems have beenbased on approximative modeling of given (measuredof somehow identified) target responses. Thedesign procedure is then particularly straightforward:a desired pole set is generated with respectFig. 2: One possible realization of a real Kautzfilter, corresponding to a sequence of complexconjugatepole pairs [1]. The normalization termsare p i = √ √(1 − ρ i )(1 + ρ i − γ i )/2 and q i =(1 − ρi )(1 + ρ i + γ i )/2, where γ i = −2RE{z i }and ρ i = |z i | 2 are expanded polynomial coefficientsof the second-order blocks.to the target response h(n) and the approximation(or the model) is composed asĥ(n) =N∑c i g i (n), c i = (h, g i ). (4)i=0That is, the filter weights are the orthogonal expansioncoefficients (Kautz-Fourier coefficients) of h(n)with respect to the choice of basis functions. One ofthe favorable specialities of Kautz filter design comparedto other IIR or pole-zero filter configurationsis that the approximation is independent of rearrangementof the pole set, which implies means forreducing as well as extending the model by pruning,tuning, and appending poles, respectively. In addition,the use of orthogonal expansion coefficientscorrespond to least-square (LS) design with respectto the particular pole set, and as a consequence ofthe orthogonality, the approximation error (energy)is given simply asE = (h, h) −N∑c 2 i , (5)i=0where (h, h) is the energy of the target response.As an alternative to the evaluation of c i = (h, g i )using the inner product formula (3), the Kautz filtertap-output weights are also attained by feeding thesignal h(−n) to the Kautz filter and reading the tapoutputs x i (n) = G i [h(−n)] at n = 0: c i = x i (0).That is, all inner products in (4) are implementedAES 120 th Convention, Paris, France, 2006 May 20–23Page 3 of 12