Applications of finite geometry in coding theory and cryptography

More documents

Recommendations

Info

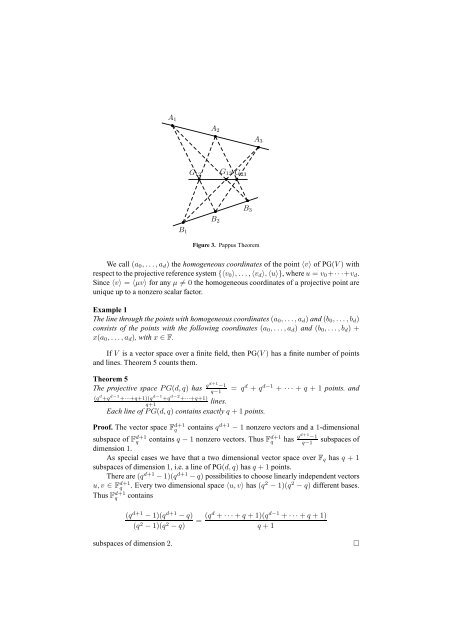
¦A 1A 2¡A 3¢G 12 G § 13 ¨G23¥¤ B 3£ B 2B 1Figure 3. Pappus TheoremWe call (a 0 , . . .,a d ) the homogeneous coordinates of the point 〈v〉 of PG(V ) withrespect to the projective reference system {〈v 0 〉, . . . , 〈v d 〉, 〈u〉}, where u = v 0 +· · ·+v d .Since 〈v〉 = 〈µv〉 for any µ ≠ 0 the homogeneous coordinates of a projective point areunique up to a nonzero scalar factor.Example 1The line through the points with homogeneous coordinates (a 0 , . . . , a d ) and (b 0 , . . . , b d )consists of the points with the following coordinates (a 0 , . . . , a d ) and (b 0 , . . . , b d ) +x(a 0 , . . . , a d ), with x ∈ F.If V is a vector space over a finite field, then PG(V ) has a finite number of pointsand lines. Theorem 5 counts them.Theorem 5The projective space PG(d, q) has qd+1 −1q−1(q d +q d−1 +···+q+1)(q d−1 +q d−2 +···+q+1)q+1lines.Each line of PG(d, q) contains exactly q + 1 points.Proof. The vector space F d+1q= q d + q d−1 + · · · + q + 1 points. andcontains q d+1 − 1 nonzero vectors and a 1-dimensionalsubspaces ofdimension 1.As special cases we have that a two dimensional vector space over F q has q + 1subspaces of dimension 1, i.e. a line of PG(d, q) has q + 1 points.There are (q d+1 − 1)(q d+1 − q) possibilities to choose linearly independent vectorssubspace of F d+1q contains q − 1 nonzero vectors. Thus F d+1qu, v ∈ F d+1qThus F d+1qhas qd+1 −1q−1. Every two dimensional space 〈u, v〉 has (q 2 − 1)(q 2 − q) different bases.contains(q d+1 − 1)(q d+1 − q)(q 2 − 1)(q 2 − q)= (qd + · · · + q + 1)(q d−1 + · · · + q + 1)q + 1subspaces of dimension 2.□
As indicated in the abstract, projective geometry is first of all investigated because ofits pure mathematical importance. But projective geometry is also important because ofits links to other research areas. We now present coding theory, one of the most importantresearch areas linked to projective geometry. For a detailed discussion of finite projectivespaces we refer to [12, 13, 15].2. Coding theory2.1. Introduction to coding theoryWhen sending a message there is always a small probability for transmission errors. Thegoal of coding theory is to develop good codes to detect and correct transmission errors.noisedata source encoder channel decoder receiverFigure 4. Transmission of data through a noisy communication channelSuppose for example that we transmit a binary message. With a probability p of 2%a transmission error occurs and one 1 is received as 0 and vice versa.Example 2 (Triple repetition code)We repeat every symbol three times, i.e. we send 000 instead of 0 and 111 instead of 1. Ifan error occurs we guess that the majority of the received symbols is correct, i.e. we willdecode 110 as 1.The probability that more than 1 error occurs in a triplet is 3p 2 (1 − p) + p 3 . Ifp = 0.02 we lowered the probability for incorrect decoding to 0.0012. The price is thatwe have to send 3 times more symbols.Example 3 (The Hamming code)Now we use the following encodingwithx 4 ≡ x 1 + x 2 + x 3 mod 2 ,x 5 ≡ x 0 + x 2 + x 3 mod 2 ,x 6 ≡ x 0 + x 1 + x 3 mod 2 .(x 0 , x 1 , x 2 , x 3 ) ↦→ (x 0 , . . .,x 6 )
Page 1 and 2: Applications of finite geometry in
Page 3: £A 1¡A 2¦Each line 〈v, w〉 of
Page 7 and 8: ⎛⎞1 0 g 1,k+1 . . . g 1,n⎜G =
Page 9 and 10: Iterating Equation (1) gives:N q (k
Page 11 and 12: θ k+1 − θ k−s+1θ s+ θ k−s
Page 13 and 14: Take two skew planes π and ¯π. L
Page 15 and 16: for every B ⊃ A.Example 8 shows h
Page 17 and 18: 67 67 1 115
Page 19: 3.3. AESIn 1997 the American Nation

Applications of finite geometry in coding theory and cryptography

Create successful ePaper yourself

Delete template?

Save as template?