3.1 Sistemas de Lienard

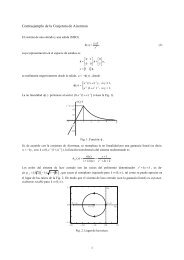
8El origen es un punto de equilibrio, y existen infinitos ciclos límites Γ n formados por los círculos de radior =1/(nπ). Estos ciclos límites se acumulan en el origen; cada uno de los ciclos Γ 2n es estable mientrasque los Γ 2n+1 son inestables. 2El teorema de Dulac asegura que H n (a, b) < ∞. El número de Hilbert H n se define comoH n =sup H n (a, b).(a,b)∈R (n+1)(n+2)Los sistemas lineales no tienen ciclos límites en R 2 de donde resulta que H 1 =0. En cambio, parael caso más simple de sistemas no lineales, los sistemas planares cuadráticos [(3.12) con n =2],no se ha podido determinar el número de Hilbert H 2 . En 1952, el matemático ruso N. V. Buatinprobó que cualquier sistema planar cuadrático tiene a lo sumo 3 ciclos límites locales. Durantecierto tiempo se creyó que H 2 =3;sin embargo, en 1979 los matemáticos chinos S. L. Shi, L. S.ChenyM.S.Wangprodujeronejemplosdesistemas planares cuadráticos con 4 ciclos límites. Porlo tanto, H 2 ≥ 4. Basado en la evidencia disponible hasta el momento, se cree que H 2 =4. En1984 Y. X. Chin aseguró haber probado este resultado; sin embargo, una serie de errores fueronencontrados en este trabajo por Y. L. Cao.Respecto de H 3 , se sabe que un sistema planar cúbico puede tener al menos 11 ciclos límite(Zoladek, 1995). En 1986 J. B. Li y Q. Huang hallaron un sistema planar cúbico con once cicloslímite. Hasta este momento, todo lo que puede decirse es que H 3 ≥ 11. El Problema 16 de Hilbertpara sistemas planares ha despertado mucho interés en los últimos años, y probablemente continúehaciéndolo por algún tiempo más.3.2.1 ReferenciasN. Bautin, On the number of limit cycles which appear with a variation of coefficients from anequilibrium position of a focus or center type, Mat. Sb., 30, 1952, pp. 181-196. A.M.S. TranslationNo. 100, 1954, pp. 3-19.T. Blows, N. Lloyd, The number of small amplitude limit cycles of Lienard equations, Math. Proc.Cambridge Phil. Soc., 95, 1984, pp. 751-758.H. Dulac, Sur les cycles limites, Bull.Soc.Math.France, 51, 1923, pp. 45-188.H. K. Khalil, Nonlinear Systems, 3ra ed., Prentice-Hall, New Jersey, 2002.J. Li, Q. Huang, Bifucation of limit cycles forming compound eyes in the cubic system. ChineseAnn. Math, 8B(4), 1987, pp. 391-403.A. Lins, W. de Melo, C. Pugh, On Lienard’s equation, Lecture Notes in Mathematics, 597,Springer-Verlag, 1977, pp. 335-357.L. Perko, Differential Equations and Dynamical Systems, 2da. Ed., Texts in Applied Mathematics7, Springer-Verlag, New York, 1996.Shi Songling, A concrete example of the existence of four limit cycles for plane quadratic systems,Sci. Sinica, 23, 1980, pp. 153-158.Zhang Ziefen, On the uniqueness of limits cicles of certain equations of nonlinear oscillations, Dokl.Akad. Nauk. SSSR, 19, 1958, pp. 659-662.Zhang Ziefen, On the existence of exactly two limit cycles for the Lienard equation, Acta Math.Sinica, 24, 1981, pp. 710-716.H. Zoladek, Eleven small limit cycles in a cubic vector field, Nonlinearity, 8, 1995, pp. 843-860.