RANDOM FIELDS AND THEIR GEOMETRY

8 1. Random fields
When N = 1, W is the standard Brownian motion on [0, ∞). When
N > 1, if we fix N − k of the indices, it is a scaled k-parameter Brownian
sheet in the remaining variables. (This is easily checked via the covariance
function.) Also, when N > 1, it follows immediately from (1.3.6) that
Wt = 0 when mink tk = 0; i.e. when t is on one of the axes. It is this image,
with N = 2, of a sheet tucked in at two sides and given a good shake, that
led Ron Pyke [76] to introduce the name.
A simple simulation of a Brownian sheet, along with its contour lines, is
in Figure 1.3.
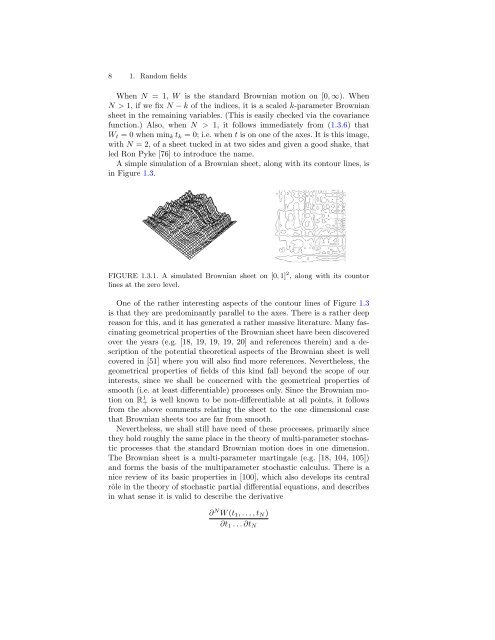
FIGURE 1.3.1. A simulated Brownian sheet on [0, 1] 2 , along with its countor
lines at the zero level.
One of the rather interesting aspects of the contour lines of Figure 1.3
is that they are predominantly parallel to the axes. There is a rather deep
reason for this, and it has generated a rather massive literature. Many fascinating
geometrical properties of the Brownian sheet have been discovered
over the years (e.g. [18, 19, 19, 19, 20] and references therein) and a description
of the potential theoretical aspects of the Brownian sheet is well
covered in [51] where you will also find more references. Nevertheless, the
geometrical properties of fields of this kind fall beyond the scope of our
interests, since we shall be concerned with the geometrical properties of
smooth (i.e. at least differentiable) processes only. Since the Brownian motion
on R 1 + is well known to be non-differentiable at all points, it follows
from the above comments relating the sheet to the one dimensional case
that Brownian sheets too are far from smooth.
Nevertheless, we shall still have need of these processes, primarily since
they hold roughly the same place in the theory of multi-parameter stochastic
processes that the standard Brownian motion does in one dimension.
The Brownian sheet is a multi-parameter martingale (e.g. [18, 104, 105])
and forms the basis of the multiparameter stochastic calculus. There is a
nice review of its basic properties in [100], which also develops its central
rôle in the theory of stochastic partial differential equations, and describes
in what sense it is valid to describe the derivative
∂ N W (t1, . . . , tN)
∂t1 . . . ∂tN