RANDOM FIELDS AND THEIR GEOMETRY

30 1. Random fields
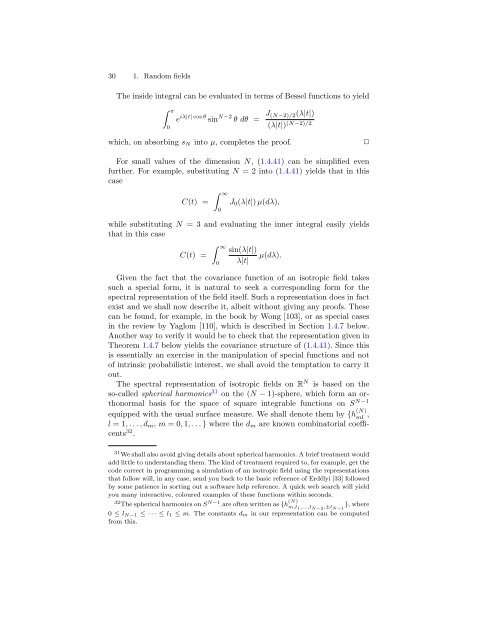
The inside integral can be evaluated in terms of Bessel functions to yield
� π
0
e iλ|t| cos θ sin N−2 θ dθ = J (N−2)/2(λ|t|)
(λ|t|) (N−2)/2
which, on absorbing sN into µ, completes the proof. ✷
For small values of the dimension N, (1.4.41) can be simplified even
further. For example, substituting N = 2 into (1.4.41) yields that in this
case
C(t) =
� ∞
0
J0(λ|t|) µ(dλ),
while substituting N = 3 and evaluating the inner integral easily yields
that in this case
C(t) =
� ∞
0
sin(λ|t|)
λ|t|
µ(dλ).
Given the fact that the covariance function of an isotropic field takes
such a special form, it is natural to seek a corresponding form for the
spectral representation of the field itself. Such a representation does in fact
exist and we shall now describe it, albeit without giving any proofs. These
can be found, for example, in the book by Wong [103], or as special cases
in the review by Yaglom [110], which is described in Section 1.4.7 below.
Another way to verify it would be to check that the representation given in
Theorem 1.4.7 below yields the covariance structure of (1.4.41). Since this
is essentially an exercise in the manipulation of special functions and not
of intrinsic probabilistic interest, we shall avoid the temptation to carry it
out.
The spectral representation of isotropic fields on R N is based on the
so-called spherical harmonics 31 on the (N − 1)-sphere, which form an orthonormal
basis for the space of square integrable functions on S N−1
equipped with the usual surface measure. We shall denote them by {h (N)
ml ,
l = 1, . . . , dm, m = 0, 1, . . . } where the dm are known combinatorial coefficents
32 .
31 We shall also avoid giving details about spherical harmonics. A brief treatment would
add little to understanding them. The kind of treatment required to, for example, get the
code correct in programming a simulation of an isotropic field using the representations
that follow will, in any case, send you back to the basic reference of Erdélyi [33] followed
by some patience in sorting out a software help reference. A quick web search will yield
you many interactive, coloured examples of these functions within seconds.
32 The spherical harmonics on S N−1 are often written as {h (N)
m,l1,...,l N−2,±l N−1 }, where
0 ≤ lN−1 ≤ · · · ≤ l1 ≤ m. The constants dm in our representation can be computed
from this.