View PDF - Project Euclid

CURVATURE AND COMPLEX SINGULARITIES 449
(2.5) | KI ds >- 47r
!
(Fary-Milnor).
Proof.
We give C parametrically according to arc length by x(s), and consider
the Gauss map
y C-- S
defined by y(s) x’ (s). Now, and this is the main geometric point, for any great
circle H, n(H f3 y(C)) is just the number of times that the tangent line to C lies
in a 2-plane parallel to H. Equivalently, if we choose coordinates (Xl, x2, x3) so
that/4 is the (xa, x)-plane, then n(I-I N y(C)) is just the number of critical values
of the height function x3(s) on C. This is always an even number _> 2, and if
n(/4 C) y(C)) is equal to 2 on an open set of great circles then C is unknotted.
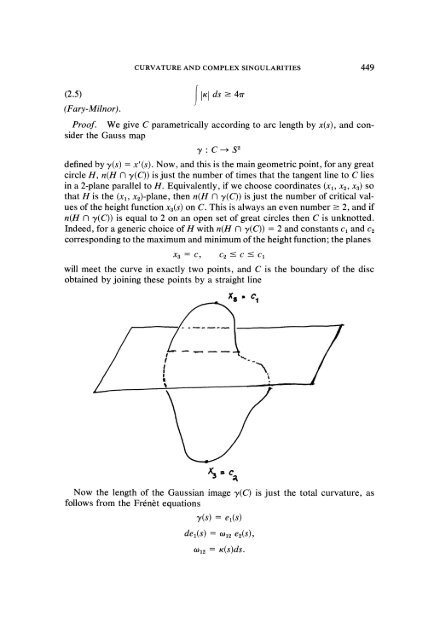
Indeed, for a generic choice of/4 with n(/4 y(C)) 2 and constants ca and cz
corresponding to the maximum and minimum of the height function; the planes
2 3 C C2 -< C--< C
will meet the curve in exactly two points, and C is the boundary of the disc
obtained by joining these points by a straight line
Now the length of the Gaussian image y(C) is just the total curvature, as
follows from the Fr6n6t equations
y(s) ea(s)
dea(s) ooa ez(s),
o (s)ds.