View PDF - Project Euclid

466 PHILLIP A. GRIFFITHS
exists and is called the Lelong number of V at the origin. We shall now prove
that
(3.17) /x(V) C te mult0 (V)
is a (constant times) the multiplicity of V at the origin. (r For this we let
E c IpN-1
be the closure of the incidence correspondence
{(L,z)’L IPu-a,z u-- {0},Z L}.
Then E --0 {U is the blow-up of Gu at the origin, and E N- is the total
space of the universal Hopf line bundle. The closure of V {0} in E gives the
proper transform of V; since the fibre 9 & N- of g over the origin is
the limiting position of chords 0z (z V), the intersection
T= 9 N-1
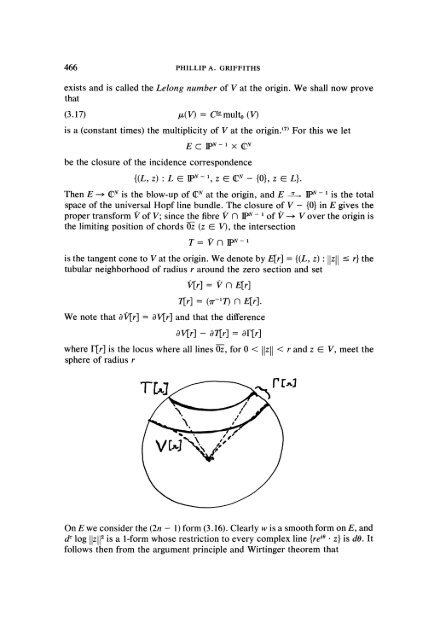
is the tangent cone to V at the origin. We denote by r] {(L, z) ilzll r} the
tubular neighborhood of radius r around the zero section and set
r] 9 r]
r] (- r].
We note that Or] Or] and that the difference
0r] 0r] 0r[r]
where Fir] is the locus where all lines 0z, for 0 < ilzll
sphere of radius r
T
< r and z V, meet the
On E we consider the (2n 1) form (3.16). Clearly w is a smooth form on E, and
d log Ilzll is a 1-form whose restriction to every complex line {re ’. z} is dO. It
follows then from the argument principle and Wirtinger theorem that