View PDF - Project Euclid

CURVATURE AND COMPLEX SINGULARITIES 497
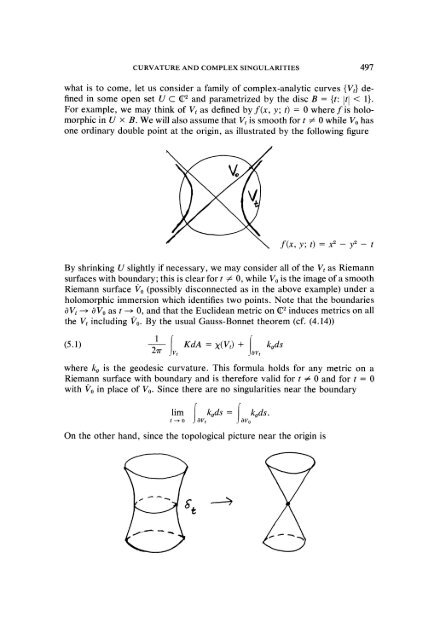
what is to come, let us consider a family of complex-analytic curves {Vt} defined
in some open set U C 2 and parametrized by the disc B {t" It < 1}.
For example, we may think of Vt as defined by f(x, y; t) 0 where f is holomorphic
in U B. We will also assume that V is smooth for t 0 while V0 has
one ordinary double point at the origin, as illustrated by the following figure
f(x, y; t) x yz
By shrinking U slightly if necessary, we may consider all of the Vt as Riemann
surfaces with boundary; this is clear for 0, while V0 is the image of a smooth
Riemann surface 0 (possibly disconnected as in the above example) under a
holomorphic immersion which identifies two points. Note that the boundaries
OVt 0 Vo as 0, and that the Euclidean metric on induces metrics on all
the Vt including 9o. By the usual Gauss-Bonnet theorem (cf. (4.14))
(5.1)
Iv KdA=x(Vt)+ Io 2r kods
v
where ko is the geodesic curvature. This formula holds for any metric on a
Riemann surface with boundary and is therefore valid for 0 and for 0
with 0 in place of V0. Since there are no singularities near the boundary
lim f kods= f kods.
OVt OVo
On the other hand, since the topological picture near the origin is