Waveguides - ieeetsu

www.ece.rutgers.edu/∼orfanidi/ewa 273
274 Electromagnetic Waves & Antennas – S. J. Orfanidis – June 21, 2004
Although these expressions look different, they are actually equal, W e ′ = W m ′ . Indeed,
using the property β 2 /k 2 c + 1 = (β2 + k 2 c )/k2 c = k2 /k 2 c = ω2 /ω 2 c and the relationships
between the constants in (8.7.1), we find:
µ ( |H 1 | 2 +|H 0 | 2) = µ ( |H 0 | 2 β2
k 2 +|H 0 | 2) = µ|H 0 | 2 ω2
c
ωc
2 = µ η |E 0| 2 = ɛ|E 2 0 | 2
The equality of the electric and magnetic energies is a general property of waveguiding
systems. We also encountered it in Sec. 2.3 for uniform plane waves. The total
energy density per unit length will be:
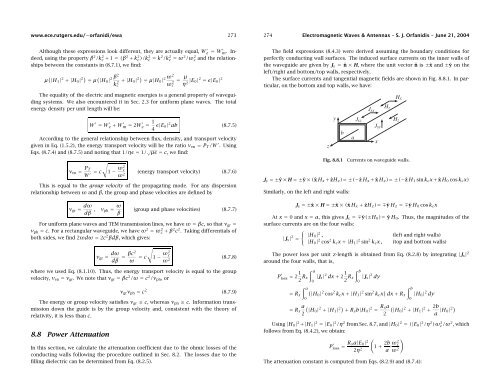
The field expressions (8.4.3) were derived assuming the boundary conditions for
perfectly conducting wall surfaces. The induced surface currents on the inner walls of
the waveguide are given by J s = ˆn × H, where the unit vector ˆn is ±ˆx and ±ŷ on the
left/right and bottom/top walls, respectively.
The surface currents and tangential magnetic fields are shown in Fig. 8.8.1. In particular,
on the bottom and top walls, we have:
W ′ = W ′ e + W′ m = 2W′ e = 1 4 ɛ|E 0| 2 ab (8.7.5)
According to the general relationship between flux, density, and transport velocity
given in Eq. (1.5.2), the energy transport velocity will be the ratio v en = P T /W ′ . Using
Eqs. (8.7.4) and (8.7.5) and noting that 1/ηɛ = 1/ √ µɛ = c, we find:
√
v en = P T
W ′ = c 1 − ω2 c
(energy transport velocity) (8.7.6)
ω 2
This is equal to the group velocity of the propagating mode. For any dispersion
relationship between ω and β, the group and phase velocities are defined by
v gr = dω
dβ ,
v ph = ω β
(group and phase velocities) (8.7.7)
For uniform plane waves and TEM transmission lines, we have ω = βc, so that v gr =
v ph = c. For a rectangular waveguide, we have ω 2 = ω 2 c + β2 c 2 . Taking differentials of
both sides, we find 2ωdω = 2c 2 βdβ, which gives:
√
v gr = dω
dβ = βc2
ω
= c 1 − ω2 c
ω 2 (8.7.8)
where we used Eq. (8.1.10). Thus, the energy transport velocity is equal to the group
velocity, v en = v gr . We note that v gr = βc 2 /ω = c 2 /v ph ,or
Fig. 8.8.1
Currents on waveguide walls.
J s =±ŷ × H =±ŷ × (ˆx H x + ẑH z )=±(−ẑ H x + ˆx H z )=±(−ẑ H 1 sin k c x + ˆx H 0 cos k c x)
Similarly, on the left and right walls:
J s =±ˆx × H =±ˆx × (ˆx H x + ẑH z )=∓ŷ H z =∓ŷ H 0 cos k c x
At x = 0 and x = a, this gives J s =∓ŷ(±H 0 )= ŷ H 0 . Thus, the magnitudes of the
surface currents are on the four walls:
{
|J s | 2 |H0 | 2 , (left and right walls)
=
|H 0 | 2 cos 2 k c x +|H 1 | 2 sin 2 k c x, (top and bottom walls)
The power loss per unit z-length is obtained from Eq. (8.2.8) by integrating |J s | 2
around the four walls, that is,
P ′ loss = 2 1 2 R s
∫ a
0
|J s | 2 dx + 2 1 2 R s
∫ b
0
|J s | 2 dy
v gr v ph = c 2 (8.7.9)
The energy or group velocity satisfies v gr ≤ c, whereas v ph ≥ c. Information transmission
down the guide is by the group velocity and, consistent with the theory of
relativity, it is less than c.
8.8 Power Attenuation
In this section, we calculate the attenuation coefficient due to the ohmic losses of the
conducting walls following the procedure outlined in Sec. 8.2. The losses due to the
filling dielectric can be determined from Eq. (8.2.5).
∫ a
(
= R s |H0 | 2 cos 2 k c x +|H 1 | 2 sin 2 k c x ) ∫ b
dx + R s |H 0 | 2 dy
0
0
a (
= R s |H0 | 2 +|H 1 | 2) + R s b|H 0 | 2 = R sa (
|H0 | 2 +|H 1 | 2 + 2b 2
2
a |H 0| 2)
Using |H 0 | 2 +|H 1 | 2 =|E 0 | 2 /η 2 from Sec. 8.7, and |H 0 | 2 = (|E 0 | 2 /η 2 )ωc/ω 2 2 , which
follows from Eq. (8.4.2), we obtain:
P ′ loss = R sa|E 0 |
(1 2
+ 2b )
ω 2 c
2η 2 a ω 2
The attenuation constant is computed from Eqs. (8.2.9) and (8.7.4):