Waveguides - ieeetsu

www.ece.rutgers.edu/∼orfanidi/ewa 267
268 Electromagnetic Waves & Antennas – S. J. Orfanidis – June 21, 2004
Then, the corresponding electric field will be:
where we defined the constants:
E y (x)= −η TE H x (x)= −η TE
jβ
k c
H 0 sin k c x ≡ E 0 sin k c x
H 1 = jβ
k c
H 0
E 0 =−η TE H 1 =−η TE
jβ
k c
H 0 =−jη ω ω c
H 0
(8.4.2)
where we used η TE = ηω/βc. In summary, the non-zero field components are:
H z (x)= H 0 cos k c x
H x (x)= H 1 sin k c x
E y (x)= E 0 sin k c x
⇒
H z (x, y, z, t)= H 0 cos k c xe jωt−jβz
H x (x, y, z, t)= H 1 sin k c xe jωt−jβz
E y (x, y, z, t)= E 0 sin k c xe jωt−jβz (8.4.3)
Assuming perfectly conducting walls, the boundary conditions require that there be
no tangential electric field at any of the wall sides. Because the electric field is in the
y-direction, it is normal to the top and bottom sides. But, it is parallel to the left and
right sides. On the left side, x = 0, E y (x) vanishes because sin k c x does. On the right
side, x = a, the boundary condition requires:
E y (a)= E 0 sin k c a = 0 ⇒ sin k c a = 0
This requires that k c a be an integral multiple of π:
k c a = nπ ⇒ k c = nπ (8.4.4)
a
These are the so-called TE n0 modes. The corresponding cutoff frequency ω c = ck c ,
f c = ω c /2π, and wavelength λ c = 2π/k c = c/f c are:
ω c = cnπ
a , f c = cn
2a , λ c = 2a (TE n0 modes) (8.4.5)
n
The dominant mode is the one with the lowest cutoff frequency or the longest cutoff
wavelength, that is, the mode TE 10 having n = 1. It has:
k c = π a , ω c = cπ a , f c = c
2a , λ c = 2a (TE 10 mode) (8.4.6)
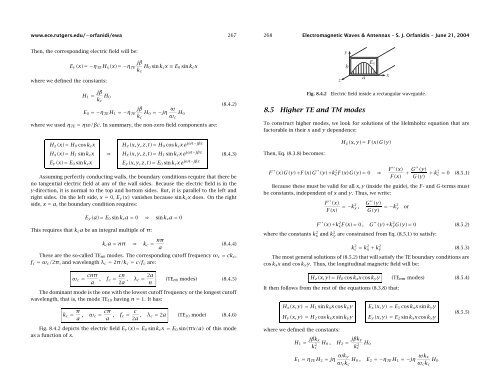
Fig. 8.4.2 depicts the electric field E y (x)= E 0 sin k c x = E 0 sin(πx/a) of this mode
as a function of x.
Fig. 8.4.2
8.5 Higher TE and TM modes
Electric field inside a rectangular waveguide.
To construct higher modes, we look for solutions of the Helmholtz equation that are
factorable in their x and y dependence:
Then, Eq. (8.3.8) becomes:
H z (x, y)= F(x)G(y)
F ′′ (x)G(y)+F(x)G ′′ (y)+k 2 cF(x)G(y)= 0 ⇒ F′′ (x)
F(x) + G′′ (y)
G(y) + k2 c = 0 (8.5.1)
Because these must be valid for all x, y (inside the guide), the F- and G-terms must
be constants, independent of x and y. Thus, we write:
F ′′ (x)
F(x) =−k2 x ,
G ′′ (y)
G(y) =−k2 y
F ′′ (x)+k 2 xF(x)= 0 , G ′′ (y)+k 2 yG(y)= 0 (8.5.2)
where the constants k 2 x and k2 y are constrained from Eq. (8.5.1) to satisfy:
k 2 c = k 2 x + k 2 y (8.5.3)
The most general solutions of (8.5.2) that will satisfy the TE boundary conditions are
cos k x x and cos k y y. Thus, the longitudinal magnetic field will be:
H z (x, y)= H 0 cos k x x cos k y y (TE nm modes) (8.5.4)
It then follows from the rest of the equations (8.3.8) that:
H x (x, y) = H 1 sin k x x cos k y y
H y (x, y) = H 2 cos k x x sin k y y
where we defined the constants:
H 1 = jβk x
k 2 c
H 0 ,
H 2 = jβk y
k 2 c
H 0
or
E x (x, y) = E 1 cos k x x sin k y y
E y (x, y) = E 2 sin k x x cos k y y
(8.5.5)
E 1 = η TE H 2 = jη ωk y
ω c k c
H 0 ,
E 2 =−η TE H 1 =−jη ωk x
ω c k c
H 0