Waveguides - ieeetsu

www.ece.rutgers.edu/∼orfanidi/ewa 265
266 Electromagnetic Waves & Antennas – S. J. Orfanidis – June 21, 2004
TE modes
TE modes are characterized by the conditions E z = 0 and H z ≠ 0. It follows from the
second of Eqs. (8.1.17) that E T is completely determined from H T , that is, E T = η TE H T ×ẑ.
The field H T is determined from the second of (8.1.16). Thus, all field components
for TE modes are obtained from the equations:
∇T 2 H z + k 2 cH z = 0
H T =− jβ
k 2 ∇ T H z
c
E T = η TE H T × ẑ
(TE modes) (8.3.6)
The relationship of E T and H T is identical to that of uniform plane waves propagating
in the z-direction, except the wave impedance is replaced by η TE . The Poynting vector
of Eq. (8.2.2) then takes the form:
P z = 1 2 Re(E T × H ∗ T )·ẑ = 1 |E T | 2 = 1 2η TE 2 η TE|H T | 2 = 1 2 η β 2
TE
k 4 |∇ T H z | 2 (8.3.7)
c
The cartesian coordinate version of Eq. (8.3.6) is:
∇ 2 T E z + k 2 c E z = 0
E T =− jβ
k 2 ∇ T E z
c
H T = 1 ẑ × E T
η TM
(TM modes) (8.3.10)
Again, the relationship of E T and H T is identical to that of uniform plane waves
propagating in the z-direction, but the wave impedance is now η TM . The Poynting vector
takes the form:
P z = 1 2 Re(E T × H ∗ T )·ẑ = 1 |E T | 2 = 1 β 2
2η TM 2η TM k 4 |∇ T E z | 2 (8.3.11)
c
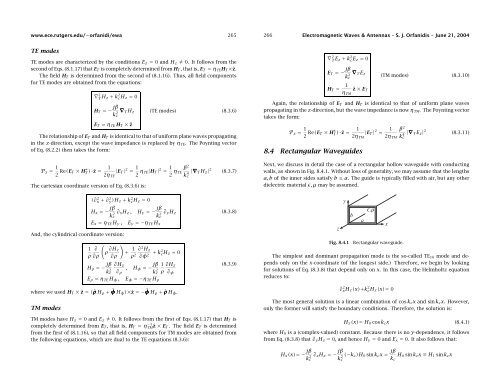
8.4 Rectangular Waveguides
Next, we discuss in detail the case of a rectangular hollow waveguide with conducting
walls, as shown in Fig. 8.4.1. Without loss of generality, we may assume that the lengths
a, b of the inner sides satisfy b ≤ a. The guide is typically filled with air, but any other
dielectric material ɛ, µ may be assumed.
(∂ 2 x + ∂ 2 y)H z + k 2 cH z = 0
H x =− jβ ∂ x H z ,
H y =− jβ ∂ y H z
(8.3.8)
k 2 c
k 2 c
And, the cylindrical coordinate version:
(
1 ∂
ρ ∂H )
z
+ 1 ∂ 2 H z
ρ ∂ρ ∂ρ ρ 2 ∂φ + 2 k2 c H z = 0
H ρ =− jβ ∂H z
k 2 , H φ =− jβ 1 ∂H z
(8.3.9)
c ∂ ρ k 2 c ρ ∂ φ
E ρ = η TE H φ , E φ =−η TE H ρ
where we used H T × ẑ = (ˆρH ρ + ˆφH φ )×ẑ =−ˆφH ρ + ˆρH φ .
TM modes
TM modes have H z = 0 and E z ≠ 0. It follows from the first of Eqs. (8.1.17) that H T is
completely determined from E T , that is, H T = η −1
TMẑ × E T. The field E T is determined
from the first of (8.1.16), so that all field components for TM modes are obtained from
the following equations, which are dual to the TE equations (8.3.6):
Fig. 8.4.1
Rectangular waveguide.
The simplest and dominant propagation mode is the so-called TE 10 mode and depends
only on the x-coordinate (of the longest side.) Therefore, we begin by looking
for solutions of Eq. (8.3.8) that depend only on x. In this case, the Helmholtz equation
reduces to:
∂ 2 xH z (x)+k 2 cH z (x)= 0
The most general solution is a linear combination of cos k c x and sin k c x. However,
only the former will satisfy the boundary conditions. Therefore, the solution is:
H z (x)= H 0 cos k c x (8.4.1)
where H 0 is a (complex-valued) constant. Because there is no y-dependence, it follows
from Eq. (8.3.8) that ∂ y H z = 0, and hence H y = 0 and E x = 0. It also follows that:
H x (x)= − jβ
k 2 c
∂ x H z =− jβ
k 2 (−k c )H 0 sin k c x = jβ H 0 sin k c x ≡ H 1 sin k c x
c
k c