A Toy Model of Chemical Reaction Networks - TBI - Universität Wien

5.3. NETWORK PROPERTIES 45
O 3
NO 3
NO 2
O 2
O 3
NO 3
NO 2
O 2
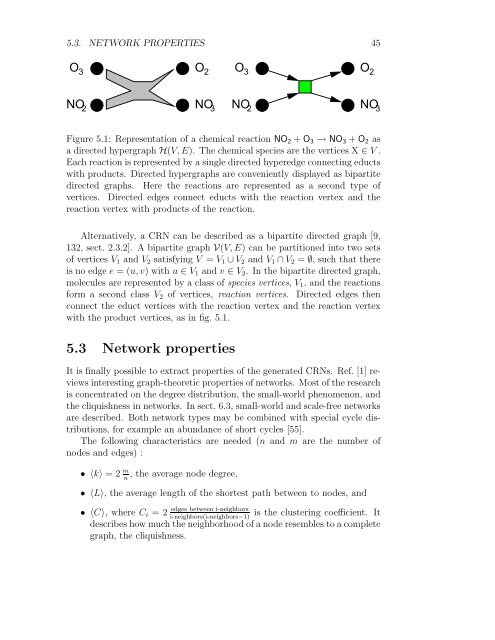
Figure 5.1: Representation of a chemical reaction NO 2 + O 3 → NO 3 + O 2 as
a directed hypergraph H(V, E). The chemical species are the vertices X ∈ V .
Each reaction is represented by a single directed hyperedge connecting educts
with products. Directed hypergraphs are conveniently displayed as bipartite
directed graphs. Here the reactions are represented as a second type of
vertices. Directed edges connect educts with the reaction vertex and the
reaction vertex with products of the reaction.
Alternatively, a CRN can be described as a bipartite directed graph [9,
132, sect. 2.3.2]. A bipartite graph V(V, E) can be partitioned into two sets
of vertices V 1 and V 2 satisfying V = V 1 ∪ V 2 and V 1 ∩ V 2 = ∅, such that there
is no edge e = (u, v) with u ∈ V 1 and v ∈ V 2 . In the bipartite directed graph,
molecules are represented by a class of species vertices, V 1 , and the reactions
form a second class V 2 of vertices, reaction vertices. Directed edges then
connect the educt vertices with the reaction vertex and the reaction vertex
with the product vertices, as in fig. 5.1.
5.3 Network properties
It is finally possible to extract properties of the generated CRNs. Ref. [1] reviews
interesting graph-theoretic properties of networks. Most of the research
is concentrated on the degree distribution, the small-world phenomenon, and
the cliquishness in networks. In sect. 6.3, small-world and scale-free networks
are described. Both network types may be combined with special cycle distributions,
for example an abundance of short cycles [55].
The following characteristics are needed (n and m are the number of
nodes and edges) :
• 〈k〉 = 2 m , the average node degree,
n
• 〈L〉, the average length of the shortest path between to nodes, and
edges between i-neighbors
• 〈C〉, where C i = 2 is the clustering coefficient. It
i-neighbors(i-neighbors−1)
describes how much the neighborhood of a node resembles to a complete
graph, the cliquishness.