Flux and Gauss' Law
Flux and Gauss' Law
Flux and Gauss' Law
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
PHY110W ELECTRICITY SUPPLEMENTARY GAUSS’ LAW<br />
<strong>Flux</strong> <strong>and</strong> Gauss’ <strong>Law</strong><br />
<strong>Flux</strong> is related to field lines.<br />
Imagine that you can see electric field lines… (Put on the special glasses if you have to!)<br />
Now put a non-metallic object (like a balloon) in the field, so that the field lines pass right through the object.<br />
You’ll notice that some field lines enter the object, some exit it <strong>and</strong> some just skim the surface.<br />
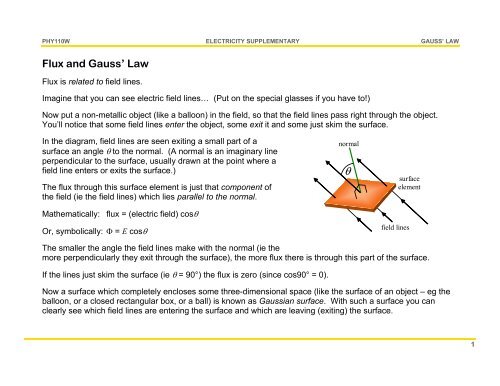
In the diagram, field lines are seen exiting a small part of a<br />
surface an angle θ to the normal. (A normal is an imaginary line<br />
perpendicular to the surface, usually drawn at the point where a<br />
field line enters or exits the surface.)<br />
The flux through this surface element is just that component of<br />
the field (ie the field lines) which lies parallel to the normal.<br />
Mathematically: flux = (electric field) cosθ<br />
Or, symbolically: Φ = E cosθ<br />
normal<br />
θ<br />
surface<br />
element<br />
field lines<br />
The smaller the angle the field lines make with the normal (ie the<br />
more perpendicularly they exit through the surface), the more flux there is through this part of the surface.<br />
If the lines just skim the surface (ie θ = 90°) the flux is zero (since cos90° = 0).<br />
Now a surface which completely encloses some three-dimensional space (like the surface of an object – eg the<br />
balloon, or a closed rectangular box, or a ball) is known as Gaussian surface. With such a surface you can<br />
clearly see which field lines are entering the surface <strong>and</strong> which are leaving (exiting) the surface.<br />
1
PHY110W ELECTRICITY SUPPLEMENTARY GAUSS’ LAW<br />
We make things mathematically simpler (!) by turning the normal into<br />
the elemental area vector, dA , whose magnitude is equal to the area<br />
of the surface element, <strong>and</strong> whose direction is always out of the<br />
surface (at right angles to it, like the normal).<br />
So field lines which enter the Gaussian surface (for which θ is larger<br />
than 90°) result in negative flux (because cosθ is now negative).<br />
And, representing the field by the electric field vector, E , we can now<br />
write the equation even more simply (!) as:<br />
<br />
Φ = E ⋅dA<br />
area vector,<br />
<br />
dA<br />
θ<br />
electric field<br />
vector, E<br />
To find the flux through a whole area, we must sum the flux through each of<br />
the elements which makes up that area, or (as the size of each element → 0)<br />
integrate over the whole area:<br />
<br />
total Φ = ∫ E ⋅dA<br />
<br />
If the whole area is a complete Gaussian surface, we write: Φ = ∫ E ⋅dA<br />
,<br />
where Φ now represents the net flux through the Gaussian surface.<br />
The importance of this equation is that Gauss’ <strong>Law</strong> tells us that the net flux through a Gaussian surface is<br />
proportional to the charge enclosed by that surface, or mathematically: ε<br />
0Φ = qenclosed<br />
. Which gives us…<br />
Gauss’ <strong>Law</strong>:<br />
<br />
ε E⋅ dA=<br />
q<br />
∫<br />
0 enclosed<br />
…<strong>and</strong> from this we derive useful equations for the electric field due to various charge distributions.<br />
2
PHY110W ELECTRICITY SUPPLEMENTARY GAUSS’ LAW<br />
Types of questions<br />
Questions on this section usually fall into one of three categories:<br />
1. Calculating the flux through some given surface (maybe part of a bigger, complete, Gaussian surface).<br />
[This type of question is a bit artificial <strong>and</strong> is really just for practice.]<br />
2. Working with the net flux through an entire Gaussian surface <strong>and</strong> applying Gauss’ <strong>Law</strong> to find the<br />
enclosed charge (or vice versa).<br />
3. Using the principle of Gauss’ <strong>Law</strong> to determine the electric field due to some distribution of charge.<br />
[Which is the main purpose of this section – determining electric fields – so that we can easily determine<br />
the force on a charged particle, <strong>and</strong> from here the amount of work done in moving it, etc etc.]<br />
Examples:<br />
* * * * *<br />
Type 1. A cubic Gaussian surface is bounded by the planes at x = 0.40 m,<br />
y = 0.60 m <strong>and</strong> z = 0.40 m as shown in the sketch. The electric field in this<br />
region is non-uniform <strong>and</strong> is given by E = (2.00 + 3.00x 2 )î N/C. Calculate<br />
the flux through the right h<strong>and</strong> face (ie the shaded face) of the cube.<br />
<br />
Solution: Φ= ∫ E ⋅dA<br />
Over the whole shaded surface x = 0.40 m, so the electric field is a<br />
constant (2.00 + 3.00 × 0.40 2 ) = 2.48 N/C <strong>and</strong> lies in the positive x<br />
direction, ie parallel to the area vector, so cosθ = 1, <strong>and</strong> we get<br />
0.6<br />
0.2<br />
y<br />
z 0.4<br />
0.4<br />
Φ<br />
right<br />
= E∫ dA = E A = 2.48 × 0.40 2 = 0,397 N⋅m 2 /C<br />
[Strictly speaking, you should evaluate the dot product more formally using unit vectors.]<br />
x<br />
3
PHY110W ELECTRICITY SUPPLEMENTARY GAUSS’ LAW<br />
Type 2. A cubic Gaussian surface is bounded by the planes at x = 0.40 m,<br />
y = 0.60 m <strong>and</strong> z = 0.40 m as shown in the sketch. The electric field in this<br />
region is non-uniform <strong>and</strong> is given by E = (2.00 + 3.00x 2 )î N/C. Calculate:<br />
0.6<br />
y<br />
(a)<br />
the flux through each face of the cube;<br />
0.2<br />
(b) the net charge enclosed by the cube.<br />
<br />
Solution: (a) This time we must apply Φ = E ⋅dA<br />
over each of the six faces –<br />
∫<br />
z 0.4<br />
0.4<br />
which is actually not as much work as it seems, because the field has no components in the y<br />
or z directions, so there will be no flux through the top, bottom, front <strong>and</strong> back faces:<br />
Ie Φ top = Φ bottom = Φ front = Φ back = 0 N⋅m 2 /C<br />
We’ve already calculated the flux through the right h<strong>and</strong> face (in Type 1), so we only have to<br />
follow the same procedure for the left face, where x = 0 m.<br />
Here the electric field is a constant (2.00 + 3.00 × 0 2 ) = 2.00 N/C <strong>and</strong> again lies in the positive<br />
x direction. This time, however, the area vector points in the negative x direction (because it<br />
always points out of the Gaussian surface), so cosθ = –1, <strong>and</strong> we get<br />
Φ<br />
left<br />
= E∫ dA =−E A = –2.0 × 0.40 2 = –0,32 N⋅m 2 /C<br />
(b) Using Gauss’ <strong>Law</strong>, qenclosed<br />
= ε<br />
0Φ (where Φ is the total flux through all six faces)…<br />
∴q enclosed = 8.85 × 10 -12 × (0 + 0 + 0 + 0 + 0.397 + (–0.32)) = +6.80 × 10 -13 C<br />
x<br />
4
PHY110W ELECTRICITY SUPPLEMENTARY GAUSS’ LAW<br />
Type 3.<br />
The diagram shows, in cross section, a central metal ball, two<br />
spherical metal shells, <strong>and</strong> three spherical Gaussian surfaces of<br />
radii R, 2R <strong>and</strong> 3R, all with the same centre. The charges on the<br />
three objects are: ball, Q; smaller shell, 3Q; larger shell, 5Q.<br />
Show that the magnitude of the electric field at any point on any<br />
one of the surfaces is the same as at any other point on any of<br />
the surfaces.<br />
Shell<br />
R<br />
3R<br />
2R<br />
Gaussian<br />
surface<br />
Solution:<br />
There is no reference to first principles here, so we can simply use the equation for the electric<br />
field due to an enclosed point charge [which was derived earlier (p 17 of Lecture Notes) by<br />
<br />
applying Gauss’ <strong>Law</strong> (in the form ε<br />
0<br />
E⋅ dA = q ) to a point charged surrounded by a<br />
∫<br />
concentric Gaussian sphere of radius r]:<br />
E =<br />
enclosed<br />
q<br />
4πε<br />
r<br />
Q<br />
For the innermost Gaussian surface, r = R, <strong>and</strong> q (ie enclosed q) = Q, so E =<br />
2<br />
4πε<br />
R<br />
0<br />
2<br />
0<br />
For the next Gaussian surface, r = 2R, <strong>and</strong> the net enclosed charge is q = (Q + 3 Q), so<br />
4Q<br />
Q<br />
E = 2 2<br />
4 πε (2 R) = 4πε<br />
R<br />
, ie the same value as for points on the innermost surface.<br />
0 0<br />
And so on. (You should be able to finish it on your own now…)<br />
5