MAGNETIC FIELDS DUE TO CURRENTS

PHY110W MAGNETISM MAGNETIC FIELDS DUE TO CURRENTS
MAGNETIC FIELDS DUE TO CURRENTS
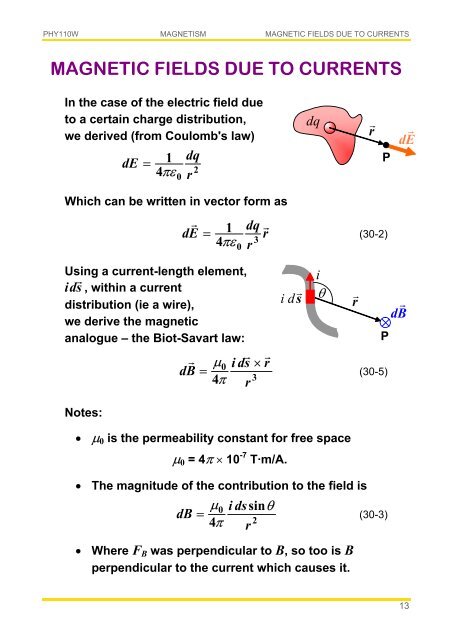
In the case of the electric field due
to a certain charge distribution,
we derived (from Coulomb's law)
dE
=
1
4πε
0
dq
2
r
dq
r
P
dE
Which can be written in vector form as
dE =
1
4πε
0
dq
r
3
r
(30-2)
Using a current-length element,
ids , within a current
distribution (ie a wire),
we derive the magnetic
analogue – the Biot-Savart law:
µ 0 ids
dB =
4π
r
Notes:
×
3
r
i
i ds θ
r dB
×
P
(30-5)
• µ 0 is the permeability constant for free space
µ 0 = 4π × 10 -7 T·m/A.
• The magnitude of the contribution to the field is
µ 0 idssinθ
dB
4π
2
r
= (30-3)
• Where F B was perpendicular to B, so too is B
perpendicular to the current which causes it.
13

PHY110W MAGNETISM MAGNETIC FIELDS DUE TO CURRENTS
MAGNETIC FIELD DUE TO CURRENT
IN A LONG, STRAIGHT WIRE
d s
i
s
θ
R
r
× dB
P
Using symmetry (every current element ds in the upper
half of the wire has a corresponding element in the
lower half causing the same field at P), the magnitude
of the magnetic field at point P due to a long, straight,
current-carrying wire is
∞
0i
2 4
µ
B = sinθ
ds
π ∫ (30-7)
2
r
0
= ° − =
R
, and
r
where sinθ
sin( 180 θ)
∴ B =
µ 0i
2π
∞
∫
0
R
(
2 2)
s
+
R
32
ds
2 2
r = s + R
∞
µ 0i
⎡ s ⎤
∴ B = 12
2π
R ⎢( 2 2
s R ) ⎥
⎣ + ⎦0
µ 0i
∴ B = (30-6)
2π
R
The direction of B is given by the RH curled fingers rule.
14

PHY110W MAGNETISM MAGNETIC FIELDS DUE TO CURRENTS
THE MAGNETIC FIELD AROUND A LONG,
STRAIGHT, CURRENT-CARRYING WIRE
i
B
r
The force a current-carrying wire experiences in an
external magnetic field is therefore due to the
superpositioning of the two magnetic fields:
N
S
…
N
F
S
15

PHY110W MAGNETISM MAGNETIC FIELDS DUE TO CURRENTS
MAGNETIC FIELD DUE TO CURRENT
IN A CIRCULAR WIRE
i
R
α
x
r
dB y
P
α
dB
dB x
From the Biot-Savart law, the field at point P (along the
axis of the a circular loop, radius R), due to a differential
current element ids at the top of the loop, is given by
µ 0 idssinα
µ 0i
ds
dB = =
2
( 2 2
4π
r 4π
R + x )
By symmetry, all the components perpendicular to the
axis (dB y ) sum to zero, so we need consider only dB x :
dB
x
µ 0i
ds µ 0i
ds R
= cosα
=
4π
R + x 4 R + x R + x
( 2 2) π ( 2 2) ( 2 2)
12
i, R and x have the same values for all elements around
the loop, so when we integrate around the circumference…
B
x
µ 0iR
=
4π
R + x
(
2 2)
32
∫ ds (and ∫ ds is just 2πR)
so
B
x
=
2
µ iR
0
2
(
2 2)
R
+
x
32
(30-28)
16

PHY110W MAGNETISM MAGNETIC FIELDS DUE TO CURRENTS
A CURRENT-CARRYING COIL
AS A MAGNETIC DIPOLE
By considering a cross-section
through a current-carrying coil, we
can see why such an arrangement
can be viewed as a magnetic dipole.
i
i out
i in
For a coil of N turns the magnetic
dipole moment was determined to be µ = NiA (29-33)
So the magnetic field along the axis of a currentcarrying
coil can be written as
B x
µ 0µ
=
2π
R + x
(
2 2)
and for large distances x >> R,
µ µ
B x
2π
x
32
0
= (30-29)
3
… which has the identical form to the expression for
the electric field along the axis of an electric dipole:
E
p
= (23-9)
3
2πε0z
A current loop produces a magnetic
field like that of a bar magnet and
thus has north and south poles.
The direction of µ is given by the
right hand curled fingers rule.
S
µ N
17

PHY110W MAGNETISM MAGNETIC FIELDS DUE TO CURRENTS
MAGNETIC FIELD DUE TO
A MOVING CHARGE
From the Biot-Savart law, the field due to a differential
current element ids is given by
µ 0 ids×
r
dB =
4π
3
r
Setting ds =
dQ
v dt , and i = , we get
dt
µ 0 dQ v × r
dB =
4π
3
r
If we define the differential charge element dQ as the
charge on a single particle contributing to the current,
then the magnetic field due to a moving charged
particle is given by
µ 0 qv × r
B =
4π
3
r
As before, the direction of B is given by the direction
of the cross product v × r , or the right hand curled
fingers rule.
18

PHY110W MAGNETISM MAGNETIC FIELDS DUE TO CURRENTS
FORCE BETWEEN
TWO PARALLEL CONDUCTORS
d
B L
F a
b
ba
F
ab
B
a
i a
i b
b
The magnitude of the magnetic field due to the current
in wire a at every point on wire b is given by
B
a
µ 0ia
=
2π
d
and consequently the magnitude of the force on wire b
due to the magnetic field of wire a (written F ba ) is
µ 0Liaib
Fba = iLB b a = = Fab
(30-15)
2π
d
• F ba and F ab are an action-reaction pair.
• Parallel currents attract.
• Antiparallel currents repel.
The ampere can now be defined as …
that unvarying current which, when maintained in each
of two parallel conductors of infinite length and situated
one metre apart in empty space, causes between them a
force of exactly 2 × 10 -7 N per metre of length.
19

PHY110W MAGNETISM MAGNETIC FIELDS DUE TO CURRENTS
AMPERE’S LAW
Gauss’s law related the surface integral of the electric
flux over a closed (Gaussian) surface to the net amount
of charge enclosed by that surface.
Ampere’s law relates the line integral of the magnetic
field around a closed (Amperian) loop to the net
amount of current enclosed by that loop.
Mathematically: ∫ B
⋅ ds = µ 0iencl
(30-16)
Amperian
loop
i 3
i 1
i 2
ds
θ
B
According to the right hand curled fingers rule, as we
integrate anticlockwise around the above loop i 1 is
regarded as positive, while i 2 is negative.
i 3 is ignored because it is not enclosed by the loop.
B⋅ ds = Bdscosθ = µ i − i
∫
∫
0 1 2 (30-18)
So: ( )
In the same way Gauss’s law helped to determine the
magnitude of electric fields due to symmetrical charge
distributions, Ampere’s law is useful in determining the
magnitude of the resultant magnetic fields due to
current distributions which have some symmetry…
20

PHY110W MAGNETISM MAGNETIC FIELDS DUE TO CURRENTS
APPLICATIONS OF AMPERE’S LAW I
Magnetic field outside a long, straight conductor
For a long, straight wire
carrying current directly
out of the page the
symmetry dictates that we
surround the wire with a
concentric circular
Amperian loop of radius r.
Amperian
loop
i
ds B
r
B lies at a tangent to the loop everywhere on the loop,
so the angle θ between B and ds is zero.
∴ B⋅ ds = B ds = B2π
r
∫
∫
And applying Ampere’s law:
B2π r = µ ( ) 0 + i
So the magnitude of the magnetic field around a long,
straight, current-carrying conductor is
B
µ i
2π
r
0
= (30-19)
(Which agrees exactly with what was derived from the
Biot-Savart law.)
21

PHY110W MAGNETISM MAGNETIC FIELDS DUE TO CURRENTS
APPLICATIONS OF AMPERE’S LAW II
Magnetic field of a solenoid
A solenoid is a long, straight, closely
wound helical coil of wire, usually
with a constant diameter which is
much smaller than its length.
For an ideal solenoid
(of infinite length, and no
leakage) the magnetic
field outside the solenoid
is zero, while the field
lines inside are straight
and uniformly spaced.
D
A
h
C
B
B
Using the rectangular Amperian loop ABCDA,
B C D A
B ⋅ ds = B ⋅ ds + B ⋅ ds + B ⋅ ds + B ⋅ ds = Bh
∫ ∫ ∫ ∫ ∫
A B C D
Applying Ampere’s law to a solenoid with n turns per
metre and carrying current i we get:
Bh
= µ i ( nh)
So the magnitude of the magnetic field inside an
infinitely long solenoid is
0
B
= µ 0ni
(30-25)
The equation will work for real solenoids provided it is
applied to interior points well away from the ends.
22