14.4. Normal Curvature and the Second Fun- damental Form

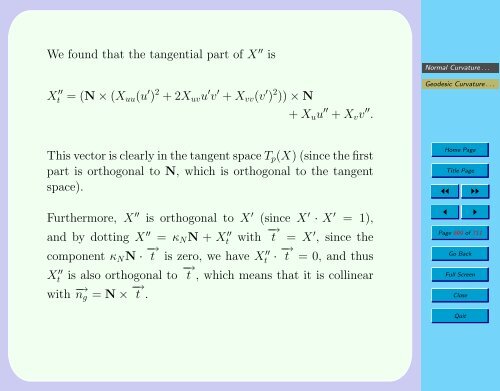
We found that the tangential part of X ′′ is
Normal Curvature . . .
X ′′
t
= (N × (X uu (u ′ ) 2 + 2X uv u ′ v ′ + X vv (v ′ ) 2 )) × N
+ X u u ′′ + X v v ′′ .
Geodesic Curvature . . .
This vector is clearly in the tangent space T p (X) (since the first
part is orthogonal to N, which is orthogonal to the tangent
space).
Furthermore, X ′′ is orthogonal to X ′ (since X ′ · X ′ = 1),
and by dotting X ′′ = κ N N + X t ′′ with −→ t = X ′ , since the
component κ N N · −→ t is zero, we have X t ′′ · −→ t = 0, and thus
X ′′
t
is also orthogonal to −→ t , which means that it is collinear
with −→ n g = N × −→ t .
Home Page
Title Page
◭◭ ◮◮
◭
◮
Page 699 of 711
Go Back
Full Screen
Close
Quit