2001 Santibañez - PD control with feedforward compensation for robot manipulators analysis and experimentation.pdf

5 66 IEEE TRANSACTIONS ON ROBOTICS AND AUTOMATION, VOL. IO. NO. 4, AUGUST 1994
rectangular
elliptical
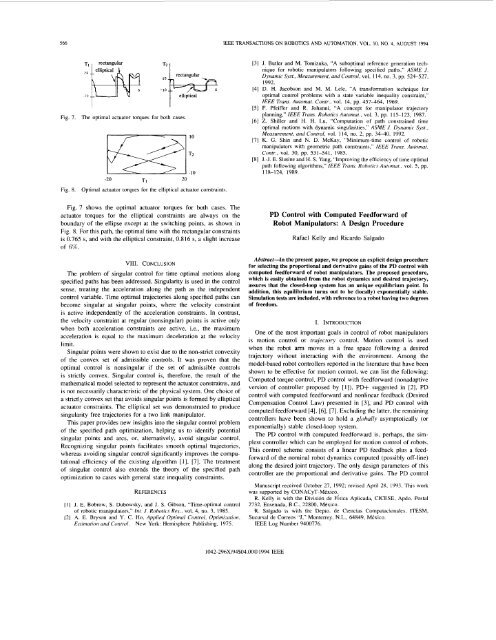
Fig. 7. The optimal actuator torques for both cases.
10
T2
-10
[31 J. Butler and M. Tomizuka, “A suboptimal reference generation teehnique
for robotic manipulators following specified paths,” ASME J.
Dynamic Syst., Measurement, and Control. vol. 114. no. 3, pp. 524527,
1992.
[4] D. H. Jacobson and M. M. Lele, “A transformation technique for
optimal control problems with a state variable inequality constraint,”
IEEE Trans. Automat. Contr.. vol. 14, pp. 457464, 1969.
[5] F. Pfeiffer and R. Johanni, “A concept for manipulator trajectory
planning,” IEEE Trans. Rohotics Automat., vol. 3, pp. 115-123, 1987.
161 Z. Shiller and H. H. Lu, ‘Computation of path constrained time
optimal motions with dynamic singularities,” ASME J. Dyntrmic Syst..
Measurement, and Control, vol. 114, no. 2, pp. 3440, 1992.
[7] K. G. Shin and N. D. McKay, “Minimum-time control of robotic
manipulators with geometric path constraints,” lEEE Truns. Automat.
Contr., vol. 30, pp. 531-541, 1985.
[8] J.-J. E. Slotine and H. S. Yang, “Improving the efficiency of time optimal
path following algorithms,” IEEE Trans. Robotic,.\ Automat.. vol. 5, pp.
118-124, 1989.
Fig. 8. Optimal actuator torques for the elliptical actuator constraints
Fig. 7 shows the optimal actuator torques for both cases. The
actuator torques for the elliptical constraints are always on the
boundary of the ellipse except at the switching points, as shown in
Fig. 8. For this path, the optimal time with the rectangular constraints
is 0.765 s, and with the elliptical constraint, 0.816 s, a slight increase
of 6‘,%.
VIII. CONCLUSION
The problem of singular control for time optimal motions along
specified paths has been addressed. Singularity is used in the control
sense, treating the acceleration along the path as the independent
control variable. Time optimal trajectories along specified paths can
become singular at singular points, where the velocity constraint
is active independently of the acceleration constraints. In contrast,
the velocity constraint at regular (nonsingular) points is active only
when both acceleration constraints are active, i.e., the maximum
acceleration is equal to the maximum deceleration at the velocity
limit.
Singular points were shown to exist due to the non-strict convexity
of the convex set of admissible controls. It was proven that the
optimal control is nonsingular if the set of admissible controls
is strictly convex. Singular control is, therefore, the result of the
mathematical model selected to represent the actuator constraints, and
is not necessarily characteristic of the physical system. One choice of
a strictly convex set that avoids singular points is formed by elliptical
actuator constraints. The elliptical set was demonstrated to produce
singularity free trajectories for a two link manipulator.
This paper provides new insights into the singular control problem
of the specified path optimization, helping us to identify potential
singular points and arcs, or, altematively, avoid singular control.
Recognizing singular points facilitates smooth optimal trajectories,
whereas avoiding singular control significantly improves the computational
efficiency of the existing algorithm [ 11, [7]. The treatment
of singular control also extends the theory of the specified path
optimization to cases with general state inequality constraints.
REFERENCES
I I I J. E. Bobrow, S. Dubowsky, and J. S. Gibson, “Time-optimal control
of robotic manipulators,” Int J. Rohotics Res.. vol. 4, no. 3, 1985.
(21 A. E. Bryson and Y. C. Ho, Applied Optimal Control. Optimizcttion,
Estimation and Control. New York: Hemisphere Publishing, 1975.
PD Control with Computed Feedforward of
Robot Manipulators: A Design Procedure
Rafael Kelly and Ricardo Salgado
Abstract-In the present paper, we propose an explicit design procedure
for selecting the proportional and derivative gains of the PD control with
computed feedforward of robot manipulators. The proposed procedure,
which is easily obtained from the robot dynamics and desired trajectory,
assures that the closed-loop system has an unique equilibrium point. In
addition, this equilibrium turns out to be (locally) exponentially stable.
Simulation tests are included, with reference to a robot having two degrees
of freedom.
I. INTRODUCTION
One of the most important goals in control of robot manipulators
is motion control or trajectory control. Motion control is used
when the robot arm moves in a free space following a desired
trajectory without interacting with the environment. Among the
model-based robot controllers reported in the literature that have been
shown to be effective for motion control, we can list the following:
Computed torque control, PD control with feedforward (nonadaptive
version of controller proposed by [I]). PD+ suggested in [2], PD
control with computed feedforward and nonlinear feedback (Desired
Compensation Control Law) presented in [3], and PD control with
computed feedforward [4], [6],[7]. Excluding the latter, the remaining
controllers have been shown to hold a glohal/y asymptotically (or
exponentially) stable closed-loop system.
The PD control with computed feedforward is, perhaps, the simplest
controller which can be employed for motion control of robots.
This control scheme consists of a linear PD feedback plus a feedforward
of the nominal robot dynamics computed (possibly off-line)
along the desired joint trajectory. The only design parameters of this
controller are the proportional and derivative gains. The PD control
Manuscript received October 27, 1992; revised April 28, 1993. This work
was supported by CONACyT-Mtxico,
R. Kelly is with the Division de Fisiea Aplicada, CICESE, Apdo. Postal
2732. Ensenada, B.C., 22800, Mtxico.
R. Salgado is with the Depto. de Cieneias Computacionales, ITESM,
Sucursal de Correos “I,” Monterrey, N.L., 64849, Mexico.
IEEE Log Number 9400776.
1042-29hX/94$04.000 1994 IEEE

IEEE TRANSACTIONS ON ROBOTICS AND AUTOMATION, VOL. 10, NO. 4, AUGUST 1994 561
with computed feedforward has the nice property that it reduces to
PD control with desired gravity compensation in the particular case
of position control.
The stability analysis of the PD control with computed feedforward
of robot manipulators has been performed previously. Namely. in 141,
the study of a large class of robot controllers is addressed, including
PD control with computed feedforward. The adaptive version of this
controller was given at the same time in [5]. Reference [6] treats the
analysis of a robot controller based on a positive-real approach. which
includes the PD controller with computed feedforward as a particular
case. A unified framework to study a set of PD-type controllers was
developed in [7]. This was carried out by considering an energybased
Lyapunov function, and again, the PD control with computed
feedforward is contained in the set of controllers under study. The
conclusions of the above works can be summarized as follows: The
closed-loop system is locally exponentially stable provided that the
proportional and derivative gains are sufficiently large.
On the other hand, experimental results of this controller have
been presented in [SI-[IO]. where by selecting the proportional and
derivative gains sufficiently high, the performance obtained is not
only better than using only PD, but equals that of the computed
torque technique.
In view of the simplicity and applicability of the PD controller
with computed feedforward, it is of interest to dispose of guidelines
for tuning the controller parameters. This is the main objective
of this paper. Our contribution is a design procedure which gives
explicit lower bounds on the proportional and derivative gains that
guarantee stability of the closed-loop dynamics. These bounds are
easily obtained form the robot dynamics and on the dmesired trajectory.
The design procedure was obtained first giving conditions in order
to guarantee equilibrium uniqueness. and second, by proposing a
Lyapunov function to show local exponential stability. Thus, this
design guideline assures that the closed-loop system has only one
equilibrium which is locally exponentially stable.
Throughout this paper, we use the notation A7,t{.4} and A,,L{.4}
to indicate the smallest and largest eigenvalues, respectively. of a
symmetric positive definite bounded matrix A(x). for any x E R".
The norm of vector x is defined as llxll = J- x7'x and that of
matrix .-1 is defined as the corresponding induced norm (1.4)1 =
JxJxTg.
The remaining part of this paper is organized as follows. In Section
I1 we present the robot dynamic model and the control law. The
stability analysis and the design guideline are given in Section 111.
Computer simulations on a two-DOF robot are presented in Section
IV, and finally, we offer brief concluding remarks in Section V.
11. ROBOT DYNAMIC htODEL AND CONTROL PROBLEM FORMULATlOh
In the absence of friction and other disturbances, the dynamics of
a serial n-link rigid robot can be written as [ 1 I]:
U(q)ii + C(q. q,q + g(q) = 7 (1)
where q is the 12 x 1 vector of joint displacements, T Is the it x 1 vector
of applied torques, M(q) is the 71 x n symmetric positive definite
manipulator inertia matrix, C(q. q)q is the rt x 1 vector of centripetal
and Coriolis torques, and g(q) is the n x 1 vector of gravitational
torques. We assume that the links are jointed together with revolute
joints and matrix C(q. q) is defined using the Christoffel symbols.
Although the equation of motion (1) is complex. it has several
fundamental properties which can be exploited to facilitate control
system design. These propertjes are stated in the following.
Propc~y I: The matrix- M(q) - 2C(q. q) is skewsjnzmrtric.
This inzplies M(q) =: C(q.q) + C(q,q)".
Property 2 There eAkt positive constants IC I
TUC h that for all x, y, z. v, w E W, we have
1) IIM(x)z - ~\f(Y)ZlI i k.hlIIX - YII IIZII
2) IlC(x. z)w - C(Y3 Vbll I k.c-, llz - VI1 llwll
+ kc2 llzll Ilx - YII IIWII
3 II~(X1Y)Zll i kc 1 llYll llzll
4) Ildx) - dY)II I k.,Ilx - Yll.
Furthermore, the constants k ZI , kc,, kr
as
k,., , kc, and IC,
and k , can be computed
where :UtJ(q) is the ij-element of matrix M(q), r,,k(q) is the ijk
Christoffel symbol, and gz(q) is the i-element of vector g(q).
Now, we are in position to formulate the control problem. Let q

568 IEEE TRANSACTIONS ON ROBOTICS AND AUTOMATION, VOL. 10, NO. 4, AUGUST 1994
A. Equilibrium Uniqueness
In order to guarantee that the origin is the unique equilibrium, the
matrix I kz/llqdl.2r + kv211qdll;f + k,.
thus, under this choice on I

IEEE TRANSACTIONS ON ROBOTICS AND AUTOMATION, VOL. 10, NO. 4, AUGUST 1994 569
unique equilibrium of the closed-loop system. Also,. condition (13)
on I

570 IEEE TRANSACTIONS ON ROBOTICS AND AUTOMATION, VOL. 10, NO. 4, AUGUST 1994
o.21
0. I
initial position
Fig. 4.
End-effector path
-0.05 *v I I I -
-0.05 0 0. I 0.2 0.3
o.# Y (m)
The elements of gravitational torque vector g(q) are given by
g1(q) = (7)?11,1 + tIl.Lll).q C'os(q1)
+ tt121r2.f/ ('04'll + q 2)
yn(q) = rn2l,sg ros(ql + ~ 2 ) .
The motion problem considered is that of the robot end-ffector
tracks a point on a circle centered at y = L = 0.2 ni and radius 0.073
m which tums two times per second. This task can be expressed in
terms of the Cartesian coordinates as
3 .
y

IEEE TRANSACTIONS ON ROBOTICS AND AUTOMATION, VOL. 10, NO. 4, AUGUST 1994 571
ACKNOWLEDGMENT
The authors wish to thank Dr. H. Berghuis for insightful comments
and suggestions.
REFERENCES
J. J. Slotine and W. Li, “On the adaptive control of robot manipulators.”
Inr. .I. Robotics Res.. vol. 6, no. 3, pp. 49-59, 1987.
8. Paden and R. Panja, “Globally asymptotically stable PD+ controller
lor robot manipulators,” fnt. J. Control. vol. 47, no. 6, pp. 16961712,
1988.
N. Sadegh and R. Horowitz, “Stability and robustness analysis of a class
of adaptive controllers for robotic manipulators.” In(. J. Robotics Res..
vol. 9, no. 3, pp. 74-92, 1990.
J. T. Wen and D. S. Bayard, “New class of control laws for robotic
manipulators: Part 1. Non-adaptive case.” fnt. .I. Control, vol. 47. no.
5. pp. 1361-1385, 1988.
D. S. Bayard and J. T. Wen. “New class of control laws for robotic
manipulators: Part 2. Adaptive case,” Inr. J. Control. vol. 47, no. 5, pp.
1387- 1406. 1988.
B. Paden and B. Riedle, “A positive-real modification of a class of
nonlinear controllers for robot manipulators,” in Pro(.. Amer. Control
Cmf.. Atlanta. GA, 1988. pp. 1782-1785.
J. T. Wen, “A unified perspective on robot control: The energy Lyapunov
function approach,” Inr. J. Adapfive Control Signal Processing, vol. 4.
no. 6, pp. 487-500, 1990.
C. H. An, C. G. Atkeson, and J. M. Hollerbach, Model-Based Control
of Robot ManipUkJfOrX Cambridge, MA: MIT Press, 1988.
T. Kokkinis and R. Stoughton. “Dynamics and control of a closedchain
robot arms with application to a new direct-drive robot arm,” Int.
J. Robotics Autom., vol. 6, no. I, pp. 25-34. 1991.
T. J. Tam, A. K. Bejczy, G. T. Marth, and A. K. Ramadorai, “Performance
comparison of four manipulator servo controllers,” lEEE Control
SystPms, vol. 13. pp. 22-29, 1993.
M. Spong and M. Vidyasagar, Robot Dynamics and Control. New
York: Wiley, 1989.
Y. H. Chen and G. Leitmann, “Robustness of uncertain systems in absence
of matching assumptions,” Int. J. Control. vol. 45, pp. 1527-1542,
1987.
H. Khalil, Nonlinear Sysfems.
New York: MacMillan, 1992.
2. Qu and J. Dorsey. “Robust tracking control of robots by a linear
feedback law,” IEEE Trans. Automatic Control. vol. 36, pp. 1081-1084.
1991.
T. Ozaki, T. Suzuki, T. Furuhashi, S. Okuma, and Y. Uchikawa,
“Trajectory control of robotic manipulators using neural networks.”
fEEE Trans. [nd. E1ec.t.. vol. 38, pp. 195-202, 1991.