Ad Quadratum Construction and Study of the Regular Polyhedra

95
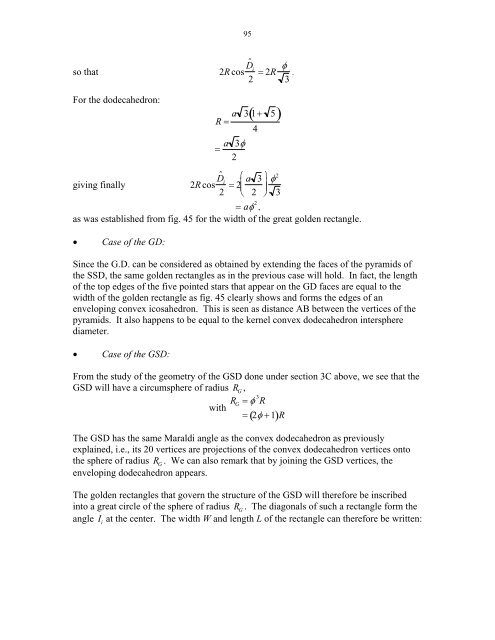
so that 2R cos ˆ
D i
2
For the dodecahedron:
R
2R
3 .
a 315 4
a 3
2
giving finally 2R cos ˆ D
i a 3
2 2
2
2
3
2
a ,
as was established from fig. 45 for the width of the great golden rectangle.
Case of the GD:
Since the G.D. can be considered as obtained by extending the faces of the pyramids of
the SSD, the same golden rectangles as in the previous case will hold. In fact, the length
of the top edges of the five pointed stars that appear on the GD faces are equal to the
width of the golden rectangle as fig. 45 clearly shows and forms the edges of an
enveloping convex icosahedron. This is seen as distance AB between the vertices of the
pyramids. It also happens to be equal to the kernel convex dodecahedron intersphere
diameter.
Case of the GSD:
From the study of the geometry of the GSD done under section 3C above, we see that the
GSD will have a circumsphere of radius RG ,
with RG 3 R
21R The GSD has the same Maraldi angle as the convex dodecahedron as previously
explained, i.e., its 20 vertices are projections of the convex dodecahedron vertices onto
the sphere of radius R G . We can also remark that by joining the GSD vertices, the
enveloping dodecahedron appears.
The golden rectangles that govern the structure of the GSD will therefore be inscribed
into a great circle of the sphere of radius R G . The diagonals of such a rectangle form the
angle I i at the center. The width W and length L of the rectangle can therefore be written: