Ad Quadratum Construction and Study of the Regular Polyhedra

More documents

Recommendations

Info

Icosahedron: r ri 2 1 3 1 38 0.850 r D ˆ i cos R 2 cos I ˆ i ri I ˆ i but cos 2 R 2 ; r D ˆ i so that: cos ri 2 ; and, as previously established we have cos ˆ D i 2 r ri 0.934 As an effect of the duality principle we can see that for the tetrahedron ri r R ri 1 3 cosCi cos Ti 2 for the cube and the octahedron ri R cube r ri and r i R oct. r r i oct. 2 3 sinDi cos Ci 2 cube 1 2 1 tanI i ctnI i cos O i 2 for the dodecahedron and the icosahedron r i R doc. r r i icos. and r i R icos. r r i 3 cos Di 2 doc. 2 cos I i 2
Comparison with Orthoscheme Approach: 39 Schläfli 14 established general formulae giving the ratio between the radii of these spheres based on the study of the decomposition of the polyhedra in orthoschemes which are oppositely congruent tetrahedra making up our pyramids. The decomposition is made along the axis of the pyramid giving as many orthoschemes as sides to the face of the polyhedron under study. Furthermore, the four faces of these tetrahedra are right triangles, and the lengths of the three edges meeting at the polyhedron center are radii of the circumsphere, insphere, and intersphere respectively. 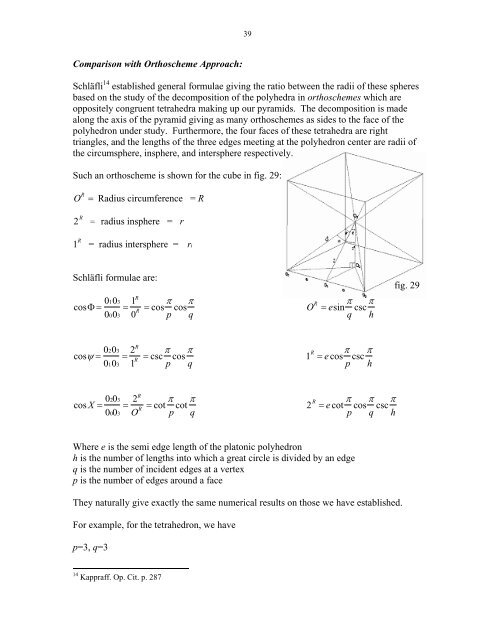Such an orthoscheme is shown for the cube in fig. 29: O R Radius circumference = R 2 R = radius insphere = r 1 R = radius intersphere = ri Schläfli formulae are: cos 0103 0003 1R 0 R cos p cos q OR esin csc q h cos 0203 0103 2R 1 R csc cos p q 1Recos csc p h cos X 0203 0003 2R O R cot cot p q 2Recot cos csc p q h Where e is the semi edge length of the platonic polyhedron h is the number of lengths into which a great circle is divided by an edge q is the number of incident edges at a vertex p is the number of edges around a face They naturally give exactly the same numerical results on those we have established. For example, for the tetrahedron, we have p=3, q=3 14 Kappraff. Op. Cit. p. 287 fig. 29
Page 1 and 2: The Louis and Jeanette Brooks Engin
Page 3 and 4: Copyright 2001 by Jean Le Mée
Page 5 and 6: Dodecahedron ......................
Page 7 and 8: Acknowledgements Special thanks are
Page 9 and 10: viii The realization of the project
Page 11 and 12: Introduction: 1 Ad- Quadratum Const
Page 13 and 14: Generation of the Platonic Forms: 1
Page 15 and 16: …which can be more briefly put as
Page 17 and 18: 7 Tetrahedron: Cube: Octahedron: Do
Page 19 and 20: Designate CH by x, then cos ˆ C i
Page 21 and 22: Dodecahedron: 11 For the dodecahedr
Page 23 and 24: with a 10 5 13 5 5R a 2 10 55R
Page 25 and 26: 15 A simple way of constructing eac
Page 27 and 28: Similarly, cosU ˆ O S 1 ,and ther
Page 29 and 30: C ˆ D ˆ O i 2 The cube dihedra
Page 31 and 32: 21 As indicated for the cube (fig.
Page 33 and 34: 23 or MO 5 2 3 R so that if we (ar
Page 35 and 36: Tetrahedron: For the tetrahedron, a
Page 37 and 38: a 6 6 R 2 6 6 27 R 12 6 r 3 3
Page 39 and 40: 29 r 0. 794 R The more complex for
Page 41 and 42: 31 Now, consider triangles AA HO a
Page 43 and 44: Dˆ i cos 2 33 We can therefor
Page 45 and 46: 35 Ratio of Intersphere to Circumsp
Page 47: Cube: Octahedron: Dodecahedron: r R
Page 51 and 52: 1R O R ri R 41 cos csc 3 3 cos
Page 53 and 54: 43 They are not what is generally t
Page 55 and 56: 45 Kepler (1571-1630) discovered in
Page 57 and 58: 47 We shall now determine the diame
Page 59 and 60: 49 The stellation process is one wh
Page 61 and 62: 51 i. Case of the Dodecahedron The
Page 63 and 64: 53 These dipyramids in turn can be
Page 65 and 66: 55 If the faces of the pyramids on
Page 67 and 68: 57 - the great dodecahedron by addi
Page 69 and 70: 59 and therefore through the vertic
Page 71 and 72: R e a the well-known relation in
Page 73 and 74: from Since ABCD is a golden rectang
Page 75 and 76: We have: Noting that cos Di 2 65 R
Page 77 and 78: 67 Fig. 46A shows such a pentagonal
Page 79 and 80: The inner geometry of the GD will b
Page 81 and 82: 71 2 2 2 Now H sin And sin 1c
Page 83 and 84: 73 GSD. Since the apexes of the pyr
Page 85 and 86: The circumsphere radius of the enve
Page 87 and 88: where R S is the circumsphere of th
Page 89 and 90: And since We verify that as establi
Page 91 and 92: 81 The intersection of these pyrami
Page 93 and 94: Now, as has just been established,
Page 95 and 96: 85 fig. 45
Page 97 and 98: We have tanw 87 2 2 1 1 4 1 5 Wi
Page 99 and 100:
89 The structure of this scaled G.I
Page 101 and 102:
the three mutually perpendicular go
Page 103 and 104:
93 For the dodecahedron, the golde
Page 105 and 106:
95 so that 2R cos ˆ D i 2 For the
Page 107 and 108:
Alternative Methods of Generating t
Page 109 and 110:
99 As Fernand Hallyn 25 points our,
Page 111 and 112:
101 held by their authors. From the
Page 113 and 114:
103 By joining each such vertex to
Page 115 and 116:
105 fig. 61
Page 117 and 118:
107 For the cube, select a pole on
Page 119 and 120:
109 fig. 63A fig. 63B fig. 63C
Page 121 and 122:
111 r 2 2 2.828 (We consider the
Page 123 and 124:
113 fig. 64 fig. 65 fig. 66
Page 125 and 126:
fig. 67 115 fig. 69 fig. 6 fig. 68
Page 127 and 128:
the structure of all regular polyhe
Page 129 and 130:
119 Ad Quadratum method and the Gen
Page 131 and 132:
121 fig. 74
Page 133 and 134:
123 Ad Quadratum and the Pythagorea
Page 135 and 136:
125 fig. 78
Page 137 and 138:
OT y 1 x 127 y So that, if OT is a
Page 139 and 140:
129 fruitful paradigm. For more tha
Page 141 and 142:
5 3 1 (unisson) 5 3 30 3 20 2 (fi
Page 143 and 144:
133 fig. 79
Page 145 and 146:
135 The difference between a whole
Page 147 and 148:
137 In either case, consonance is l
Page 149 and 150:
139 The interval between the notes
Page 151 and 152:
We can also write: MN ML 141 1 3 L
Page 153 and 154:
143 Ad Quadratum and the Just Inton
Page 155 and 156:
145 from nothing. It is indivisible
Page 157 and 158:
AA' OA1 2 1 BB' 2 = PP' OP 1 147
Page 159 and 160:
149 fig. 87
Page 161 and 162:
151
Page 163 and 164:
153 Ad Quadratum, the Millennium Sp
Page 165 and 166:
155 own positions in it. Kepler’s
Page 167 and 168:
157 The Millennium Sphere and the L
Page 169 and 170:
159 fig. 89 fig. 90
Page 171 and 172:
161 fig. 91
Page 173 and 174:
This determines the envelope of des
Page 175 and 176:
Remarks TABLE 1 TRIGONOMETRIC PROPE
Page 177 and 178:
TABLE 3 REGULAR CONVEX POLYHEDRA ME
Page 179 and 180:
R G r R G R S R S r 169 From r to
Page 181 and 182:
3. Octahedron: fig. 3Ap 4. Dodecahe
Page 183 and 184:
173 One can also reason that there
Page 185 and 186:
Replacing in (1) by (2) or, rearran
Page 187 and 188:
and from (6) we see that 177 But 3
Page 189 and 190:
179 Alternatively, from (8) we obta
Page 191 and 192:
181 fig. 56
Page 193 and 194:
183 and finally R a 4 Therefore a
show all

Ad Quadratum Construction and Study of the Regular Polyhedra

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?