Desargues' Brouillon Project and the Conics of ... - J.P. Hogendijk
Desargues' Brouillon Project and the Conics of ... - J.P. Hogendijk
Desargues' Brouillon Project and the Conics of ... - J.P. Hogendijk
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
10 Jan f! <strong>Hogendijk</strong><br />
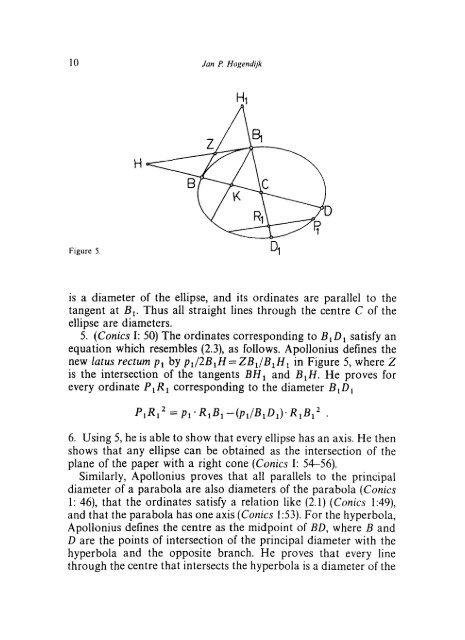
Figure 5.<br />
H<br />
is a diameter <strong>of</strong> <strong>the</strong> ellipse, <strong>and</strong> its ordinates are parallel to <strong>the</strong><br />
tangent at B,. Thus all straight lines through <strong>the</strong> centre C <strong>of</strong> <strong>the</strong><br />
ellipse are diameters.<br />
5. (<strong>Conics</strong> I: 50) The ordinates corresponding to BIDl satisfy an<br />
equation which resembles (2.3), as follows. Apollonius defines <strong>the</strong><br />
new l am rectum p1 by pl/2B1H=ZB,/B,H, in Figure 5, where Z<br />
is <strong>the</strong> intersection <strong>of</strong> <strong>the</strong> tangents BH1 <strong>and</strong> B,H. He proves for<br />
every ordinate PIR, corresponding to <strong>the</strong> diameter BIDl<br />
P1R12 = p1- RIB, -(pl/B,Dl)471B12 .<br />
6. Using 5, he is able to show that every ellipse has an axis. He <strong>the</strong>n<br />
shows that any ellipse can be obtained as <strong>the</strong> intersection <strong>of</strong> <strong>the</strong><br />
plane <strong>of</strong> <strong>the</strong> paper with a right cone (<strong>Conics</strong> I: 54-56).<br />
Similarly, Apollonius proves that all parallels to <strong>the</strong> principal<br />
diameter <strong>of</strong> a parabola are also diameters <strong>of</strong> <strong>the</strong> parabola (<strong>Conics</strong><br />
1: 46), that <strong>the</strong> ordinates satisfy a relation like (2.1) (<strong>Conics</strong> 1:49),<br />
<strong>and</strong> that <strong>the</strong> parabola has one axis (<strong>Conics</strong> 153). For <strong>the</strong> hyperbola,<br />
Apollonius defines <strong>the</strong> centre as <strong>the</strong> midpoint <strong>of</strong> BD, where B <strong>and</strong><br />
D are <strong>the</strong> points <strong>of</strong> intersection <strong>of</strong> <strong>the</strong> principal diameter with <strong>the</strong><br />
hyperbola <strong>and</strong> <strong>the</strong> opposite branch. He proves that every line<br />
through <strong>the</strong> centre that intersects <strong>the</strong> hyperbola is a diameter <strong>of</strong> <strong>the</strong>