Desargues' Brouillon Project and the Conics of ... - J.P. Hogendijk
Desargues' Brouillon Project and the Conics of ... - J.P. Hogendijk
Desargues' Brouillon Project and the Conics of ... - J.P. Hogendijk
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
6 Jan I? <strong>Hogendijk</strong><br />
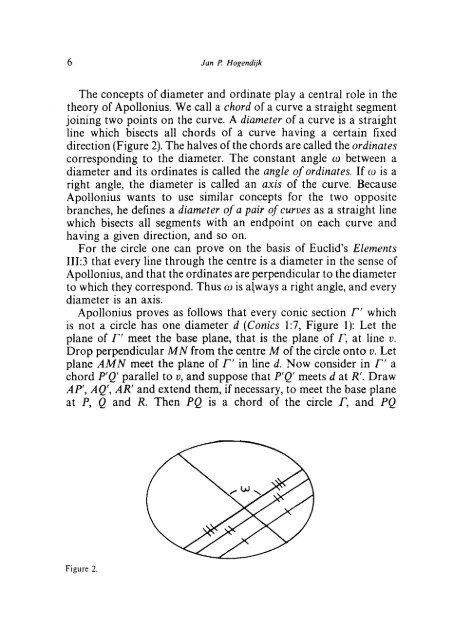
The concepts <strong>of</strong> diameter <strong>and</strong> ordinate play a central role in <strong>the</strong><br />
<strong>the</strong>ory <strong>of</strong> Apollonius. We call a chord <strong>of</strong> a curve a straight segment<br />
joining two points on <strong>the</strong> curve. A diameter <strong>of</strong> a curve is a straight<br />
line which bisects all chords <strong>of</strong> a curve having a certain fixed<br />
direction (Figure 2). The halves <strong>of</strong> <strong>the</strong> chords are called <strong>the</strong> ordinates<br />
corresponding to <strong>the</strong> diameter. The constant angle o between a<br />
diameter <strong>and</strong> its ordinates is called <strong>the</strong> angle <strong>of</strong>ordinates. If w is a<br />
right angle, <strong>the</strong> diameter is called an axis <strong>of</strong> <strong>the</strong> curve. Because<br />
Apollonius wants to use similar concepts for <strong>the</strong> two opposite<br />
branches, he defines a diameter <strong>of</strong>a pair <strong>of</strong>curues as a straight line<br />
which bisects all segments with an endpoint on each curve <strong>and</strong><br />
having a given direction, <strong>and</strong> so on.<br />
For <strong>the</strong> circle one can prove on <strong>the</strong> basis <strong>of</strong> Euclid’s Elements<br />
II1:3 that every line through <strong>the</strong> centre is a diameter in <strong>the</strong> sense <strong>of</strong><br />
Apollonius, <strong>and</strong> that <strong>the</strong> ordinates are perpendicular to <strong>the</strong> diameter<br />
to which <strong>the</strong>y correspond. Thus o is always a right angle, <strong>and</strong> every<br />
diameter is an axis.<br />
Apollonius proves as follows that every conic section r’ which<br />
is not a circle has one diameter d (<strong>Conics</strong> 1:7, Figure 1): Let <strong>the</strong><br />
plane <strong>of</strong> r’ meet <strong>the</strong> base plane, that is <strong>the</strong> plane <strong>of</strong> r, at line u.<br />
Drop perpendicular MN from <strong>the</strong> centre M <strong>of</strong> <strong>the</strong> circle onto u. Let<br />
plane AMN meet <strong>the</strong> plane <strong>of</strong> r’ in line d. Now consider in r‘ a<br />
chord P’Q’ parallel to u, <strong>and</strong> suppose that P’Q’ meets d at R‘. Draw<br />
AP‘, AQ’, AR’ <strong>and</strong> extend <strong>the</strong>m, if necessary, to meet <strong>the</strong> base plane<br />
at P, Q <strong>and</strong> R. Then PQ is a chord <strong>of</strong> <strong>the</strong> circle r, <strong>and</strong> PQ<br />
Figure 2.