- Page 1:
DOCTORAL THESIS IN PHOTONICS ICFO B
- Page 5:
Spatial Characterization Of Two-Pho
- Page 9 and 10:
Contents Contents vii Acknowledgeme
- Page 11 and 12:
Acknowledgements This thesis compil
- Page 13 and 14:
Abstract In the same way that elect
- Page 15 and 16:
Resumen De la misma manera que la e
- Page 17 and 18:
Introduction The role of photons in
- Page 19:
This thesis is based on the followi
- Page 22 and 23:
1. General description of two-photo
- Page 24 and 25:
1. General description of two-photo
- Page 26 and 27:
1. General description of two-photo
- Page 28 and 29:
1. General description of two-photo
- Page 30 and 31:
1. General description of two-photo
- Page 32 and 33:
1. General description of two-photo
- Page 35 and 36:
CHAPTER 2 Correlations and entangle
- Page 37 and 38:
2.1. The purity as a correlation in
- Page 39 and 40:
2.2. Correlations between space and
- Page 41 and 42:
2.2. Correlations between space and
- Page 43 and 44:
2.3. Correlations between signal an
- Page 45 and 46:
2.3. Correlations between signal an
- Page 47 and 48:
Signal purity 1 0 (a) 0.1 3 2.3. Co
- Page 49 and 50:
CHAPTER 3 Spatial correlations and
- Page 51 and 52:
3.2. OAM transfer in general SPDC c
- Page 53 and 54:
3.2. OAM transfer in general SPDC c
- Page 55:
Phase front 3.3. OAM transfer in co
- Page 58 and 59:
4. OAM transfer in noncollinear con
- Page 60 and 61:
4. OAM transfer in noncollinear con
- Page 62 and 63:
4. OAM transfer in noncollinear con
- Page 64 and 65:
4. OAM transfer in noncollinear con
- Page 66 and 67:
4. OAM transfer in noncollinear con
- Page 68 and 69:
4. OAM transfer in noncollinear con
- Page 70 and 71:
4. OAM transfer in noncollinear con
- Page 72 and 73:
5. Spatial correlations in Raman tr
- Page 74 and 75:
5. Spatial correlations in Raman tr
- Page 76 and 77:
5. Spatial correlations in Raman tr
- Page 78 and 79:
5. Spatial correlations in Raman tr
- Page 81 and 82:
CHAPTER 6 Summary This thesis chara
- Page 83 and 84:
APPENDIX A The matrix form of the m
- Page 85 and 86:
v =γ 2 L 2 Np sin ϕi − γ 2 L 2
- Page 87:
The matrix C is given by C = 1 ⎛
- Page 90 and 91:
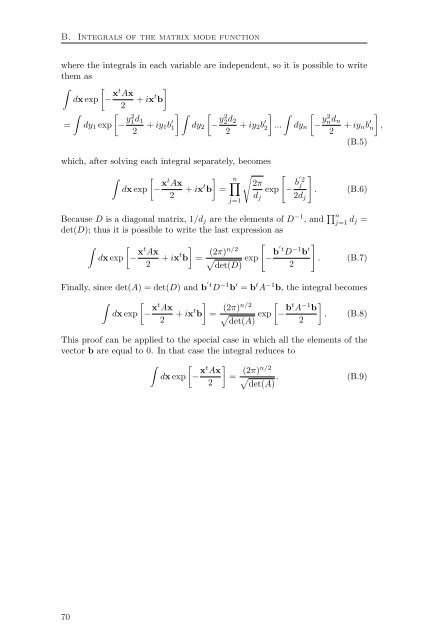
B. Integrals of the matrix mode fun
- Page 92 and 93:
C. Methods for OAM measurements Inp
- Page 94 and 95:
Bibliography [13] M. Barbieri, C. C
- Page 96 and 97:
Bibliography [41] C. I. Osorio, A.
- Page 98:
Bibliography [68] S. Chen, Y.-A. Ch
- Page 103:
Spatial Characterization Of Two-Pho
- Page 107:
A Luz Stella y Luis Alfonso, mis pa
- Page 110 and 111:
Contents B Integrals of the matrix
- Page 112 and 113:
When he [Kepler] found that his lon
- Page 114 and 115:
Abstract The matrix notation, intro
- Page 116 and 117:
Abstract La notación matricial int
- Page 118 and 119:
Introduction the transfer of oam fr
- Page 121 and 122:
CHAPTER 1 General description of Tw
- Page 123 and 124:
y y x z z pump s i (a) signal idle
- Page 125 and 126:
1.3. Approximations and other consi
- Page 127 and 128:
x 1.3. Approximations and other con
- Page 129 and 130:
x Wave fronts 1.3. Approximations a
- Page 131 and 132:
1 0 -0.2 1.3. Approximations and ot
- Page 133:
1.4. The mode function in matrix fo
- Page 136 and 137:
2. Correlations and entanglement (a
- Page 138 and 139:
2. Correlations and entanglement ch
- Page 140 and 141: 2. Correlations and entanglement th
- Page 142 and 143: 2. Correlations and entanglement Fi
- Page 144 and 145: 2. Correlations and entanglement q
- Page 146 and 147: 2. Correlations and entanglement Si
- Page 148 and 149: 2. Correlations and entanglement ri
- Page 150 and 151: 3. Spatial correlations and OAM tra
- Page 152 and 153: 3. Spatial correlations and OAM tra
- Page 154 and 155: 3. Spatial correlations and OAM tra
- Page 157 and 158: CHAPTER 4 OAM transfer in noncollin
- Page 159 and 160: 4.2. Effect of the pump beam waist
- Page 161 and 162: 1.0 0.0 4.2. Effect of the pump bea
- Page 163 and 164: Coincidences 800 0 -4 4.2. Effect o
- Page 165 and 166: Weight 1 0 4.3. Effect of the Poynt
- Page 167 and 168: 4.3. Effect of the Poynting vector
- Page 169 and 170: Before the crystal 4.3. Effect of t
- Page 171 and 172: CHAPTER 5 Spatial correlations in R
- Page 173 and 174: x y z 5.1. The quantum state of Sto
- Page 175 and 176: where 5.2. Orbital angular momentum
- Page 177 and 178: weight 1 0.6 -180º -90º 0º 90º
- Page 179: 5.3. Spatial entanglement 5.20 is s
- Page 182 and 183: 6. Summary Experiments that explain
- Page 184 and 185: A. The matrix form of the mode func
- Page 186 and 187: A. The matrix form of the mode func
- Page 189: APPENDIX B Integrals of the matrix
- Page 193 and 194: Bibliography [1] M. A. Nielsen and
- Page 195 and 196: Bibliography [27] J. P. Torres, A.
- Page 197 and 198: Bibliography [55] M. C. Booth, M. A