The Picard-Lefschetz theory of complexified Morse functions 1 ...

14 Joe Johns
Lagrangian embedding N ⊂ E. Consequently there is an exact Weinstein embedding
D(T ∗ N) ⊂ E. Now, let L ⊂ T ∗ N be any closed exact Lagrangian submanifold. By
rescaling L ǫL by some small ǫ > 0, we get an exact Lagrangian embedding L ⊂ E.
Now that we know L ⊂ E we can invoke Seidel’s decomposition theorem (∗). Roughly,
it says that we can represent L algebraically (at the level of Floer theory) in terms of
the Lefschetz thimbles ∆4,∆2,∆0 of π. To make this more explicit we need to know
how the Lefschetz thimbles interact Floer theoretically. That is, we need to know the
Floer homology groups
(1)
HF(∆4,∆2), HF(∆2,∆0), HF(∆4,∆0),
and also the triangle product (which is defined by counting holomorphic triangles with
boundary on ∆4,∆2,∆0):
(2)
HF(∆4,∆2) ⊗ HF(∆2,∆0) −→ HF(∆4,∆0).
These are precisely the calculations carried out in [J08], except we actually consider
the vanishing spheres Li = ∂∆i ⊂ M, i = 0, 2, 4 and do the corresponding equivalent
calculations in the regular fiber M. (In general, one does not expect to compute things
like (1) and (2) explicitly. It is only because of the very explicit and symmetrical nature
of M and L0, L2, L4 that the calculations in [J08] can be carried out.)
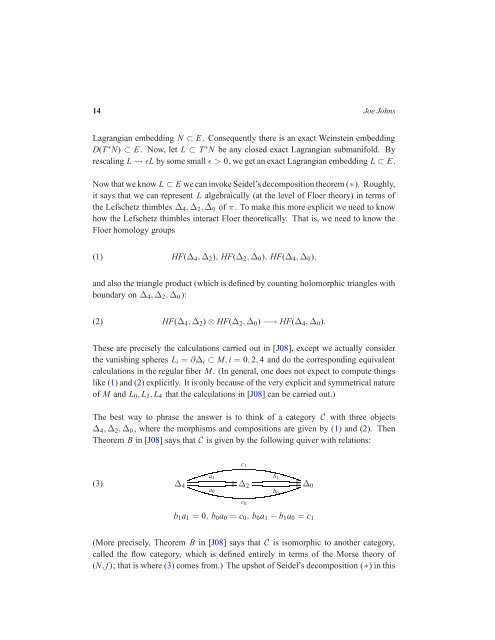
The best way to phrase the answer is to think of a category C with three objects
∆4,∆2,∆0 , where the morphisms and compositions are given by (1) and (2). Then
Theorem B in [J08] says that C is given by the following quiver with relations:
(3)
∆4
a1
a0
c1
∆2
c0
b1
b0
∆0
b1a1 = 0, b0a0 = c0, b0a1 − b1a0 = c1
(More precisely, Theorem B in [J08] says that C is isomorphic to another category,
called the flow category, which is defined entirely in terms of the Morse theory of
(N, f ); that is where (3) comes from.) The upshot of Seidel’s decomposition (∗) in this