Torsion
Torsion
Torsion
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
136 CHAPTER 5. TORSION<br />
The warping function, Ψ(x2, x3), which yields a stress field satisfying all equilibrium requirements is the<br />
solution of the following partial differential equation and associated boundary condition<br />
∂Ψ<br />
∂x2<br />
− x3<br />
∂2Ψ ∂x2 +<br />
2<br />
∂2Ψ ∂x2 = 0; on A<br />
3<br />
dx3<br />
ds −<br />
. (5.63)<br />
∂Ψ dx2<br />
+ x2 = 0. along C<br />
∂x3 ds<br />
The solution of this problem is rather complicated in view of the complex boundary condition that must<br />
hold along C.<br />
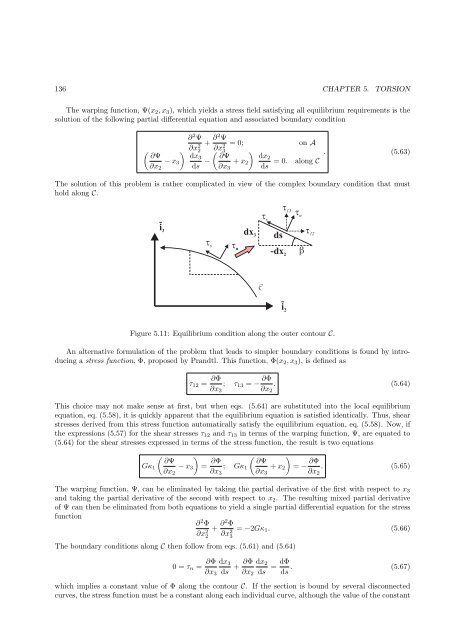
i 3<br />
s<br />
n<br />
dx 3 ds<br />
-dx 2<br />
Figure 5.11: Equilibrium condition along the outer contour C.<br />
An alternative formulation of the problem that leads to simpler boundary conditions is found by introducing<br />
a stress function, Φ, proposed by Prandtl. This function, Φ(x2, x3), is defined as<br />
C<br />
s<br />
13<br />
i 2<br />
n<br />
<br />
τ12 = ∂Φ<br />
; τ13 = −<br />
∂x3<br />
∂Φ<br />
. (5.64)<br />
∂x2<br />
This choice may not make sense at first, but when eqs. (5.64) are substituted into the local equilibrium<br />
equation, eq. (5.58), it is quickly apparent that the equilibrium equation is satisfied identically. Thus, shear<br />
stresses derived from this stress function automatically satisfy the equilibrium equation, eq. (5.58). Now, if<br />
the expressions (5.57) for the shear stresses τ12 and τ13 in terms of the warping function, Ψ, are equated to<br />
(5.64) for the shear stresses expressed in terms of the stress function, the result is two equations<br />
Gκ1<br />
∂Ψ<br />
∂x2<br />
− x3<br />
<br />
12<br />
= ∂Φ<br />
<br />
∂Ψ<br />
; Gκ1 + x2 = −<br />
∂x3 ∂x3<br />
∂Φ<br />
. (5.65)<br />
∂x2<br />
The warping function, Ψ, can be eliminated by taking the partial derivative of the first with respect to x3<br />
and taking the partial derivative of the second with respect to x2. The resulting mixed partial derivative<br />
of Ψ can then be eliminated from both equations to yield a single partial differential equation for the stress<br />
function<br />
∂ 2 Φ<br />
∂x 2 2<br />
+ ∂2 Φ<br />
∂x 2 3<br />
The boundary conditions along C then follow from eqs. (5.61) and (5.64)<br />
0 = τn = ∂Φ<br />
∂x3<br />
dx3<br />
ds<br />
= −2Gκ1. (5.66)<br />
∂Φ dx2<br />
+<br />
∂x2 ds<br />
dΦ<br />
= . (5.67)<br />
ds<br />
which implies a constant value of Φ along the contour C. If the section is bound by several disconnected<br />
curves, the stress function must be a constant along each individual curve, although the value of the constant