Torsion

142 CHAPTER 5. TORSION
According to the principle of minimum total potential energy, the deformation that corresponds to the
equilibrium configuration of the system will make the total potential energy a minimum. In this case, the
deformation is the assumed warping, Ψ, but since this is directly related to the stress function, Φ, we can
choose approximations for either. Following the general procedure described in section 3.6.10, approximate
solutions to the torsional problem can be obtained by starting from an assumed structure of the stress
function, then minimizing the total potential energy. This procedure will be demonstrated in the examples
below.
The expression of the total potential energy given by eq. (5.82) is valid for solid cross-sections bounded
by a single curve. For such sections, the assumed stress function should be chosen with Φ = 0 along C.
For sections bound by several disconnected curves, the applied torque should be evaluated with the help of
eq. (5.71), and the stress function equals a different constant along each individual curve.
5.3.4 Examples
Example 5.3: Torsion of rectangular section - a crude solution
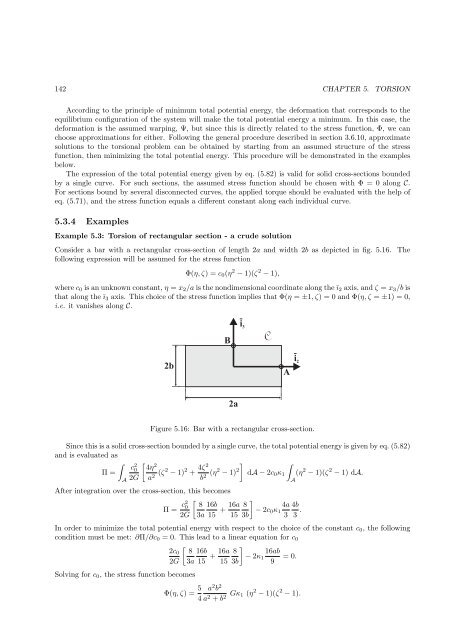
Consider a bar with a rectangular cross-section of length 2a and width 2b as depicted in fig. 5.16. The
following expression will be assumed for the stress function
Φ(η, ζ) = c0(η 2 − 1)(ζ 2 − 1),
where c0 is an unknown constant, η = x2/a is the nondimensional coordinate along the ī2 axis, and ζ = x3/b is
that along the ī3 axis. This choice of the stress function implies that Φ(η = ±1, ζ) = 0 and Φ(η, ζ = ±1) = 0,
i.e. it vanishes along C.
2b
B
2a
Figure 5.16: Bar with a rectangular cross-section.
Since this is a solid cross-section bounded by a single curve, the total potential energy is given by eq. (5.82)
and is evaluated as
Π =
c2 2
0 4η
2G a2 (ζ2 − 1) 2 + 4ζ2
b2 (η2 − 1) 2
dA − 2c0κ1 (η 2 − 1)(ζ 2 − 1) dA.
A
After integration over the cross-section, this becomes
Π = c2 0
2G
8 16b
3a 15
+ 16a
15
i 3
C
A
A
i 2
8 4a 4b
− 2c0κ1
3b 3 3 .
In order to minimize the total potential energy with respect to the choice of the constant c0, the following
condition must be met: ∂Π/∂c0 = 0. This lead to a linear equation for c0
2c0 8 16b 16a 8 16ab
+ − 2κ1 = 0.
2G 3a 15 15 3b 9
Solving for c0, the stress function becomes
a 2 b 2
Φ(η, ζ) = 5
4 a2 + b2 Gκ1 (η 2 − 1)(ζ 2 − 1).