Torsion

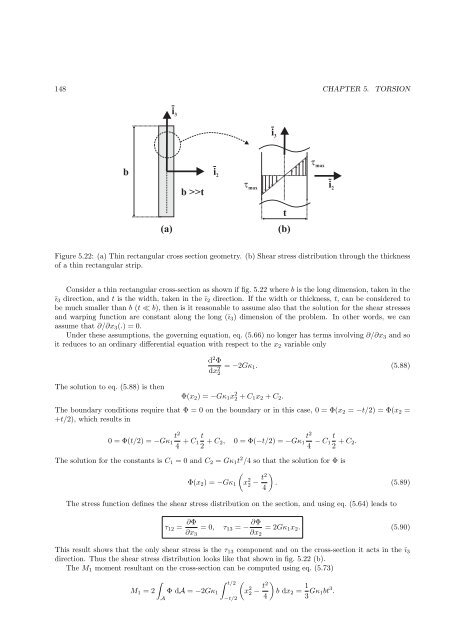
148 CHAPTER 5. TORSION
b
i 3
b >>t
i 2
max
(a) (b)
Figure 5.22: (a) Thin rectangular cross section geometry. (b) Shear stress distribution through the thickness
of a thin rectangular strip.
Consider a thin rectangular cross-section as shown if fig. 5.22 where b is the long dimension, taken in the
ī3 direction, and t is the width, taken in the ī2 direction. If the width or thickness, t, can be considered to
be much smaller than b (t ≪ b), then is it reasonable to assume also that the solution for the shear stresses
and warping function are constant along the long (ī3) dimension of the problem. In other words, we can
assume that ∂/∂x3(.) = 0.
Under these assumptions, the governing equation, eq. (5.66) no longer has terms involving ∂/∂x3 and so
it reduces to an ordinary differential equation with respect to the x2 variable only
The solution to eq. (5.88) is then
d 2 Φ
dx 2 2
i 3
t
max
= −2Gκ1. (5.88)
Φ(x2) = −Gκ1x 2 2 + C1x2 + C2.
The boundary conditions require that Φ = 0 on the boundary or in this case, 0 = Φ(x2 = −t/2) = Φ(x2 =
+t/2), which results in
t
0 = Φ(t/2) = −Gκ1
2
4
t
+ C1
2 + C2,
t
0 = Φ(−t/2) = −Gκ1
2
4
i 2
t
− C1 + C2.
2
The solution for the constants is C1 = 0 and C2 = Gκ1t 2 /4 so that the solution for Φ is
Φ(x2) = −Gκ1
x 2 2 − t2
. (5.89)
4
The stress function defines the shear stress distribution on the section, and using eq. (5.64) leads to
τ12 = ∂Φ
= 0, τ13 = −
∂x3
∂Φ
= 2Gκ1x2. (5.90)
∂x2
This result shows that the only shear stress is the τ13 component and on the cross-section it acts in the ī3
direction. Thus the shear stress distribution looks like that shown in fig. 5.22 (b).
The M1 moment resultant on the cross-section can be computed using eq. (5.73)
t/2
M1 = 2 Φ dA = −2Gκ1 x
A
−t/2
2 2 − t2
b dx2 =
4
1
3 Gκ1bt 3 .