Chapter One: Vector Analysis The use of vectors and vector analysis ...
Chapter One: Vector Analysis The use of vectors and vector analysis ...
Chapter One: Vector Analysis The use of vectors and vector analysis ...
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<strong>Chapter</strong> <strong>One</strong>: <strong>Vector</strong> <strong>Analysis</strong><br />
<strong>The</strong> <strong>use</strong> <strong>of</strong> <strong><strong>vector</strong>s</strong> <strong>and</strong> <strong>vector</strong> <strong>analysis</strong> can greatly simplify the mathematics <strong>use</strong>d in<br />
expressing <strong>and</strong> manipulating the laws <strong>and</strong> theorems <strong>of</strong> electric <strong>and</strong> magnetic fields.<br />
Scalars <strong>and</strong> <strong>Vector</strong>s:<br />
Scalar is a quantity that has only magnitude, such as: time, distance, temperature, entropy,<br />
energy, mass, electric potential ………<br />
<strong>The</strong> <strong>vector</strong> is a quantity that has both magnitude <strong>and</strong> direction, such as: velocity, force,<br />
displacement, electric <strong>and</strong> magnetic field intensity …………<br />
<strong>Vector</strong> Operations<br />
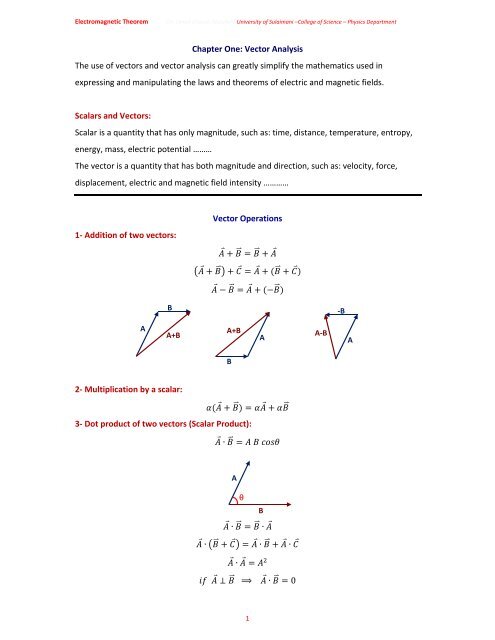
1‐ Addition <strong>of</strong> two <strong><strong>vector</strong>s</strong>:<br />
<br />
<br />
<br />
B<br />
‐B<br />
A<br />
A+B<br />
A+B<br />
A<br />
A‐B<br />
A<br />
B<br />
2‐ Multiplication by a scalar:<br />
<br />
3‐ Dot product <strong>of</strong> two <strong><strong>vector</strong>s</strong> (Scalar Product):<br />
∙ <br />
A<br />
<br />
B<br />
∙ ∙<br />
∙ ∙ ∙<br />
∙ <br />
⟹ ∙ 0<br />
1
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Example:<br />
Let , calculate the dot product <strong>of</strong> with itself:<br />
∙ ∙ ∙ ∙ ∙ ∙<br />
2<br />
This is the law <strong>of</strong> cosine.<br />
4‐ Cross product <strong>of</strong> two <strong><strong>vector</strong>s</strong> (<strong>Vector</strong> Product):<br />
<br />
where is a unit <strong>vector</strong>, pointing perpendicular to the plane <strong>of</strong> <strong>and</strong> . (the direction<br />
pointed by right‐h<strong>and</strong> rule).<br />
Right‐h<strong>and</strong> rule: Let your finger point in the direction <strong>of</strong> the first <strong>vector</strong> <strong>and</strong> curl around (via<br />
the smaller angle) toward the second; then your thumb indicates the direction <strong>of</strong> .<br />
<br />
<br />
0<br />
<strong>Vector</strong> Algebra:<br />
Let , , <strong>and</strong> be unit <strong><strong>vector</strong>s</strong> parallel to the , , <strong>and</strong> respectively.<br />
An arbitrary <strong>vector</strong> can be exp<strong>and</strong>ed in term <strong>of</strong> these basis <strong><strong>vector</strong>s</strong>:<br />
<br />
<strong>The</strong> numbers , , <strong>and</strong> are called components <strong>of</strong> .<br />
<br />
<br />
∙ ∙ <br />
∙ <br />
∙ ∙ ∙ 1<br />
2
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
∙ ∙ ∙ 0<br />
∙ <br />
<br />
|| <br />
<br />
∙ ; ∙ ; ∙ <br />
In the case <strong>of</strong> cross product:<br />
<strong>The</strong>refore:<br />
0<br />
<br />
<br />
<br />
<br />
<br />
This cumbersome expression can be written as a determinant:<br />
<br />
<br />
<br />
<br />
<br />
<br />
Where is perpendicular to both <strong>and</strong> .<br />
Prove <strong>of</strong> dot product rule:<br />
<br />
, <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
, <br />
<br />
<br />
<br />
3
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
∙ ∙ <br />
cos cos <br />
cos cos sin sin <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
∴ ∙ cos<br />
Prove <strong>of</strong> cross product rule:<br />
<br />
<br />
<br />
<br />
2 2 <br />
<br />
2 <br />
<br />
<br />
2 2 2 <br />
2 <br />
2 2 <br />
∙ cos 1cos sin <br />
∴ sin<br />
<strong>Vector</strong> Directions:<br />
<strong>The</strong> position <strong>vector</strong> or radius <strong>vector</strong> <strong>of</strong> point , , is defined as the directed distance<br />
from the origin 0,0,0 to point , , as:<br />
<br />
<strong>The</strong> distance <strong>vector</strong> is the displacement from one point to another, such as, , , to<br />
, , <strong>and</strong> is represented mathematically as:<br />
<br />
<strong>The</strong>refore, the position <strong>vector</strong> is a special case <strong>of</strong> displacement <strong>vector</strong>.<br />
4
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Example:<br />
Find the angle between the face diagonals <strong>of</strong> a cube.<br />
Let:<br />
z<br />
(0,0,1)<br />
<br />
x<br />
<br />
<br />
(1,0,0)<br />
(0,1,0)<br />
y<br />
1 0 1 <br />
0 1 1 <br />
∙ 0011<br />
|| √2 ; || √2<br />
∙ ⟹ ∙<br />
<br />
1 2<br />
⟹ 60 °<br />
Problem:<br />
Find the angle between the body diagonals <strong>of</strong> a cube.<br />
Let:<br />
z<br />
(0,0,1)<br />
<br />
x<br />
(1,0,0)<br />
<br />
<br />
(0,1,0)<br />
y<br />
5
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
1 1 1 <br />
1 1 1 <br />
∙ 1111<br />
|| √3 ; || √3<br />
∙ ⟹ ∙<br />
<br />
1 3<br />
⟹ 70.5288 °<br />
Problem:<br />
Use the cross product to find the components <strong>of</strong> the <strong>vector</strong> perpendicular to the plane<br />
shown in Fig.<br />
z<br />
3<br />
<br />
x<br />
1<br />
2<br />
y<br />
Let:<br />
1 2 0 <br />
1 0 3 <br />
<br />
1 2 0 6 3 2 <br />
1 0 3<br />
6 3 2 7<br />
<br />
<br />
Triple Products:<br />
Since the cross product <strong>of</strong> two <strong><strong>vector</strong>s</strong> is itself a <strong>vector</strong>, it can be dotted or crossed with a<br />
third <strong>vector</strong> to form a triple product.<br />
1‐ Scalar triple product: ∙ <br />
Geometrically ∙ is the volume <strong>of</strong> the parallelepiped generated by , ,<br />
<br />
6
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
∙ ∙ ∙ <br />
<br />
∙ <br />
<br />
2‐ <strong>Vector</strong> triple product: <br />
∙ ∙<br />
∙ ∙ ∙ ∙ <br />
∙ ∙ <br />
Prove: ∙ ∙<br />
<br />
<br />
<br />
<br />
<br />
∙ ∙<br />
<br />
<br />
<br />
<br />
∴ Left h<strong>and</strong> = right h<strong>and</strong>.<br />
<br />
Problem:<br />
Prove that: 0<br />
Since; ∙ ∙<br />
<br />
∙ ∙ ∙ ∙ ∙ ∙ 0<br />
Problem:<br />
Under what conditions dose <br />
7
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
We have<br />
∙ ∙ ∙ ∙<br />
∙ ∙<br />
If this is zero; then either is parallel to (including the case in which they point in<br />
opposite directions, or one is zero), or else ∙ ∙ 0 in which case is<br />
perpendicular to <strong>and</strong> (including the case 0).<br />
Concluding: either:<br />
1‐ is parallel to , or<br />
2‐ is perpendicular to <strong>and</strong> .<br />
Position Displacement:<br />
<strong>The</strong> location <strong>of</strong> a point in three dimensions can be described by listing its Cartesian<br />
coordinates , , . <strong>The</strong> <strong>vector</strong> to that point form the origin is called the position <strong>vector</strong>;<br />
Its magnitude:<br />
<strong>The</strong> unit <strong>vector</strong> pointing radially outword, is<br />
<br />
|| <br />
̂ <br />
|| <br />
<br />
z<br />
<br />
<br />
, , <br />
<br />
y<br />
x<br />
<br />
<br />
<strong>The</strong> infinitesimal displacement <strong>vector</strong>, from , , to , , is:<br />
<br />
<strong>The</strong> infinitesimal surface <strong>of</strong> Cartesian Coordinate is:<br />
<br />
8
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<strong>Vector</strong> Transformation:<br />
Suppose, the , , system is rotated by angle , relative to , , , about the<br />
common ′.<br />
cos<br />
sin<br />
z'<br />
z<br />
While;<br />
<br />
<br />
y'<br />
′<br />
<br />
<br />
cos cos cos cos sin sin cos sin <br />
sin sin sin cos cos sin sin cos <br />
In matrix notation;<br />
′<br />
′ <br />
<br />
<br />
<br />
More generally, for rotation about an arbitrary axis in three dimensions:<br />
′ <br />
′ <br />
′ <br />
Tenser <strong>of</strong> rank 2<br />
In general an n th ‐rank tensor has 3 n components.<br />
y<br />
9
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Gradient:<br />
Suppose, we have a function <strong>of</strong> three variables, say, the temperature ,, in a room.<br />
A theorem on partial derivative state that the derivative <strong>of</strong> can be calculated from:<br />
<br />
<br />
This tells us how changes when alter all three variables by the infinitesimal amount , <br />
, ; Above equation in a dot products<br />
Where;<br />
Is the gradient <strong>of</strong> .<br />
<br />
<br />
<br />
∙ ∙ <br />
is a <strong>vector</strong> quantity.<br />
<br />
<br />
<br />
<br />
<strong>The</strong> gradient points in the direction <strong>of</strong> maximum increase <strong>of</strong> the function .<br />
<strong>The</strong> magnitude gives the slop (rate <strong>of</strong> increase) along this maximal direction.<br />
If gradient vanish 0 at , , . this is, then, a stationary point <strong>of</strong> the function<br />
, , . It could be a maximum or a minimum points.<br />
<br />
is called "Del" operator.<br />
Del is not a <strong>vector</strong>, it is without specific meaning until we provide it with a function to act<br />
upon.<br />
Example:<br />
Find the gradient <strong>of</strong> <br />
<br />
<br />
<br />
<br />
2<br />
<br />
2<br />
<br />
2<br />
<br />
<br />
<br />
<br />
<br />
|| ̂<br />
10
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<strong>The</strong> Divergence:<br />
Del operator act on a <strong>vector</strong> function , via the dot product.<br />
∙V ∙ <br />
<strong>The</strong> result is scalar.<br />
∙V <br />
<br />
<br />
<br />
<strong>The</strong> divergence is measure, how much the <strong>vector</strong> spreads out.<br />
Positive Divergence<br />
Zero Divergence<br />
Positive Divergence<br />
Example:<br />
Calculate the divergence <strong>of</strong> the following <strong>vector</strong> functions:<br />
a/ <br />
∙ 1113<br />
<br />
b/ 2 2 <br />
∙ 2 2 0222<br />
<br />
Problem:<br />
Compute the divergence <strong>of</strong> ̂<br />
<br />
∙ <br />
∙ / / / <br />
11
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
∙<br />
/ 2 <br />
/ <br />
2 <br />
/ <br />
2 /<br />
∙ 3 3 <br />
∙3<br />
3 0<br />
<br />
/ <br />
/ <br />
/ <br />
<strong>The</strong> Curl:<br />
Del operator can act on a <strong>vector</strong> function , via the crosss product:<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<strong>The</strong> curl measure, how much the <strong>vector</strong> curl around the point in question.<br />
Two<br />
figures have a substantial curl.<br />
Example:<br />
Calculate<br />
the curl <strong>of</strong> functions:<br />
<br />
<br />
<br />
<br />
2 <br />
<br />
<br />
0<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
0 0
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Problem:<br />
Calculate the curls <strong>of</strong> functions:<br />
3 2 <br />
<br />
<br />
<br />
6 2 3 <br />
<br />
3 2<br />
2 3 <br />
<br />
<br />
<br />
2 3 <br />
<br />
2 3<br />
2 2 <br />
<br />
<br />
<br />
0<br />
<br />
2 2<br />
Product Rules:<br />
<strong>The</strong> calculation <strong>of</strong> ordinary derivatives is facilitated by a number <strong>of</strong> general rules, such as:<br />
<strong>The</strong> sum rule:<br />
<br />
<br />
<br />
<br />
<strong>The</strong> rule for multiplying by a constant:<br />
<br />
<br />
<br />
<strong>The</strong> product rule:<br />
<br />
<br />
<br />
And, the quotient rule:<br />
<br />
<br />
<br />
<strong>The</strong>re are six product rules for Del operator:<br />
Two for gradients:<br />
<br />
<br />
<br />
<br />
<br />
13
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
∙ ∙ ∙<br />
Two for divergences:<br />
∙ ∙ ∙<br />
∙ ∙ ∙ <br />
<strong>and</strong>, two for curls:<br />
<br />
∙ ∙ ∙ ∙<br />
<strong>The</strong> pro<strong>of</strong>s <strong>of</strong> these rules wanted.<br />
<strong>The</strong> pro<strong>of</strong>s come straight from the product rule for ordinary derivatives.<br />
It is also possible to formulate three quotients rule:<br />
<br />
<br />
<br />
∙ <br />
∙ ∙<br />
<br />
<br />
<br />
<br />
<strong>The</strong> pro<strong>of</strong>s <strong>of</strong> these rules wanted.<br />
Problems:<br />
Prove product rules:<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
Problems:<br />
Prove product rules:<br />
∙ ∙ ∙<br />
14
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
∙ <br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
∙ <br />
<br />
<br />
∙ <br />
<br />
<br />
<br />
∙ ∙ ∙<br />
Problems:<br />
Prove product rules:<br />
∙ ∙ ∙ <br />
∙ <br />
<br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
∙ <br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
∙ ∙ ∙ <br />
Problems:<br />
Prove product rules:<br />
<br />
15
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
Problem: Compute ̂ ∙̂ where ̂ is the position unit <strong>vector</strong>.<br />
̂ <br />
|| <br />
<br />
Let’s just do the component:<br />
1<br />
̂ ∙̂ <br />
<br />
<br />
<br />
̂ ∙̂ <br />
<br />
1<br />
1<br />
1<br />
<br />
2<br />
1<br />
<br />
2 1<br />
<br />
2 <br />
̂ ∙̂ <br />
<br />
1<br />
1<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
̂ ∙̂ <br />
1 1 <br />
0<br />
Same goes for the other components: Hence; ̂ ∙̂ 0<br />
16
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Problem:<br />
Let 2 3 ,<br />
3 2 <br />
Check product rules:<br />
1‐ ∙ ∙ ∙ <br />
2‐ ∙ ∙ ∙<br />
3‐ ∙ ∙ ∙ ∙<br />
Pro<strong>of</strong> <strong>of</strong> (1):<br />
<br />
2 36 9 2 6 <br />
3 2 0<br />
∙ 6 9 2 6 <br />
∙ 6 9 0 15<br />
3 2 3 2 0<br />
∴B ∙ 0<br />
0 2 3 0 2 3 <br />
5 <br />
∴A ∙ 15<br />
∴ ∙ ∙ 015 15<br />
∴ ∙ ∙ ∙ <br />
Second Derivatives:<br />
<strong>The</strong> gradient, the divergence, <strong>and</strong> curl are the only first derivative we can make with ; by<br />
applying twice we can construct five species <strong>of</strong> second derivatives,<br />
<strong>The</strong> gradient is a <strong>vector</strong>, so we can take the divergence <strong>and</strong> curl <strong>of</strong> it:<br />
1‐ Divergence <strong>of</strong> gradient: ∙<br />
2‐ Curl <strong>of</strong> gradient: <br />
<strong>The</strong> divergence ∙ is a scalar, all we can do is take its gradient:<br />
3‐ Gradient <strong>of</strong> divergence: ∙<br />
17
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<strong>The</strong> curl is a <strong>vector</strong>, so we can take its divergence <strong>and</strong> curl:<br />
4‐ Divergence <strong>of</strong> curl: ∙ <br />
5‐ Curl <strong>of</strong> Curl: <br />
Let’s consider them one at a time:<br />
1)<br />
∙ ∙ <br />
<br />
<br />
<br />
∙ <br />
<br />
<br />
<br />
This object, which we write is called the Laplacian <strong>of</strong> .<br />
Note that the Laplacian <strong>of</strong> a scalar is a scalar. Occasionally, we shall speak <strong>of</strong> the Laplacian<br />
<strong>of</strong> a <strong>vector</strong>, .<br />
<br />
2)<br />
<strong>The</strong> curl <strong>of</strong> a gradient is always zero:<br />
0<br />
This is an important fact, which we shall <strong>use</strong> repeatedly.<br />
3)<br />
∙ for some reason seldom occurs in physical applications.<br />
Notice that ∙ is not the same as the Laplace <strong>of</strong> <strong>vector</strong>:<br />
∙ ∙<br />
4)<br />
<strong>The</strong> Divergence <strong>of</strong> curl ∙ , like the curl <strong>of</strong> gradient, is always zero:<br />
∙ 0<br />
5)<br />
From definition <strong>of</strong> <br />
∙ <br />
We could go through a similar ritual to work out third derivative, but fortunately second<br />
derivative suffice for practically all physical applications.<br />
18
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Problem:<br />
Calculate the Laplacian <strong>of</strong> the following functions:<br />
a) 234<br />
b) sin sin sin <br />
c) sin 4 cos 3<br />
d) 3 2 <br />
a))<br />
b))<br />
<br />
2 ; <br />
<br />
0 ⟹ 2<br />
<br />
<br />
<br />
sin sin sin ⟹ 3 sin sin sin 3 <br />
c))<br />
d))<br />
<br />
25 ;<br />
<br />
16 ;<br />
<br />
9 ⟹ 0<br />
<br />
2 ; <br />
<br />
0 ⟹ 2<br />
<br />
<br />
0 ; <br />
6 ⟹ 6<br />
<br />
<br />
<br />
0 ⟹ 0<br />
2 6 <br />
Problem:<br />
Prove that the divergence <strong>of</strong> a curl is always zero.<br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
By equality <strong>of</strong> cross‐derivative:<br />
∙ 0<br />
19
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Problem:<br />
Prove that the curl <strong>of</strong> gradient is always zero.<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
By equality <strong>of</strong> cross‐derivative:<br />
0<br />
20
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Integral Calculus:<br />
1‐ Line integrals (Path):<br />
A line integral is an expression <strong>of</strong><br />
the form,<br />
∙ <br />
where is a <strong>vector</strong> function, <br />
is the infinitesimal displacement <strong>vector</strong>.<br />
And the integral is to<br />
be carried out along a prescribed path from point to point .<br />
<br />
<br />
If the path in question forms a closed loop (that is if ), I shall put a circle on the integral<br />
sign:<br />
∙ <br />
Example <strong>of</strong> line integrals is the work done by<br />
a force :<br />
∙ <br />
Ordinarily, the value <strong>of</strong> a line integral depends critically on the particular path taken from <br />
to .<br />
But if the<br />
line integral is independent <strong>of</strong> the path; a force<br />
that has this property is called<br />
Conservative.<br />
Example:<br />
Calculate<br />
the line integral <strong>of</strong> the function 2 1 from the point<br />
1 ,1<br />
, 0 to the point 2 , 2 , 0 along the paths 1 & 2 in Fig. What is<br />
∮ ∙ <br />
for the loop<br />
that goes from to along 1 <strong>and</strong> returns to along 2?
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<br />
∙ 21 ∙ <br />
Path 1 consists <strong>of</strong> two paths:<br />
(i) along the horizontal segment<br />
∙ 21 <br />
0 ; ; 1<br />
∙ ⟹ ∙ <br />
(ii) along the vertical segment<br />
0 ; ; 2<br />
∙ 21 41 ⟹ ∙ 4 1<br />
∴ Path 1<br />
∙ <br />
<br />
<br />
11011<br />
<br />
<br />
<br />
<br />
1<br />
10<br />
On path 2:<br />
; ; 0 ; <br />
∙ 2 1 3 2<br />
<br />
∙ <br />
<br />
<br />
3 2 2 1 10<br />
<br />
∙ 11101<br />
2‐ Surface integrals (Flux):<br />
A surface integral is an expression <strong>of</strong> the form:<br />
∙ <br />
<br />
where is again some <strong>vector</strong> function, is an infinitesimal path <strong>of</strong> area, which direction<br />
perpendicular to the surface.<br />
22
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
If the surface is closed, in which case I shall again put a circle on the integral signs:<br />
<br />
∙ <br />
Example:<br />
Calculate<br />
the surface<br />
integral <strong>of</strong><br />
2 2 3 <br />
over five sides<br />
(excluding the bottom) <strong>of</strong> the cubical box ( side 2 ) in Fig.<br />
(i)<br />
2 ;<br />
<br />
⟹<br />
∙ 2 4 <br />
<br />
<br />
(ii)<br />
∙ 4<br />
<br />
<br />
16<br />
<br />
0 ; <br />
⟹<br />
∙ 2<br />
0<br />
<br />
∙ 0<br />
(iii)<br />
2<br />
; <br />
⟹<br />
∙ <br />
2 <br />
<br />
<br />
∙ <br />
2 12<br />
<br />
<br />
(iv)<br />
0<br />
; <br />
⟹<br />
∙ 2 <br />
<br />
<br />
∙ <br />
2 12<br />
<br />
<br />
(v)<br />
2 ; <br />
<br />
<br />
⟹<br />
∙ 3
Electromagnetic <strong>The</strong>orem (Dr. Omed G<br />
Department<br />
<br />
Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics D<br />
∙ <br />
4<br />
<br />
Evidently<br />
the total flux is:<br />
∙ 160<br />
12122 420<br />
3‐ Volume integrals:<br />
A volume<br />
integral is an expression <strong>of</strong> the form:<br />
<br />
<br />
where is a scalar function <strong>and</strong> is an infinitesimal volume element:<br />
in Cartesian coordinates:<br />
<br />
Occasionally we shall encounter volume integrals <strong>of</strong> <strong>vector</strong> functions:<br />
<br />
<br />
beca<strong>use</strong> the unit <strong><strong>vector</strong>s</strong> are constants, they<br />
come outside the integral.<br />
Example:<br />
Calculate<br />
the volume<br />
integral <strong>of</strong> <br />
over the prism in Fig.<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
1 <br />
2 <br />
<br />
<br />
<br />
3 3 0 2 2 <br />
3 <br />
4 1 0 9 1 12 3 8
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Example:<br />
Calculate the volume integral <strong>of</strong> over the volume in Fig.<br />
z<br />
(0,0,1)<br />
<br />
y<br />
(0,2,0)<br />
x<br />
(3,0,0)<br />
<br />
3 2 <br />
3 <br />
<br />
3 2 0 <br />
3 0 1<br />
20 03 06 <br />
2 3 6 <br />
Using equation <strong>of</strong> normal on the surface , <strong>and</strong> the point 3 , 0 , 0<br />
the surface equation can be found from the equation:<br />
0<br />
∴ 2 3 3 0 6 0 0<br />
2 3 6 6 0<br />
⟹ 3 3 <br />
To find the relation between <strong>and</strong> ; we <strong>use</strong> the two points<br />
<br />
<br />
<br />
<br />
⟹<br />
, ⟹ 2 , 0<br />
, ⟹ 0 , 1<br />
2<br />
0 02<br />
10<br />
⟹<br />
2 2 ⟹ 2 2 <br />
2<br />
<br />
2<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
2 <br />
<br />
<br />
<br />
25
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<br />
<br />
<br />
<br />
<br />
<br />
9 3 3 <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
9 18 <br />
9 9 9 <br />
<br />
<br />
312 <br />
18 12<br />
3 <br />
<br />
<br />
<br />
3 12 18 12 3 <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
33 <br />
2<br />
918 <br />
9 9 9 <br />
<br />
1 70<br />
<br />
<br />
3 <br />
<br />
<br />
2 <br />
<br />
<br />
<br />
<strong>The</strong> Fundamental <strong>The</strong>orem for Gradients:<br />
Suppose we have a scalar function <strong>of</strong> three variables , , , going from to along<br />
the path , the total change in well be:<br />
Notes:<br />
Line integrals ordinary depend on the path taken from to .<br />
But the right side <strong>of</strong> above equation makes no referencee to the path<br />
– only to the end<br />
points.<br />
<br />
∙
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Evidently, gradient have the special property<br />
that their line integral are path independent.<br />
Corollary(1):<br />
<br />
<br />
∙ is independent <strong>of</strong> path taken from to .<br />
Corollary(2):<br />
∮ ∙ <br />
0 since the beginning <strong>and</strong> end points are identical, <strong>and</strong> hence 0.<br />
Example:<br />
Let <br />
, <strong>and</strong> take point to be the origin 0 , 0 , 0 <strong>and</strong> the<br />
point 2 , 1 , 0. Check<br />
the fundamental theorem for gradients.<br />
Althoughh the integral is independent <strong>of</strong> path, we must pick specific path in order to<br />
evaluate it.<br />
Let’s go out along the<br />
(step ) <strong>and</strong> then up (step );<br />
<strong>The</strong> fundamental theorem <strong>of</strong> Gradient state:<br />
<br />
0 ;<br />
<br />
<br />
<br />
T y 2 <br />
<br />
∙ <br />
<br />
2<br />
<br />
<br />
<br />
⟹<br />
<br />
<br />
2<br />
(i)<br />
∙ 2<br />
<br />
0 ;<br />
0<br />
⟹<br />
T<br />
∙ 0<br />
T<br />
∙ 0
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
(ii)<br />
2<br />
; 0<br />
⟹ T∙ 2 <br />
4 <br />
<br />
T∙ 4 2<br />
1 0 2<br />
<br />
We have 20 2<br />
If we tack<br />
the path (iii):<br />
⟹<br />
<br />
⟹ T∙ <br />
2 <br />
<br />
<br />
T∙ <br />
<br />
<br />
2<br />
<br />
<br />
<strong>The</strong> Fundamental <strong>The</strong>orem for Divergences:<br />
<strong>The</strong> fundamental theorem for divergences state that:<br />
∙ V ∙ <br />
<br />
<br />
This theorem has at least three special names:<br />
Gauss’s theorem , Green’s theorem , or, simply, the Divergence theorem.<br />
It say that the integral <strong>of</strong> a derivative (in this<br />
case the divergence) over a region (in this case<br />
a volume) is equal to<br />
the value <strong>of</strong> the function at the boundary (in this case the surface) that<br />
bounds the volume.<br />
Example:<br />
Check the<br />
divergencee theorem using the function:<br />
2<br />
2 <br />
<strong>and</strong> the unit cube situated at the<br />
origin.<br />
Solution:
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<strong>The</strong> fundamental theorem for divergences state that:<br />
(i)<br />
∙ <br />
<br />
V ∙ <br />
∙ 2<br />
∙ 2 2<br />
<br />
<br />
<br />
<br />
<br />
⟹ <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
∴ ∙ 2<br />
<br />
<br />
<br />
<br />
1 ⟹ 1 1<br />
<br />
<br />
(ii)<br />
(iii)<br />
(iv)<br />
(v)<br />
(vi)<br />
So the total flux is:<br />
<br />
∙ <br />
<br />
<br />
∙ <br />
∙ <br />
<br />
<br />
<br />
∙ <br />
<br />
<br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
2 <br />
<br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
2 1<br />
<br />
<br />
0 0<br />
∙ 1<br />
3 1<br />
3 4<br />
3 1<br />
3 102<br />
<br />
29
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Problem:<br />
Test the divergence theorem for the function:<br />
2 3 <br />
Take as your volume the cube shown in Fig., with sides <strong>of</strong> length (2).<br />
Solution:<br />
<strong>The</strong> fundamental theorem for divergences state that:<br />
<br />
∙ <br />
<br />
<br />
∙ <br />
<br />
∙ <br />
<br />
∙ <br />
∙<br />
<br />
∙ V ∙ <br />
∙ 23<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
2 4 6 <br />
∙ 8 16 <br />
∙ 4 16<br />
2 0<br />
∙163248<br />
<br />
<br />
2<br />
3 <br />
<br />
<br />
23 <br />
<br />
2<br />
<br />
<br />
<br />
<br />
<br />
46 2 0
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Numbering the surfaces as in Fig:<br />
(i)<br />
; 2 ; ∙ 2 <br />
(ii)<br />
<br />
∙ <br />
<br />
<br />
2 8<br />
<br />
; 0 ; ∙ 0<br />
∙ 0<br />
(iii)<br />
; 2 ; ∙ 4 <br />
(iv)<br />
<br />
∙ <br />
<br />
<br />
4 16<br />
<br />
; 0 ; ∙ 0<br />
∙ 0<br />
(v)<br />
; 2 ; ∙ 6 <br />
(vi)<br />
<br />
∙ <br />
<br />
<br />
6 24<br />
<br />
; 0 ; ∙ 0<br />
∙ 0<br />
31
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
So the total flux is:<br />
∙ 8 162448<br />
<strong>The</strong> Fundamental <strong>The</strong>orem for Curls:<br />
<strong>The</strong> fundamental theorem <strong>of</strong> Curls, which goes by the special name <strong>of</strong> Stokes’ theorem state<br />
that:<br />
<br />
<br />
∙ V<br />
As always, the integral <strong>of</strong> a derivative (here, the curl) over a region (here, a patch <strong>of</strong> surface)<br />
is equal to value <strong>of</strong> the function at the boundary (here, the perimeter <strong>of</strong> the patch).<br />
∙ <br />
If your finger point in<br />
the direction <strong>of</strong> the line integral, then your thumb fixed the direction<br />
<strong>of</strong> .<br />
Corollary(1):<br />
<br />
∙ depends only on the boundary line, not on the particular surface<br />
<strong>use</strong>d.<br />
Corollary(2):<br />
∮ <br />
∙ 0 for any closed surface.<br />
Example:<br />
Suppose<br />
2 3 4 <br />
Check Stokes’ theorem for the square surface in Fig.
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<strong>The</strong> Stokes’ theorem state that:<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
∙ V<br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
∵ 0<br />
4 2 2 <br />
⟹ ∙ 4 2 <br />
∙ 4<br />
3<br />
<br />
⟹ ∙ 4<br />
<br />
<br />
Breaking the line integral into four segments:<br />
(i)<br />
0<br />
; 0 ; ∙ 3 <br />
(ii)<br />
0<br />
; 1 ; ∙ 4 <br />
(iii)<br />
0<br />
(iv)<br />
; 1 ; ∙ 3 <br />
<br />
0 ; 0 ; ∙ 0<br />
⟹<br />
⟹<br />
⟹<br />
⟹<br />
∙ 3 1<br />
∙ 4 4 3<br />
∙ <br />
3 1<br />
∙ 0 <br />
0
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics D<br />
So:<br />
V ∙ 1<br />
4<br />
3 10<br />
4<br />
3<br />
Problem:<br />
Test Stokes’ theorem<br />
for the function:<br />
2 3 <br />
Using the<br />
triangular shaded arm <strong>of</strong> Fig.<br />
<strong>The</strong> Stokes’ theorem state that:<br />
∙ V ∙ <br />
<br />
<br />
<br />
<br />
0 2 03 0 <br />
<br />
2 3<br />
2 3 <br />
⟹ ∙ 2 <br />
<br />
∙ 2 <br />
<br />
<br />
∙ 2<br />
0 <br />
<br />
<br />
∙ 2 <br />
<br />
<br />
∙ 44<br />
<br />
<br />
∙ 4 2 <br />
<br />
<br />
Department
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
∙ 88 <br />
<br />
Meanwhile;<br />
∙ 2 3 <br />
<strong>The</strong>re are three segments:<br />
(i)<br />
(ii)<br />
0 ; 0 ; : 0 ⟶ 2<br />
∙ 0 ⟹ ∙ 0<br />
0 ; 2 ; 0 ; ; : 2 ⟶ 0<br />
∙ 2 22 ⟹ ∙ 22 <br />
∙ 4 <br />
<br />
<br />
∙ 2 <br />
<br />
<br />
∙ 8 2<br />
3 8 8<br />
3<br />
<br />
<br />
(iii)<br />
So:<br />
0 ; 0 ; : 2 ⟶ 0<br />
∙ 0 ⟹ ∙ 0<br />
V ∙ 8<br />
3<br />
35
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Orthogonal Coordinate Systems:<br />
<strong>The</strong> most st<strong>and</strong>ardd orthogonal coordinate<br />
systems that are commonly <strong>use</strong>d are:<br />
(1) Cartesian (or Rectangular) Coordinate System , ,<br />
(2) Spherical Coordinate System<br />
, , <br />
(3) Cylindrical Coordinate System<br />
, ,<br />
Spherical Polar Coordinates:<br />
<strong>The</strong> spherical plot coordinates<br />
, , <strong>of</strong> a point are definedd in Fig;<br />
∶ 0 ⟶ ∞<br />
∶ 0 ⟶ <br />
∶ 0 ⟶ 2<br />
: is the distance from the origin (the magnitude <strong>of</strong> the<br />
position <strong>vector</strong>).<br />
: the angle down from the z‐axis, is called<br />
the polar angle.<br />
: the angle around<br />
from the x‐axis, is the azimuthal angle.<br />
<strong>The</strong>ir relation to Cartesian coordinates , , can read from the<br />
figure.<br />
sin cos <br />
sin sin <br />
cos<br />
Any <strong>vector</strong> can be expressed as:<br />
<br />
, & are the<br />
radial, polar & azimuthal components <strong>of</strong> .<br />
, & are unit <strong><strong>vector</strong>s</strong>, pointing in the direction <strong>of</strong><br />
increase <strong>of</strong><br />
the corresponding<br />
coordinates.<br />
In terms <strong>of</strong> the Cartesian unit <strong><strong>vector</strong>s</strong>:<br />
sin<br />
cos sin sin cos <br />
coscos cos sin sin <br />
sin cos
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> S<br />
Check: ∙ ∙ ∙ 1<br />
∙ ∙ ∙ 0<br />
; ; <br />
∙ <br />
<br />
sin cos sin sin cos <br />
cos cos cos sin sin<br />
sin cos 0<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
An infinitesimal displacement in the direction is:<br />
<br />
An infinitesimal element <strong>of</strong> length in the direction is:<br />
<br />
An infinitesimal element <strong>of</strong> length in the direction is:<br />
sin<br />
Thus, the<br />
general infinitesimal displacement<br />
is:<br />
sin<br />
<br />
<strong>The</strong> infinitesimal volume element , in spherical coordinates, is:<br />
<br />
sin <br />
For surface elements<br />
:<br />
1‐ If is constant:<br />
sin <br />
Science – Physics Department
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics D<br />
2‐ If is constant:<br />
sin <br />
3‐ If is constant:<br />
<br />
<br />
In general:<br />
sin sin <br />
<br />
Here, are<br />
the <strong>vector</strong> derivative in<br />
spherical coordinates:<br />
Gradient:<br />
T ∂ T<br />
∂r 1 ∂T<br />
<br />
∂θ 1 ∂T<br />
s in ∂φ <br />
Divergence:<br />
1 ∂<br />
∙V <br />
∂r 1 ∂<br />
sin ∂θ sin 1 ∂<br />
<br />
sin ∂φ <br />
Curl:<br />
V 1<br />
sin<br />
∂ ∂θ sin<br />
∂ <br />
∂φ 1 1 ∂ <br />
∂ sin ∂φ ∂r <br />
1 ∂ ∂r ∂ <br />
∂θ<br />
<br />
Laplacian:<br />
1 ∂ ∂T<br />
T ∂r ∂r 1 ∂<br />
sin ∂θ sin ∂T<br />
∂θ 1 ∂ T<br />
sin <br />
<br />
∂φ Department
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Problem:<br />
Compute the Gradient <strong>and</strong> Laplacian <strong>of</strong> the function<br />
Solution:<br />
cossincos<br />
T ∂T<br />
∂r 1 <br />
∂T<br />
∂θ <br />
1<br />
sin <br />
∂T<br />
∂φ <br />
T cos sin cos sin cos cos 1<br />
sin sin sin <br />
cos sin cos sin cos cos sin <br />
T ∙<br />
T 1 ∂ ∂T<br />
<br />
∂r ∂r 1<br />
sin <br />
T 1 ∂<br />
1<br />
∂r cos sin cos <br />
sin <br />
<br />
1<br />
sin <br />
∂<br />
sin sin <br />
∂φ<br />
1 ∂ ∂r cos sin cos 1<br />
sin<br />
<br />
1<br />
sin <br />
∂<br />
sin <br />
∂φ<br />
∂ ∂T<br />
sin<br />
∂θ ∂θ 1<br />
sin <br />
∂ T<br />
∂φ <br />
∂<br />
sin sin cos cos <br />
∂θ<br />
∂<br />
sin sin cos cos <br />
∂θ<br />
1 1<br />
2 cos sin cos <br />
sin 2 sin cos cos cossin cos<br />
1<br />
<br />
sin cos <br />
1<br />
<br />
sin 2sincos2sin cos2 sincoscos cossin cos<br />
cos<br />
<br />
1<br />
sin sin cos cos cos 0<br />
Problem:<br />
Check the divergence theorem for the function , using spherical volume <strong>of</strong> radius<br />
, centered at the origin.<br />
Divergence theorem state:<br />
∙ <br />
V ∙ <br />
<br />
<br />
39
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
∙V 1 ∂<br />
∂r 1<br />
sin<br />
∙V 1 ∂<br />
∂r 1<br />
sin<br />
∂<br />
∂θ sin <br />
1<br />
sin <br />
∂<br />
∂θ 0 1<br />
sin <br />
∙V 1 4 4 <br />
∂<br />
∂φ <br />
∂<br />
∂φ 0<br />
∙ 4 sin <br />
<br />
4 sin <br />
<br />
<br />
<br />
<br />
4 <br />
22 4<br />
4<br />
V ∙ ∙ sin <br />
<br />
At surface <strong>of</strong> sphere .<br />
<br />
<br />
sin<br />
<br />
<br />
4π <br />
Problem:<br />
Check the divergence theorem for the function , using as your volume the<br />
sphere <strong>of</strong> radius , centered at the origin.<br />
Divergence theorem state:<br />
∙ <br />
∙V 1 ∂<br />
∂r 1<br />
sin<br />
∙V 1 ∂<br />
∂r 1 1<br />
sin<br />
<br />
V ∙ <br />
<br />
∂<br />
∂θ sin <br />
∙V 0<br />
∙ 0<br />
1<br />
sin <br />
∂<br />
∂θ 0 1<br />
sin <br />
∂<br />
∂φ <br />
∂<br />
∂φ 0<br />
V ∙ 1 ∙ sin <br />
40
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<br />
<br />
sin<br />
<br />
<br />
<br />
4π<br />
<strong>The</strong>y don’t agree!!!!! !!<br />
Problem:<br />
Compute<br />
the divergence <strong>of</strong> the function:<br />
cos sin <br />
sin cos <br />
Check the<br />
divergencee theorem for this function, using as<br />
your volume the inverted<br />
hemispherical bowl <strong>of</strong> radius , resting on the plane<br />
<strong>and</strong> centered at the origin.<br />
Divergence theorem state:<br />
∙ V ∙ <br />
<br />
<br />
1 ∂<br />
∙V <br />
∂r 1 ∂<br />
sin ∂θ sin 1 ∂<br />
<br />
sin ∂φ <br />
1 ∂<br />
1 ∂<br />
<br />
∂r cos sin ∂θ si<br />
1 ∂<br />
in sin<br />
<br />
sin ∂φ sin cos <br />
1 1<br />
1<br />
3 cos 2 sincos <br />
sin sin sin sin<br />
<br />
3 cos 2 cos sin <br />
5 cossin<br />
∙ 5 cos sin sin <br />
<br />
/ <br />
5 cos sin sin <br />
<br />
/<br />
<br />
3 5 coscos 2 0 sin
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<br />
<br />
/<br />
3 2 5 cos sin <br />
<br />
<br />
sin <br />
10 <br />
3 2 <br />
/<br />
<br />
5<br />
3 <br />
We have two surfaces:<br />
1) <strong>The</strong> hemisphere:<br />
sin<br />
<br />
; : 0 → 2 ;<br />
: 0 → /2<br />
V ∙ cos sin<br />
<br />
/<br />
<br />
sincos<br />
<br />
<br />
<br />
π <br />
2) <strong>The</strong> flat bottom: sin<br />
<br />
/2 ; : 0 → 2 ; : 0 → <br />
V ∙ sin sin<br />
<br />
<br />
<br />
<br />
<br />
<strong>The</strong> total:<br />
<br />
2π <br />
3<br />
V ∙ π <br />
2π <br />
3 5 3 π <br />
Problem:<br />
Test the Gradient theorem for the function:<br />
cos sincos<br />
Using the<br />
path shown in Figure, from 0 , 0 , 0 to 0 , 0 , 2.
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Solution:<br />
<strong>The</strong> Gradient theorem state:<br />
<br />
∙ <br />
<br />
T ∂T<br />
∂r 1 <br />
<br />
∂T<br />
∂θ <br />
1<br />
sin <br />
∂T<br />
∂φ <br />
T cos sin cos sin cos cos 1<br />
sin sin sin <br />
cos sin cos sin cos cos sin <br />
Segment (1):<br />
<br />
; 0 ; : 0 →2<br />
; T∙ cos sin cos 01 <br />
T∙ <br />
<br />
<br />
2<br />
Segment (2):<br />
<br />
; 2 ; : 0 → <br />
sin 2 ; T∙ sin2 2sin<br />
/<br />
T∙ 2sin<br />
<br />
2cos| / 2<br />
Segment (3):<br />
2 ; <br />
; : →0<br />
2 ; T∙ sin cos cos 2 2sin<br />
Total:<br />
Mean while;<br />
T∙ 2sin<br />
<br />
/<br />
T∙ <br />
<br />
<br />
<br />
2cos| /<br />
2222<br />
2<br />
0 ; 2 ; 2<br />
43
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Cylindrical Coordinates:<br />
<strong>The</strong> cylindrical coordinates , , <strong>of</strong> a point are defined in Fig.<br />
: is the<br />
distance from the z‐axis.<br />
∶<br />
∶<br />
0 ⟶ ∞<br />
0 ⟶ 2<br />
∶<br />
∞ ⟶ ∞<br />
<strong>The</strong>ir relation to Cartesian coordinates , , is:<br />
cos<br />
sin<br />
<br />
<br />
<br />
Any <strong>vector</strong> can be expressed as:<br />
<br />
, & are unit <strong><strong>vector</strong>s</strong>, pointing in the direction <strong>of</strong><br />
increase <strong>of</strong><br />
the corresponding<br />
coordinates. In terms<br />
<strong>of</strong> the Cartesian unit <strong><strong>vector</strong>s</strong>:<br />
cos<br />
sin<br />
<br />
sin cos <br />
<br />
∙<br />
<br />
<br />
<br />
<br />
cos <br />
sin <br />
0<br />
<br />
sin <br />
cos <br />
0<br />
<br />
0<br />
0<br />
1
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<strong>The</strong> infinitesimal displacements in cylindrical coordinates are:<br />
<br />
<br />
<br />
Thus, the general infinitesimal displacement is:<br />
<br />
<strong>The</strong> infinitesimal volume element , in cylindrical coordinates, is:<br />
<br />
<strong>The</strong> infinitesimal surface elements , in cylindrical coordinates, is:<br />
<br />
<strong>The</strong> <strong>vector</strong> derivatives in cylindrical coordinates are:<br />
Gradient:<br />
Divergence:<br />
Curl:<br />
Laplacian:<br />
V 1 <br />
∙V 1 <br />
T ∂T<br />
∂ 1 <br />
∂<br />
∂ 1 <br />
∂T<br />
∂φ ∂T<br />
∂z <br />
∂<br />
∂φ ∂ ∂z <br />
∂ <br />
∂ ∂ <br />
∂z ∂ <br />
∂z ∂ <br />
∂ 1 ∂<br />
∂ ∂ <br />
∂ <br />
T 1 <br />
∂<br />
∂<br />
∂T<br />
<br />
∂ 1 ∂ T<br />
∂φ ∂ T<br />
∂z <br />
Problem:<br />
a) Fide the divergence <strong>of</strong> the function:<br />
V 2sin φ sinφcosφ 3z <br />
45
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
b) Test the divergence theorem for this function, using the quarter‐cylinder (radius 2, height<br />
5) shown in Fig.<br />
c) Find the curl <strong>of</strong> V.<br />
a))<br />
∙V 1<br />
<br />
∙V 1<br />
<br />
∂<br />
∂<br />
∂<br />
∂ 1 <br />
2<br />
sin φ<br />
1 <br />
∂<br />
∂φ ∂ ∂z <br />
<br />
∂<br />
sinφcos<br />
∂φ φ ∂ ∂z 3 <br />
∙ V V 1 <br />
2 2sin φ<br />
1 cos<br />
φsin φ 3<br />
∙V 4 2 sin φ cos φ sin φ3<br />
∙V 7sin φcos φ<br />
b)) Divergence theorem state:<br />
∙V 8<br />
∙ V ∙ <br />
∙ 8 <br />
<br />
Mean while, the surface integral has five parts:<br />
<br />
<br />
8 <br />
<br />
<br />
/<br />
82 <br />
5 40<br />
<br />
2<br />
<br />
<br />
<br />
<br />
Top: 5 ;<br />
Bottom: 0<br />
<br />
V ∙ 3 15 <br />
V ∙ 15<br />
<br />
15<br />
<br />
; <br />
/
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
V ∙ 3 0<br />
V ∙ 0<br />
Back: <br />
; <br />
V ∙ sincos 0<br />
V ∙ 0<br />
Left: 0 ; <br />
V ∙ sincos 0<br />
Front: 2 ; <br />
V ∙ 0<br />
V ∙ 2sin φ 4 2sin φ <br />
So,<br />
/<br />
V ∙ 4 2sin φ <br />
<br />
<br />
<br />
4 5 25<br />
4<br />
/<br />
V ∙ 15 25 40<br />
2sin φ 2 sin φ 2| / <br />
1cos2<br />
<br />
<br />
/<br />
<br />
/<br />
sin2 <br />
<br />
/<br />
<br />
<br />
4 <br />
/<br />
<br />
/2 sin 0 sin0<br />
c))<br />
V 1 <br />
V 1 <br />
∂ <br />
∂ ∂ <br />
∂z ∂ <br />
∂z ∂ <br />
∂ 1 ∂<br />
∂ ∂ <br />
∂ <br />
∂<br />
∂ 3z ∂ ∂z sinφcosφ ∂ ∂z 2sin φ ∂<br />
∂ 3z <br />
1 ∂<br />
∂ sinφcosφ ∂<br />
∂ 2sin φ <br />
V 00 00 1 2 sin φ cos φ 2 sin φ cos φ 0<br />
47
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Example: If<br />
10 <br />
Determine the flux <strong>of</strong> out <strong>of</strong> the entire surface <strong>of</strong> a cylinder 1 ; 0 1.<br />
z<br />
(i)<br />
(iii)<br />
Solution1: <strong>The</strong>re are three surfaces:<br />
Flux through the surface (i):<br />
x<br />
(ii)<br />
y<br />
Φ E ∙ 10 ∙ <br />
Flux through the surface (ii):<br />
10 <br />
1 ; : 0 → 1 ; : 0 → 2<br />
<br />
Φ 10 10 π <br />
<br />
Φ E ∙ 10 ∙ <br />
<br />
<br />
Flux through the surface (iii):<br />
10 <br />
0 ; : 0 → 1 ; : 0 → 2<br />
<br />
Φ 10 10 π<br />
<br />
Φ E ∙ 10 ∙ <br />
<br />
<br />
10 <br />
1 ; : 0 → 1 ; : 0 → 2<br />
<br />
Φ 10 <br />
<br />
<br />
Flux through all surface <strong>of</strong> a cylinder:<br />
<br />
10<br />
2 1 2π 10π 1<br />
ΦE ∙ 10π 10π 10π 1 0<br />
48
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Solution2:<br />
Using the divergence theorem:<br />
∙E 1 <br />
∙E 1 <br />
ΦE ∙ <br />
<br />
∂<br />
∂ 1 <br />
∂<br />
∂ 10 1 <br />
∙ <br />
<br />
∂<br />
∂φ ∂ ∂z <br />
∂<br />
∂φ 0 ∂ ∂z 10 <br />
∙E 1 2 10 2 10 0<br />
Φ ∙ 0<br />
Classification <strong>of</strong> <strong>Vector</strong> Field:<br />
A <strong>vector</strong> field is uniquely characterized by its divergence <strong>and</strong> curl. All <strong>vector</strong> fields can be<br />
classified in terms <strong>of</strong> their vanishing or non‐vanishing divergence or curl as follows:<br />
(1) A <strong>vector</strong> field A is said to be Solenoid (or divergence less) if ( ∙ 0 ) [such field has<br />
neither source nor sink <strong>of</strong> flux]. Examples <strong>of</strong> such fields are (magnetic field, conduction<br />
current density under steady state condition). <strong>The</strong> Solenoid field can always be<br />
expressed in terms <strong>of</strong> another <strong>vector</strong> such as :<br />
∙ 0 ⇒ A ⇒ ∙ 0<br />
(2) A <strong>vector</strong> field is said to be irrotational (or conservative) if 0, examples <strong>of</strong> such<br />
fields are [Electrostatic field <strong>and</strong> gravitational field]. Thus, an irrotational field can<br />
always be expressed in terms <strong>of</strong> a gradient <strong>of</strong> scalar field , that is:<br />
0 ⇒ A ⇒ 0<br />
(3) <strong>The</strong> field is said to be divergence if ∙ 0.<br />
(4) <strong>The</strong> field is said to be Rotational (or non‐conservative) if 0.<br />
(5) When V0, the field is said to be harmonic.<br />
(6) When the field is independent on position the field is said to be uniform field.<br />
(7) When the field is independent on time the field is said to be constant field.<br />
49
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Transformation between Coordinate Systems<br />
<strong>The</strong> position <strong>of</strong> a given point in space is invariant with respect to the choice <strong>of</strong> coordinate<br />
system. That is, its location is the same irrespective <strong>of</strong> which specific coordinate system is<br />
<strong>use</strong>d to represent it. <strong>The</strong> same is true for <strong><strong>vector</strong>s</strong>. <strong>The</strong> relation between the variables<br />
( x,<br />
y,<br />
z)<br />
‐Cartesian ( ,<br />
,<br />
z)<br />
Cylindrical <strong>and</strong> ( r,<br />
, )<br />
in Spherical coordinates will be<br />
established to transform any one <strong>of</strong> the three system into <strong><strong>vector</strong>s</strong> expressed in any <strong>of</strong> the<br />
other two.<br />
1. Cartesian to Cylindrical Transformation:<br />
Point P in the figure has Cartesian coordinate ( x,<br />
y,<br />
z)<br />
<strong>and</strong> cylindrical coordinate ( ,<br />
,<br />
z)<br />
. Both systems share the coordinate (z), <strong>and</strong> the relation between the other two pairs <strong>of</strong><br />
coordinates can be obtained as follows:<br />
ρ<br />
ρ<br />
â z<br />
â <br />
â <br />
sin<br />
cos<br />
x cos<br />
y sin<br />
z z<br />
<br />
x<br />
tan<br />
2<br />
1<br />
<br />
<br />
<br />
<br />
y<br />
y<br />
x<br />
2<br />
<br />
<br />
<br />
aˆ<br />
aˆ<br />
aˆ<br />
x<br />
x<br />
x<br />
aˆ<br />
aˆ<br />
aˆ<br />
<br />
<br />
z<br />
cos<br />
sin<br />
0<br />
aˆ<br />
aˆ<br />
aˆ<br />
y<br />
y<br />
y<br />
aˆ<br />
aˆ<br />
aˆ<br />
<br />
<br />
z<br />
sin<br />
cos<br />
0<br />
aˆ<br />
aˆ<br />
aˆ<br />
z<br />
z<br />
z<br />
aˆ<br />
aˆ<br />
aˆ<br />
<br />
<br />
z<br />
0<br />
0<br />
1<br />
<strong>The</strong>refore, when we have a <strong>vector</strong> in Cartesian coordinate given by:<br />
<br />
A A aˆ<br />
A aˆ<br />
A aˆ<br />
x<br />
x<br />
y<br />
y<br />
z<br />
z<br />
50
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<strong>The</strong>n this <strong>vector</strong> is transformed to cylindrical coordinate as follows:<br />
<br />
<br />
<br />
A ˆ ˆ ˆ ˆ ˆ ( ˆ ˆ<br />
<br />
A a<br />
A<br />
x<br />
( ax<br />
a<br />
) A<br />
y<br />
( a<br />
y<br />
a<br />
) A<br />
z<br />
az<br />
a<br />
)<br />
<br />
<br />
<br />
A aˆ<br />
( ˆ ˆ ) ( ˆ ˆ ) ( ˆ ˆ<br />
<br />
A <br />
<br />
A<br />
x<br />
ax<br />
a<br />
A<br />
y<br />
a<br />
y<br />
a<br />
A<br />
z<br />
az<br />
a<br />
)<br />
<br />
<br />
<br />
A A aˆ<br />
A ( aˆ<br />
aˆ<br />
) A ( aˆ<br />
aˆ<br />
) A ( aˆ<br />
aˆ<br />
)<br />
z<br />
z<br />
x<br />
x<br />
z<br />
y<br />
y<br />
z<br />
In matrix notation, we can write the transformation <strong>of</strong> <strong>vector</strong> (A) from A , A , A ) to<br />
( A , A<br />
, A<br />
z<br />
) as:<br />
z<br />
z<br />
z<br />
(<br />
x y z<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
<br />
z<br />
<br />
<br />
<br />
<br />
aˆ<br />
<br />
a<br />
ˆ<br />
<br />
a<br />
ˆ<br />
x<br />
x<br />
x<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
z<br />
aˆ<br />
aˆ<br />
y<br />
aˆ<br />
y<br />
y<br />
aˆ<br />
aˆ<br />
<br />
<br />
aˆ<br />
z<br />
aˆ<br />
z<br />
aˆ<br />
z<br />
aˆ<br />
z<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
z<br />
<br />
<br />
<br />
<br />
<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
x<br />
y<br />
z<br />
<br />
<br />
<br />
<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
<br />
z<br />
<br />
<br />
<br />
<br />
cos<br />
<br />
<br />
<br />
sin<br />
<br />
0<br />
sin<br />
cos<br />
0<br />
0<br />
0<br />
<br />
<br />
1<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
x<br />
y<br />
z<br />
<br />
<br />
<br />
<br />
While when we have a <strong>vector</strong> ( A ) in cylindrical coordinate given by:<br />
<br />
A( ,<br />
,<br />
z)<br />
A aˆ<br />
A aˆ<br />
A ˆ , then this <strong>vector</strong> can be transformed to Cartesian<br />
<br />
<br />
coordinate as:<br />
<br />
A ˆ<br />
x<br />
A a<br />
x<br />
A<br />
<br />
A ˆ<br />
y<br />
A a<br />
y<br />
A<br />
<br />
A A aˆ<br />
A<br />
z<br />
z<br />
<br />
<br />
<br />
( aˆ<br />
( aˆ<br />
( aˆ<br />
<br />
<br />
<br />
<br />
<br />
z<br />
a z<br />
<br />
aˆ<br />
( ˆ<br />
x<br />
) A<br />
a<br />
<br />
aˆ<br />
) ( ˆ<br />
y<br />
A<br />
a<br />
<br />
aˆ<br />
) A ( aˆ<br />
z<br />
<br />
<br />
<br />
<br />
<br />
aˆ<br />
x<br />
) A<br />
<br />
aˆ<br />
y<br />
) A<br />
<br />
aˆ<br />
) A<br />
z<br />
z<br />
z<br />
( aˆ<br />
z<br />
( aˆ<br />
( aˆ<br />
<strong>The</strong>se equations in matrix notation can be written as:<br />
z<br />
z<br />
z<br />
aˆ<br />
aˆ<br />
aˆ<br />
x<br />
z<br />
y<br />
)<br />
)<br />
)<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
x<br />
y<br />
z<br />
aˆ<br />
<br />
<br />
a<br />
ˆ<br />
<br />
<br />
a<br />
ˆ<br />
<br />
<br />
<br />
aˆ<br />
aˆ<br />
aˆ<br />
x<br />
y<br />
z<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
aˆ<br />
aˆ<br />
x<br />
y<br />
z<br />
aˆ<br />
aˆ<br />
z<br />
z<br />
aˆ<br />
z<br />
aˆ<br />
aˆ<br />
aˆ<br />
x<br />
y<br />
z<br />
<br />
<br />
<br />
<br />
<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
<br />
z<br />
<br />
<br />
<br />
<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
x<br />
y<br />
z<br />
<br />
<br />
<br />
<br />
cos<br />
<br />
<br />
<br />
sin<br />
<br />
0<br />
sin<br />
cos<br />
0<br />
0<br />
0<br />
<br />
<br />
1<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
<br />
z<br />
<br />
<br />
<br />
<br />
2. Cartesian to Spherical Transformation:<br />
Point P in the figure has Cartesian coordinate ( x,<br />
y,<br />
z)<br />
<strong>and</strong> spherical coordinate ( r , , )<br />
.<br />
<strong>The</strong> relation between the coordinates can be obtained as follows:<br />
51
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
â r<br />
â <br />
â <br />
r sin<br />
r sin cos<br />
r sin sin<br />
x r sin<br />
cos<br />
y r sin<br />
sin<br />
z r cos<br />
r <br />
x<br />
tan<br />
2<br />
1<br />
cos<br />
y<br />
<br />
<br />
<br />
1<br />
y<br />
x<br />
<br />
<br />
<br />
<br />
<br />
<br />
z<br />
r<br />
2<br />
<br />
<br />
<br />
z<br />
2<br />
aˆ<br />
aˆ<br />
aˆ<br />
x<br />
x<br />
x<br />
aˆ<br />
r<br />
aˆ<br />
<br />
aˆ<br />
<br />
sin<br />
cos<br />
cos<br />
cos<br />
sin<br />
aˆ<br />
aˆ<br />
aˆ<br />
y<br />
y<br />
y<br />
aˆ<br />
aˆ<br />
<br />
aˆ<br />
r<br />
<br />
sin<br />
sin<br />
cos<br />
sin<br />
cos<br />
aˆ<br />
aˆ<br />
aˆ<br />
z<br />
z<br />
z<br />
aˆ<br />
aˆ<br />
aˆ<br />
r<br />
<br />
<br />
cos<br />
sin<br />
0<br />
<strong>The</strong>refore, when we have a <strong>vector</strong> in Cartesian coordinate given by:<br />
<br />
A A aˆ<br />
A aˆ<br />
A aˆ<br />
x<br />
x<br />
y<br />
y<br />
z<br />
z<br />
<strong>The</strong>n this <strong>vector</strong> is transformed to spherical coordinate as follows:<br />
<br />
A<br />
<br />
A<br />
<br />
A<br />
r<br />
<br />
<br />
<br />
A aˆ<br />
<br />
A aˆ<br />
<br />
A aˆ<br />
r<br />
<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
A<br />
x<br />
x<br />
x<br />
( aˆ<br />
x<br />
( aˆ<br />
( aˆ<br />
x<br />
x<br />
<br />
aˆ<br />
( ˆ<br />
r<br />
) A<br />
y<br />
a<br />
y<br />
<br />
aˆ<br />
) ( ˆ<br />
<br />
A<br />
y<br />
a<br />
<br />
aˆ<br />
) A ( aˆ<br />
<br />
y<br />
y<br />
y<br />
<br />
aˆ<br />
( ˆ ˆ<br />
r<br />
) A<br />
z<br />
az<br />
ar<br />
)<br />
<br />
aˆ<br />
) ( ˆ ˆ<br />
<br />
A<br />
z<br />
az<br />
a<br />
)<br />
<br />
aˆ<br />
) A ( aˆ<br />
aˆ<br />
)<br />
In matrix notation, we can write the transformation <strong>of</strong> <strong>vector</strong> (A) from A , A , A ) to<br />
( A r<br />
, A , A<br />
) as:<br />
<br />
z<br />
z<br />
<br />
(<br />
x y z<br />
Ar<br />
aˆ<br />
x<br />
aˆ<br />
<br />
A<br />
a<br />
ˆx<br />
aˆ<br />
<br />
<br />
A<br />
a<br />
ˆx<br />
aˆ<br />
r<br />
<br />
<br />
aˆ<br />
aˆ<br />
y<br />
y<br />
aˆ<br />
y<br />
aˆ<br />
r<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
aˆ<br />
z<br />
z<br />
z<br />
r<br />
aˆ<br />
aˆ<br />
<br />
aˆ<br />
aˆ<br />
<br />
<br />
<br />
<br />
<br />
<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
x<br />
y<br />
z<br />
<br />
<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
A<br />
r<br />
<br />
<br />
sin<br />
cos<br />
<br />
<br />
<br />
<br />
cos<br />
cos<br />
<br />
<br />
<br />
sin<br />
sin<br />
sin<br />
cos<br />
sin<br />
cos<br />
cos<br />
<br />
sin<br />
<br />
<br />
0 <br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
x<br />
y<br />
z<br />
<br />
<br />
<br />
<br />
52
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
While when we have<br />
a <strong>vector</strong> ( A ) in spherical coordinate given by:<br />
A ( r , ,<br />
<br />
) A ˆ <br />
A<br />
coordinate as:<br />
<br />
A<br />
x<br />
A aˆ<br />
x<br />
A<br />
<br />
A<br />
y<br />
A aˆ<br />
y<br />
A<br />
<br />
A A aˆ<br />
A<br />
z<br />
z<br />
r<br />
a r<br />
r<br />
r<br />
r<br />
( a<br />
ˆ<br />
(<br />
a<br />
ˆ<br />
(<br />
a<br />
ˆ<br />
r<br />
r<br />
r<br />
<br />
â<br />
<br />
A<br />
<strong>The</strong>se equations in matrix notation can be written as:<br />
<br />
<br />
aˆ<br />
x<br />
) A<br />
<br />
aˆ<br />
y<br />
) A<br />
<br />
aˆ<br />
) A<br />
z<br />
aâ , then this<br />
<strong>vector</strong> can be transformed to Cartesian<br />
<br />
<br />
<br />
<br />
<br />
( aˆ<br />
ˆ<br />
<br />
ax<br />
) A ( ˆ ˆ<br />
<br />
a<br />
ax<br />
)<br />
<br />
( aˆ<br />
aˆ<br />
y<br />
)<br />
A ( ˆ ˆ<br />
<br />
a<br />
a<br />
y<br />
)<br />
<br />
( aˆ<br />
aˆ<br />
) A ( aˆ<br />
aˆ<br />
)<br />
<br />
z<br />
<br />
<br />
z<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
aˆ<br />
r<br />
aˆ<br />
<br />
<br />
a<br />
ˆr<br />
aˆ<br />
<br />
<br />
a<br />
ˆr<br />
aˆ<br />
x<br />
y<br />
z<br />
x<br />
y<br />
z<br />
aˆ<br />
a<br />
ˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
x<br />
y<br />
z<br />
aˆ<br />
aˆ<br />
<br />
<br />
<br />
x<br />
aˆ<br />
aˆ<br />
y<br />
aˆ<br />
aˆ<br />
z<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
A<br />
<br />
A<br />
A r<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
A<br />
x <br />
A<br />
<br />
y<br />
<br />
<br />
A <br />
z <br />
sin<br />
cos<br />
<br />
<br />
<br />
sin<br />
sin<br />
<br />
cos<br />
<br />
cos<br />
cos<br />
cos<br />
sin<br />
<br />
sin<br />
sin<br />
cos<br />
<br />
<br />
<br />
0 <br />
<br />
<br />
A<br />
<br />
A<br />
A r<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
3. Cylindrical to Spherical Transformation:<br />
Point P in the figure has cylindrical coordinate ( ,<br />
,<br />
z)<br />
<strong>and</strong> spherical coordinate ( r , , <br />
) .<br />
<strong>The</strong> relation between<br />
the coordinates can be<br />
obtained as follows:<br />
x <br />
r sin<br />
cos<br />
y <br />
r sin<br />
sin<br />
z <br />
r cos<br />
<strong>and</strong><br />
<strong>and</strong><br />
<strong>and</strong><br />
x cos<br />
y <br />
sin<br />
z <br />
z<br />
r sin<br />
z r cos<br />
<br />
r<br />
<br />
<br />
x<br />
tan<br />
tan<br />
2<br />
<br />
2<br />
1<br />
<br />
<br />
<br />
<br />
1<br />
<br />
<br />
<br />
z<br />
y<br />
y<br />
x<br />
<br />
z<br />
2<br />
2
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
aˆ<br />
aˆ<br />
aˆ<br />
<br />
<br />
<br />
aˆ<br />
aˆ<br />
aˆ<br />
r<br />
<br />
<br />
sin<br />
cos<br />
0<br />
aˆ<br />
aˆ<br />
aˆ<br />
<br />
<br />
<br />
aˆ<br />
aˆ<br />
aˆ<br />
r<br />
<br />
<br />
0<br />
0<br />
1<br />
aˆ<br />
aˆ<br />
aˆ<br />
z<br />
z<br />
z<br />
aˆ<br />
aˆ<br />
aˆ<br />
r<br />
<br />
<br />
cos<br />
sin<br />
0<br />
<strong>The</strong>refore, when we have a <strong>vector</strong> in cylindrical coordinate given by:<br />
<br />
A A aˆ<br />
A aˆ<br />
A ˆ<br />
<br />
<br />
<br />
<br />
z<br />
a z<br />
<strong>The</strong>n this <strong>vector</strong> is transformed to spherical coordinate as follows:<br />
<br />
<br />
<br />
A ˆ ˆ ˆ ˆ ˆ ˆ ˆ<br />
r<br />
A ar<br />
A<br />
<br />
( a<br />
ar<br />
) A<br />
( a<br />
ar<br />
) A<br />
z<br />
( az<br />
ar<br />
)<br />
<br />
<br />
<br />
A ˆ ( ˆ ˆ ) ( ˆ ˆ ) ( ˆ ˆ<br />
<br />
A a<br />
A<br />
<br />
a<br />
a<br />
A<br />
a<br />
a<br />
A<br />
z<br />
az<br />
a<br />
)<br />
<br />
<br />
<br />
A A aˆ<br />
A ( aˆ<br />
aˆ<br />
) A ( aˆ<br />
aˆ<br />
) A ( aˆ<br />
aˆ<br />
)<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
In matrix notation, we can write the transformation <strong>of</strong> <strong>vector</strong> (A) from A , A<br />
, A ) to<br />
( , A , A<br />
) as:<br />
A r<br />
z<br />
z<br />
<br />
(<br />
z<br />
A<br />
<br />
A<br />
<br />
A<br />
r<br />
<br />
<br />
aˆ<br />
<br />
a<br />
ˆ<br />
<br />
a<br />
ˆ<br />
<br />
<br />
<br />
aˆ<br />
r<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
r<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
aˆ<br />
z<br />
z<br />
z<br />
r<br />
aˆ<br />
aˆ<br />
<br />
aˆ<br />
aˆ<br />
<br />
<br />
<br />
<br />
<br />
<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
<br />
z<br />
<br />
<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
A<br />
r<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
sin<br />
<br />
<br />
<br />
cos<br />
<br />
0<br />
0<br />
0<br />
1<br />
cos<br />
<br />
sin<br />
<br />
<br />
0 <br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
<br />
z<br />
<br />
<br />
<br />
<br />
While when we have a <strong>vector</strong> ( A ) in spherical coordinate given by:<br />
<br />
A( r,<br />
,<br />
)<br />
A aˆ<br />
A aˆ<br />
A aˆ<br />
, then this <strong>vector</strong> can be transformed to cylindrical<br />
r<br />
r<br />
<br />
<br />
coordinate as:<br />
<br />
<br />
<br />
A ˆ ˆ ˆ ( ˆ ˆ ˆ ˆ<br />
<br />
A a<br />
A<br />
r<br />
( ar<br />
a<br />
) A<br />
a<br />
a<br />
) A<br />
( a<br />
a<br />
)<br />
<br />
<br />
A aˆ<br />
( ˆ ˆ ) ( ˆ ˆ ) ( ˆ ˆ<br />
<br />
A <br />
<br />
A<br />
r<br />
ar<br />
a<br />
A<br />
a<br />
a<br />
A<br />
a<br />
a<br />
)<br />
<br />
<br />
A A aˆ<br />
A ( aˆ<br />
aˆ<br />
) A ( aˆ<br />
aˆ<br />
) A ( aˆ<br />
aˆ<br />
)<br />
z<br />
z<br />
r<br />
r<br />
z<br />
<strong>The</strong>se equations in matrix notation can be written as:<br />
<br />
<br />
<br />
<br />
z<br />
<br />
<br />
z<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
<br />
z<br />
<br />
<br />
<br />
<br />
aˆ<br />
r<br />
aˆ<br />
<br />
a<br />
ˆr<br />
aˆ<br />
<br />
a<br />
ˆr<br />
aˆ<br />
z<br />
<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
z<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
<br />
aˆ<br />
z<br />
<br />
<br />
<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
A<br />
r<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
<br />
z<br />
<br />
<br />
<br />
<br />
sin<br />
<br />
<br />
<br />
0<br />
<br />
cos<br />
cos<br />
o<br />
sin<br />
0<br />
1<br />
<br />
<br />
0<br />
A<br />
<br />
A<br />
<br />
A<br />
r<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
Example(1):<br />
<br />
Let A cos<br />
aˆ<br />
<br />
z<br />
2<br />
sin<br />
aˆ<br />
z<br />
, then :<br />
a. Transform A into Cartesian coordinate <strong>and</strong> calculate its magnitude at point (3,‐4,0).<br />
b. Transform A into spherical coordinate <strong>and</strong> calculate its magnitude at point (3,‐4,0).<br />
54
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Solution:<br />
(a). since the <strong>vector</strong> is in cylindrical coordinate, <strong>and</strong> we have the following matrix to<br />
convert it to Cartesian coordinate:<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
x<br />
y<br />
z<br />
<br />
<br />
<br />
<br />
cos<br />
<br />
<br />
<br />
sin<br />
<br />
0<br />
sin<br />
cos<br />
0<br />
0<br />
0<br />
<br />
<br />
1<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
<br />
z<br />
<br />
<br />
<br />
<br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
x<br />
y<br />
z<br />
<br />
<br />
<br />
<br />
cos<br />
<br />
<br />
<br />
sin<br />
<br />
0<br />
sin<br />
cos<br />
0<br />
0<br />
0<br />
<br />
<br />
1<br />
cos<br />
<br />
<br />
<br />
0<br />
<br />
2<br />
<br />
z sin<br />
A<br />
A<br />
A<br />
<br />
x<br />
y<br />
z<br />
cos<br />
2<br />
sin<br />
cos<br />
0 0<br />
0<br />
0 z<br />
A<br />
x<br />
0 0<br />
2<br />
sin<br />
<br />
2<br />
5cos<br />
( 53.13)<br />
1.8<br />
<br />
at point (3,‐4,0) : tan<br />
A<br />
y<br />
z 0<br />
<strong>The</strong>refore, the <strong>vector</strong> in Cartesian coordinate is:<br />
<br />
2<br />
2<br />
A cos aˆ<br />
cos<br />
sin<br />
aˆ<br />
z sin<br />
aˆ<br />
<br />
x<br />
y<br />
x<br />
2<br />
1<br />
y<br />
y<br />
x<br />
2<br />
<br />
tan<br />
9 16<br />
5<br />
1<br />
5sin( 53.13)cos(<br />
53.13)<br />
2.3<br />
z<br />
<br />
A 1.8<br />
aˆ<br />
x<br />
<br />
4<br />
53.13<br />
3<br />
2.3aˆ<br />
y<br />
0<br />
A<br />
z<br />
5<br />
0<br />
sin( 53.13)<br />
0<br />
(b). since the <strong>vector</strong> is in cylindrical coordinate, <strong>and</strong> we have the following matrix to<br />
convert it to spherical coordinate:<br />
A<br />
<br />
A<br />
<br />
A<br />
r<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
sin<br />
<br />
<br />
<br />
cos<br />
<br />
0<br />
0<br />
0<br />
1<br />
cos<br />
<br />
sin<br />
<br />
<br />
0 <br />
A<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
<br />
z<br />
<br />
<br />
<br />
<br />
A<br />
<br />
A<br />
<br />
A<br />
r<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
sin<br />
<br />
<br />
<br />
cos<br />
<br />
0<br />
0<br />
0<br />
1<br />
cos<br />
<br />
sin<br />
<br />
<br />
0 <br />
cos<br />
<br />
<br />
<br />
0<br />
<br />
2<br />
<br />
z sin<br />
<strong>The</strong> spherical point <strong>of</strong> ( 3, ‐4, 0) is given by :<br />
A<br />
r<br />
sin<br />
cos<br />
z<br />
2<br />
cos<br />
sin<br />
A<br />
<br />
r <br />
x<br />
2<br />
cos<br />
tan<br />
y<br />
1<br />
1<br />
z<br />
r<br />
y<br />
x<br />
cos<br />
cos<br />
z<br />
2<br />
2<br />
z<br />
cos<br />
tan<br />
2<br />
1<br />
1<br />
<br />
sin<br />
sin<br />
9 16<br />
0 5<br />
0 <br />
90<br />
5<br />
4<br />
53.13<br />
3<br />
A<br />
<br />
0<br />
0 0 0<br />
A r<br />
A<br />
<br />
5sin 90 cos( 53.13)<br />
5<br />
0<br />
cos90 sin( 53.13)<br />
5cos90<br />
cos( 53.13)<br />
5<br />
0<br />
sin 90 sin( 53.13)<br />
<br />
A<br />
0<br />
<br />
A (<br />
sin<br />
cos<br />
z<br />
<br />
A 3aˆ<br />
0 aˆ<br />
0 aˆ<br />
<br />
<br />
z<br />
2<br />
cos<br />
sin)<br />
aˆ<br />
<br />
A 3aˆ<br />
<br />
<br />
3<br />
0<br />
( cos<br />
cos<br />
z<br />
2<br />
sin<br />
sin)<br />
aˆ<br />
<br />
0 aˆ<br />
z<br />
55
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Example(2):<br />
(a). find the length <strong>of</strong> each <strong>of</strong> the curves: 3,<br />
<br />
,<br />
4 2<br />
z cons tan t.<br />
<br />
(b). Calculate the area <strong>of</strong> the surfaces area defined by: z 1, 1 3 , 0 <br />
4<br />
(c). determine the volume <strong>of</strong> the region defined by: 1 r 3 ,<br />
2<br />
<br />
, <br />
2 3 6 2<br />
Solution:<br />
(a).<br />
dl d<br />
<br />
<br />
dl <br />
/ 2<br />
<br />
d<br />
3 ( / 2 <br />
/ 4)<br />
<br />
4<br />
/ 4<br />
3<br />
l unit<br />
length<br />
(b)<br />
ds d<br />
d<br />
<br />
3 / 4<br />
1 4<br />
3 /<br />
2<br />
<br />
ds <br />
d<br />
d<br />
( ) ( )<br />
4 <br />
2<br />
1 0<br />
4<br />
1<br />
0<br />
<br />
S <br />
unit<br />
<strong>of</strong><br />
area<br />
(c).<br />
dv r<br />
2<br />
sin<br />
dr d<br />
d<br />
<br />
<br />
dv <br />
3 2<br />
/ 3 / 2<br />
<br />
1 / 2<br />
/ 6<br />
3<br />
r<br />
sin<br />
dr d<br />
d<br />
<br />
3<br />
1<br />
v (27 1)<br />
(cos<br />
/ 2 cos 2<br />
/ 3) ( / 2 / 6)<br />
3<br />
1<br />
<br />
13<br />
v (26) (0<br />
0.5) (<br />
) v unit <strong>of</strong><br />
3<br />
3<br />
9<br />
r<br />
2<br />
volume<br />
3<br />
1<br />
( cos<br />
)<br />
2<br />
/ 3<br />
/ 2<br />
( )<br />
/ 2<br />
/ 6<br />
Example(3):<br />
Find the gradient <strong>of</strong> the following scalar functions:<br />
a. U 4 x z<br />
2 3 y z , b. 2 ( z 2 2<br />
W 1)<br />
cos<br />
, c. V r cos cos<br />
.<br />
(a). <br />
U (<br />
<br />
x<br />
aˆ<br />
x<br />
<br />
<br />
y<br />
aˆ<br />
y<br />
<br />
<br />
z<br />
aˆ<br />
)(4xz<br />
z<br />
2<br />
3yz)<br />
<br />
4 z<br />
2<br />
aˆ<br />
x<br />
3z aˆ<br />
y<br />
(8xz<br />
3y)<br />
aˆ<br />
z<br />
(b).<br />
<br />
<br />
W ( aˆ<br />
<br />
<br />
1 <br />
aˆ<br />
<br />
<br />
<br />
<br />
z<br />
aˆ<br />
)(2 (<br />
z<br />
1) cos)<br />
2( z<br />
1) cos<br />
aˆ<br />
2 ( z<br />
1) sin<br />
aˆ<br />
4 z cos<br />
ˆ<br />
2<br />
2<br />
2<br />
z <br />
<br />
a z<br />
56
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
(c).<br />
<br />
<br />
V (<br />
r<br />
aˆ<br />
r<br />
1<br />
<br />
r<br />
<br />
aˆ<br />
<br />
<br />
<br />
r<br />
1<br />
sin<br />
<br />
aˆ<br />
<br />
<br />
)( r<br />
2<br />
cos<br />
cos)<br />
2 r cos<br />
cos<br />
aˆ<br />
r<br />
r sin<br />
cos<br />
aˆ<br />
<br />
r cot<br />
sin<br />
aˆ<br />
<br />
Example(4):<br />
For the <strong>vector</strong> field<br />
<br />
<br />
E 10 e aˆ<br />
3 z aˆ<br />
, verify the divergence theorem for the cylindrical<br />
<br />
z<br />
region enclosed by : 2 m , z 0 <strong>and</strong> z 4 m ?<br />
<strong>The</strong> divergence theorem<br />
mathematically states that:<br />
<br />
E ds ( E)<br />
dv<br />
<br />
S<br />
<br />
Z=4 m<br />
Z<br />
=2 m<br />
<strong>The</strong>re are three closed<br />
surfaces bounded by the<br />
defined region, they are:<br />
Z=0<br />
Y<br />
<strong>The</strong>refore the right h<strong>and</strong> side <strong>of</strong> the equation <strong>of</strong> divergence theorem is:<br />
<br />
E ds E ds E ds E ds<br />
<br />
S<br />
<br />
S1<br />
<br />
S 2<br />
<br />
S 2<br />
<br />
S 2<br />
<br />
S<br />
<br />
E ds <br />
<br />
E ds<br />
<br />
<br />
E ds<br />
<br />
<br />
S1<br />
2<br />
2<br />
<br />
0 0<br />
2<br />
2<br />
<br />
0 0<br />
2<br />
2<br />
<br />
0 0<br />
(10 <br />
(10 <br />
(10 <br />
<br />
E ds<br />
43.3<br />
S 2<br />
<br />
<br />
<br />
aˆ<br />
<br />
E ds 0<br />
48 43.3<br />
4.7<br />
<br />
e<br />
e<br />
e<br />
<br />
aˆ<br />
aˆ<br />
<br />
<br />
<br />
S 3<br />
3 z aˆ<br />
) <br />
d<br />
d<br />
( aˆ<br />
) <br />
z<br />
3 z aˆ<br />
) d<br />
d<br />
aˆ<br />
<br />
z<br />
3 z aˆ<br />
) dz d<br />
aˆ<br />
z<br />
z<br />
<br />
z<br />
<br />
2<br />
2<br />
<br />
0 0<br />
2<br />
2<br />
<br />
0 0<br />
2<br />
2<br />
<br />
0 0<br />
1 2<br />
3 z d<br />
d<br />
3 z (2<br />
) 3<br />
0 2 2<br />
0<br />
2<br />
1 2<br />
3 z d<br />
d<br />
3 z (2<br />
) 3<br />
4<br />
2<br />
2<br />
48<br />
2<br />
10<br />
e<br />
<br />
2<br />
dz d<br />
10<br />
e<br />
<br />
2<br />
z (2<br />
) 10<br />
e<br />
2<br />
4<br />
4 (2<br />
)<br />
While the Left h<strong>and</strong> side is:<br />
1 (<br />
E<br />
<br />
) 1 E<br />
E<br />
z<br />
E <br />
<br />
<br />
<br />
z<br />
1<br />
<br />
2 <br />
E (20 e 10e<br />
) 3 <br />
<br />
<br />
2<br />
1 (<br />
10e<br />
<br />
<br />
) 1 (0) (<br />
3z)<br />
<br />
<br />
z<br />
57
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<br />
v<br />
( <br />
E <br />
) dv <br />
first term<br />
<br />
2<br />
4 2<br />
<br />
0 0 0<br />
2<br />
4 2<br />
<br />
0 0 0<br />
second<br />
term <br />
third term<br />
<br />
(20 e<br />
20 e<br />
2<br />
4 2<br />
<br />
0 0 0<br />
2<br />
4 2<br />
<br />
0 0 0<br />
<br />
<br />
10 e<br />
<br />
10<br />
e<br />
<br />
2<br />
dd<br />
dz 80<br />
e<br />
<br />
3 d<br />
d<br />
dz<br />
d<br />
d<br />
dz 160<br />
e<br />
<br />
d<br />
<br />
2<br />
d<br />
2<br />
<br />
3 d<br />
d<br />
dz 24<br />
d<br />
24<br />
<br />
2<br />
2<br />
0<br />
2<br />
<br />
0<br />
2<br />
<br />
0<br />
by ( u dv)<br />
by ( u dv)<br />
2<br />
0<br />
48<br />
Example(5):<br />
Verify Stoke’s theorem for a <strong>vector</strong> field <br />
, in the segment <strong>of</strong> cylindrical surface<br />
defined by 2 , 60 90 , <strong>and</strong> 0 3 .<br />
<strong>The</strong> mathematical representation <strong>of</strong> Stokes’s theorem is given by:<br />
<br />
L<br />
<br />
A dl<br />
<br />
<br />
L<br />
<br />
A ds<br />
<strong>The</strong> line integral around a closed path defined by these bounded region is as follows:<br />
<br />
<br />
L<br />
b<br />
<br />
a<br />
c<br />
<br />
b<br />
d<br />
<br />
c<br />
a<br />
<br />
d<br />
<br />
B dl<br />
<br />
<br />
B dl<br />
<br />
<br />
B dl<br />
<br />
<br />
B dl<br />
<br />
<br />
B dl<br />
<br />
b<br />
<br />
a<br />
<br />
<br />
<br />
<br />
<br />
B dl<br />
<br />
B aˆ<br />
z<br />
z<br />
z<br />
z<br />
z<br />
B aˆ<br />
z<br />
B aˆ<br />
z<br />
B aˆ<br />
z<br />
c<br />
<br />
b<br />
<br />
d<br />
aˆ<br />
dz aˆ<br />
<br />
B dl<br />
<br />
z<br />
<br />
<br />
0<br />
<br />
d<br />
( aˆ<br />
) 0<br />
3<br />
<br />
0<br />
<br />
dz ( aˆ<br />
) <br />
z<br />
d<br />
c<br />
<br />
B dl<br />
<br />
cos<br />
cos90<br />
dz z<br />
2<br />
<br />
3<br />
<br />
0<br />
a<br />
<br />
d<br />
<br />
B dl<br />
0<br />
cos<br />
cos60<br />
dz z<br />
2<br />
3<br />
0<br />
3<br />
0<br />
3<br />
<br />
4<br />
ρ<br />
<strong>The</strong> left h<strong>and</strong> side <strong>of</strong> the Stokes’s theorem is :<br />
<br />
A <br />
<br />
s<br />
ds<br />
58
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
aˆ<br />
<br />
aˆ<br />
<br />
1 <br />
B <br />
<br />
<br />
0 0<br />
1<br />
B <br />
2<br />
<br />
<br />
cos<br />
aˆ<br />
<br />
aˆ<br />
z<br />
<br />
<br />
z<br />
cos<br />
<br />
sin<br />
aˆ<br />
<br />
<br />
1 <br />
aˆ<br />
<br />
<br />
<br />
<br />
sin<br />
( 0) aˆ<br />
<br />
<br />
cos<br />
(0 ) aˆ<br />
2<br />
<br />
z<br />
<br />
(0 0) <br />
<br />
Hence,<br />
<br />
s<br />
<br />
s<br />
<br />
s<br />
1<br />
( B)<br />
ds cos<br />
aˆ<br />
2<br />
s<br />
<br />
1<br />
( B)<br />
ds (cos)<br />
<br />
3 1<br />
( B)<br />
ds (0 ) <br />
2 2<br />
<br />
<br />
<br />
sin<br />
aˆ<br />
<br />
s<br />
<br />
<br />
dz d<br />
aˆ<br />
<br />
/ 2 3<br />
/ 3 0<br />
sin<br />
dz d<br />
2<br />
<br />
/ 2 3<br />
1<br />
( z)<br />
(cos<br />
/ 2 cos<br />
/ 3) (3 0)<br />
/ 3<br />
0 2<br />
3<br />
( B)<br />
ds <br />
4<br />
It is clearly seen that the left <strong>and</strong> right h<strong>and</strong> side has the same value, which indicates the<br />
validity <strong>of</strong> the Stokes’s theorem.<br />
<br />
<br />
Example (6):<br />
<br />
transform into spherical coordinate <strong>and</strong> find its magnitude at point 3, 4, 0.<br />
Solution:<br />
∙ ∙ ∙ ∙ <br />
∙ ∙ ∙ ∙ <br />
∙ ∙ ∙ ∙ <br />
<br />
∙ ∙ ∙ <br />
∙ ∙ ∙ <br />
∙ ∙ ∙ <br />
<br />
0 <br />
0 <br />
<br />
0 1 0 <br />
<br />
<br />
<br />
<br />
59
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<br />
<br />
√9165<br />
√25 5<br />
0 5 90<br />
4<br />
3 53.13<br />
5sin90 cos53.13 5 0 cos90 sin53.13 <br />
5cos90 cos53.13 5 0 sin 90 sin 53.13 . <br />
3 0 0.018 <br />
Example(7):<br />
Determine the flux <strong>of</strong> through the entire prism shown in Fig.<br />
Solution:<br />
z<br />
c<br />
<strong>The</strong> equation <strong>of</strong> surface (abc) is:<br />
<br />
1<br />
1 ,3 ,6<br />
6 2 6 ⟹ 662<br />
x<br />
a<br />
b<br />
y<br />
<strong>The</strong> equation <strong>of</strong> line (ab) is:<br />
<br />
<br />
<br />
<br />
⟹<br />
<br />
<br />
<br />
3 3 ⟹ 3 3<br />
To find flux through the entire prism we can <strong>use</strong> divergence theorem:<br />
∮ . ∙ ∙ 3 <br />
<br />
<br />
<br />
. 3 <br />
<br />
<br />
<br />
<br />
<br />
. 3 6 6 2 <br />
<br />
<br />
<br />
. 3 63 3 63 3 2<br />
<br />
3 3<br />
<br />
2<br />
60
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<br />
. 27 <br />
<br />
. 27 1 3 1 4 1 5 7.65<br />
Example(8):<br />
Show that if the scalar function , is harmonic at point √3, ,0 .<br />
Solution:<br />
∂<br />
∂θ<br />
H 1 ∂<br />
r ∂r r sin θ e 1<br />
H 1 ∂ ∂H<br />
r r<br />
∂r ∂r 1<br />
r sinθ<br />
sinθ<br />
∂H<br />
∂θ 1<br />
∂ H<br />
r sin θ ∂φ <br />
∂<br />
r sinθ ∂θ sinθ2 sin θ cosθ e <br />
H sin θ<br />
r r e 2r e 2 e<br />
r sinθ sin θ 2sinθ cos θ <br />
H sin θ<br />
r<br />
e r2 2 e<br />
r 2 cos θ sin θ<br />
At point √3, ,0, H0 ⟹ is not harmonic.<br />
1<br />
r sin θ 0<br />
Example (9):<br />
Display whether the field <strong>vector</strong> is solenoid, conservative or none <strong>of</strong> them?<br />
Solution:<br />
(1). In order to show that the field is solenoid, it must be satisfy: ∙A 0<br />
∙A 1 r ∂ ∂r r A 1<br />
rsinθ<br />
<strong>The</strong>refore the field is not solenoid:<br />
∂<br />
∂θ sin θ A 1 ∂A <br />
rsinθ ∂φ<br />
∙A 1 r ∂ ∂r r 1 r r 3 0<br />
(2). In order to show that the field is conservative, it must be satisfy: A 0<br />
<br />
<br />
1<br />
<br />
<br />
1<br />
<br />
<br />
<br />
0 0<br />
1<br />
00 00 00 0<br />
<strong>The</strong>refore the field is conservative.<br />
61
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
More example on <strong>Chapter</strong> <strong>One</strong><br />
Example(10):<br />
Given <strong><strong>vector</strong>s</strong><br />
(a). A B<br />
(b). 3 A<br />
B<br />
<br />
<br />
<br />
A aˆ<br />
3aˆ<br />
<strong>and</strong> B 5aˆ<br />
x<br />
z<br />
x<br />
2aˆ<br />
y<br />
6aˆ<br />
z<br />
, then determine the following:<br />
(c). the component <strong>of</strong> A along aˆ<br />
y<br />
(d). the unit <strong>vector</strong> parallel to 3A<br />
<br />
B<br />
<br />
Solution:<br />
(a). A<br />
B ( aˆ<br />
3aˆ<br />
) (5aˆ<br />
2aˆ<br />
6aˆ<br />
) 6aˆ<br />
2 aˆ<br />
3 aˆ<br />
A<br />
<br />
B <br />
36 4 9 7unit<br />
x<br />
z<br />
x<br />
y<br />
z<br />
x<br />
y<br />
z<br />
(b). 3A<br />
<br />
B <br />
(3aˆ<br />
9aˆ<br />
) (5aˆ<br />
2aˆ<br />
6aˆ<br />
) 2aˆ<br />
2aˆ<br />
15aˆ<br />
x<br />
z<br />
x<br />
y<br />
z<br />
x<br />
y<br />
z<br />
(c). the scalar component <strong>of</strong><br />
<br />
A along aˆ<br />
is given by: A Aaˆ<br />
( aˆ<br />
3aˆ<br />
) aˆ<br />
0<br />
y<br />
y<br />
y<br />
x<br />
z<br />
y<br />
(d). 3A<br />
<br />
B <br />
(3aˆ<br />
9aˆ<br />
) (5aˆ<br />
2aˆ<br />
6aˆ<br />
) 8aˆ<br />
2aˆ<br />
3aˆ<br />
, then the unit <strong>vector</strong><br />
x<br />
z<br />
x<br />
y<br />
z<br />
x<br />
y<br />
z<br />
parallel to this <strong>vector</strong> is calculated as:<br />
<br />
3A<br />
B<br />
a ˆ3 AB<br />
<br />
3A<br />
B<br />
<br />
8aˆ<br />
x<br />
2 aˆ<br />
y<br />
3aˆ<br />
64 4 9<br />
z<br />
<br />
8aˆ<br />
x<br />
2 aˆ<br />
y<br />
77<br />
6 aˆ<br />
z<br />
Example(11):<br />
Given points P( 1, 3,5) , Q(2,4,6)<br />
<strong>and</strong> R(0,3,8)<br />
, then find the following:<br />
(a). the position <strong><strong>vector</strong>s</strong> <strong>of</strong> P <strong>and</strong> R<br />
(b). the distance <strong>vector</strong> r <br />
QP<br />
(c). the distance between Q <strong>and</strong> R<br />
Solution:<br />
62
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
OP (1 0) aˆ<br />
x<br />
( 3<br />
0) aˆ<br />
y<br />
(5 0) aˆ<br />
z<br />
aˆ<br />
(a).<br />
OR (0 0) aˆ<br />
(3 0) aˆ<br />
(8 0) aˆ<br />
3aˆ<br />
x<br />
y<br />
z<br />
x<br />
y<br />
3aˆ<br />
8aˆ<br />
z<br />
y<br />
5aˆ<br />
z<br />
(b). r <br />
QP<br />
( 1<br />
2) aˆ<br />
( 3<br />
4) aˆ<br />
(5 6) aˆ<br />
aˆ<br />
7 aˆ<br />
aˆ<br />
x<br />
y<br />
z<br />
x<br />
y<br />
z<br />
(c).<br />
2<br />
2<br />
2<br />
QR (0 2) (3 4) (8 6) 4 1<br />
4 3unit<br />
Example(12):<br />
Derive the cosine formula<br />
c<br />
2<br />
2 2<br />
a b 2abcos<br />
<strong>and</strong> the sine formula<br />
sin A sin B sin C<br />
using dot <strong>and</strong> cross product respectively.<br />
a b c<br />
b<br />
C<br />
Cosine formula:<br />
<br />
c a<br />
b<br />
<br />
c c (<br />
a b)<br />
(<br />
a b)<br />
a<br />
c<br />
2<br />
a<br />
2<br />
b<br />
2<br />
2<br />
b<br />
2<br />
A<br />
c<br />
2a<br />
b<br />
2 2<br />
2ab<br />
cos c a b 2abcos<br />
a<br />
B<br />
Sine formula:<br />
1 1 <br />
a b b c<br />
2 2<br />
<br />
1<br />
2<br />
a bsin<br />
c <br />
1<br />
2<br />
1 <br />
c a<br />
2<br />
b c sin a <br />
1<br />
2<br />
c a<br />
sin b<br />
dividing<br />
the above equation<br />
by<br />
( a b c ) we get :<br />
sin c<br />
c<br />
<br />
sin a<br />
a<br />
<br />
sin b<br />
b<br />
Example(13):<br />
<br />
<br />
Let E 3aˆ<br />
4aˆ<br />
<strong>and</strong> F 4aˆ<br />
10aˆ<br />
5aˆ<br />
, then find:<br />
x<br />
z<br />
x<br />
y<br />
z<br />
(a). the component <strong>of</strong><br />
E along<br />
F<br />
(b). A unit <strong>vector</strong> perpendicular to both<br />
E <strong>and</strong><br />
F<br />
Solution:<br />
(a). the <strong>vector</strong> component <strong>of</strong><br />
E along F is given by:<br />
63
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<br />
E<br />
F<br />
E cos<br />
aˆ<br />
F<br />
<br />
E F<br />
<br />
F<br />
<br />
( E aˆ<br />
) aˆ<br />
4 aˆ<br />
ˆ ˆ ˆ ˆ ˆ<br />
x<br />
10<br />
a<br />
y<br />
5 a<br />
z<br />
4 a<br />
x<br />
10<br />
a<br />
y<br />
5 a<br />
z<br />
aˆ<br />
F<br />
<br />
<br />
16 100 25<br />
141<br />
<br />
4 aˆ<br />
10 ˆ 5 ˆ<br />
x<br />
a<br />
y<br />
a<br />
z<br />
( E aˆ<br />
) aˆ<br />
(3aˆ<br />
4 ˆ<br />
F F<br />
x<br />
a<br />
z<br />
) <br />
<br />
141<br />
(12 20)<br />
E (4 aˆ<br />
10 ˆ 5 ˆ<br />
F<br />
x<br />
a<br />
y<br />
a<br />
z<br />
)<br />
141<br />
aˆ<br />
F<br />
F<br />
F<br />
<br />
<br />
<br />
4 aˆ<br />
x<br />
10<br />
aˆ<br />
y<br />
141<br />
5 aˆ<br />
z<br />
<strong>The</strong> scalar component <strong>of</strong> E along F is given by:<br />
<br />
<br />
E F <br />
E<br />
ˆ<br />
F<br />
E cos<br />
E aF<br />
F<br />
<br />
4 aˆ<br />
10<br />
ˆ 5 ˆ<br />
x<br />
a<br />
y<br />
az<br />
12 20<br />
E aˆ<br />
(3aˆ<br />
4 ˆ<br />
F<br />
E<br />
F<br />
x<br />
a<br />
z<br />
) <br />
<br />
<br />
141 141<br />
Example(14):<br />
<br />
Three field quantities are given by: P aˆ<br />
aˆ<br />
,<br />
<br />
Q 2aˆ<br />
aˆ<br />
2aˆ<br />
,<br />
2<br />
x z<br />
x y z<br />
R 2aˆ<br />
3aˆ<br />
aˆ<br />
,<br />
x<br />
y<br />
z<br />
determine the following:<br />
<br />
(a). ( P Q)<br />
( P Q)<br />
P ( P Q)<br />
Q<br />
( P Q)<br />
,<br />
<br />
(b). Q ( R P)<br />
(c). sin QR<br />
<br />
(d). P ( Q R)<br />
<br />
(e). A unit <strong>vector</strong> perpendicular to both Q <strong>and</strong> R<br />
<br />
(f). <strong>The</strong> scalar <strong>and</strong> <strong>vector</strong> component <strong>of</strong> P along<br />
<br />
Q<br />
Solution:<br />
<br />
( P Q)<br />
( P Q)<br />
P ( P Q)<br />
Q<br />
( P Q)<br />
P P P Q Q<br />
P Q<br />
Q<br />
<br />
0<br />
Q P Q<br />
P 0<br />
2 P Q sinQP<br />
<br />
<br />
(a). P 4 1<br />
5 Q 4 1<br />
4 3<br />
<br />
1<br />
Q P 4 2 2<br />
<br />
QP<br />
cos<br />
72.65<br />
P Q 3<br />
5 3 5<br />
sin 72.65 0.95<br />
64
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
2 1<br />
2<br />
<br />
(b). Q ( R P)<br />
2 3 1 2<br />
(3 0) 1<br />
(2 2) 2<br />
(0 6) 6 4 12<br />
14<br />
2 0 1<br />
(c).<br />
sin<br />
QR<br />
<br />
Q R<br />
<br />
aˆ<br />
x<br />
Q R <br />
Q R 2<br />
Q R<br />
2<br />
25 4 16<br />
45<br />
aˆ<br />
y<br />
aˆ<br />
z<br />
1<br />
2<br />
3 1<br />
,<br />
<br />
Q <br />
aˆ<br />
( 1<br />
6) aˆ<br />
(4 2) aˆ<br />
( 6<br />
2) 5aˆ<br />
2aˆ<br />
x<br />
4 1<br />
4 3<br />
<strong>and</strong><br />
y<br />
z<br />
<br />
R 4 9 1<br />
14<br />
x<br />
y<br />
4aˆ<br />
z<br />
sin<br />
QR<br />
<br />
3<br />
45<br />
14<br />
0.596<br />
(d).<br />
aˆ<br />
x<br />
aˆ<br />
y<br />
aˆ<br />
z<br />
<br />
P ( Q R)<br />
2 0 1<br />
aˆ<br />
(0 2) aˆ<br />
( 5<br />
8) aˆ<br />
(4 0) 2aˆ<br />
3aˆ<br />
4aˆ<br />
5 2 4<br />
x<br />
y<br />
z<br />
x<br />
y<br />
z<br />
(e).<br />
aˆ<br />
QR<br />
<br />
Q R<br />
<br />
<br />
Q R<br />
<br />
5aˆ<br />
x<br />
2 aˆ<br />
y<br />
3 14<br />
4 aˆ<br />
z<br />
<br />
(f). <strong>The</strong> scalar <strong>and</strong> <strong>vector</strong> component P along Q can be calculated as follows:<br />
<br />
<strong>The</strong> scalar component <strong>of</strong> P along Q is equal to:<br />
<br />
<br />
P Q <br />
P<br />
ˆ<br />
Q<br />
P cos<br />
P aQ<br />
Q<br />
2 aˆ<br />
ˆ 2 ˆ<br />
x<br />
a<br />
y<br />
a<br />
z 4 2 2<br />
P aˆ<br />
(2<br />
aˆ<br />
ˆ<br />
Q<br />
x<br />
a<br />
z<br />
) <br />
<br />
<br />
3 3 3<br />
<br />
<strong>The</strong> <strong>vector</strong> component <strong>of</strong> P along Q is given by:<br />
65
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<br />
P<br />
Q<br />
E cos<br />
aˆ<br />
F<br />
<br />
P Q<br />
<br />
Q<br />
aˆ<br />
Q<br />
<br />
(<br />
P aˆ<br />
) aˆ<br />
2aˆ<br />
ˆ ˆ<br />
x<br />
a<br />
y<br />
2 az<br />
aˆ<br />
Q<br />
<br />
3<br />
2 aˆ<br />
ˆ 2 ˆ 2 ˆ ˆ 2 ˆ<br />
x<br />
a<br />
y<br />
az<br />
ax<br />
a<br />
y<br />
a<br />
( P aˆ<br />
) ˆ (2 ˆ ˆ<br />
Q<br />
aQ<br />
a<br />
x<br />
az<br />
) <br />
<br />
<br />
3 3<br />
4 2<br />
2<br />
P (2aˆ<br />
ˆ 2 ˆ ) (2 ˆ ˆ 2 ˆ<br />
Q<br />
x<br />
a<br />
y<br />
a<br />
z<br />
ax<br />
a<br />
y<br />
az<br />
)<br />
9<br />
9<br />
Q<br />
Q<br />
z<br />
Example(15):<br />
Find the distance between the following pairs <strong>of</strong> points:<br />
a‐ P 1 (1,1,2) <strong>and</strong> P 2 (0,2,2)<br />
<br />
<br />
b‐ P 1 (2, ,1) <strong>and</strong> P2<br />
(4, ,0)<br />
,<br />
3<br />
2<br />
<br />
<br />
c‐ P<br />
1( 3, , ) <strong>and</strong> P2<br />
(4, , ) .<br />
2<br />
2<br />
Solution:<br />
(a).<br />
2<br />
2<br />
2<br />
P P (1 0) (2 2) (1 2) 2<br />
1 2<br />
<br />
unit<br />
(b).<br />
x<br />
x<br />
p<br />
p1<br />
p2<br />
1<br />
p<br />
cos<br />
2 cos60 1<br />
cos<br />
4 cos90 0<br />
2<br />
<br />
1<br />
2<br />
1<br />
2<br />
(1 0)<br />
2<br />
(1.73 4)<br />
2<br />
y<br />
(1 0)<br />
p1<br />
y<br />
2<br />
sin<br />
2sin 60 1.73<br />
p2<br />
1<br />
sin<br />
4sin 90 4<br />
2<br />
1<br />
2.06 unit<br />
2<br />
z<br />
p1<br />
z<br />
1<br />
p2<br />
0<br />
(c).<br />
x<br />
x<br />
p1<br />
p2<br />
p p<br />
1<br />
r sin<br />
cos<br />
3sin<br />
cos90 0<br />
r<br />
2<br />
1<br />
2<br />
<br />
1<br />
sin<br />
cos<br />
4sin 90cos<br />
4<br />
2<br />
1<br />
2<br />
(0 ( 4))<br />
2<br />
(0 0)<br />
2<br />
( 3<br />
0)<br />
2<br />
y<br />
p1<br />
y<br />
r sin<br />
sin<br />
2sin<br />
sin 60 0<br />
p2<br />
1<br />
r<br />
2<br />
sin<br />
sin<br />
4sin 90sin<br />
0<br />
16 9 5 unit<br />
1<br />
2<br />
1<br />
2<br />
z<br />
p1<br />
r cos<br />
3cos<br />
3<br />
z<br />
1<br />
p2<br />
r<br />
2<br />
1<br />
cos<br />
4cos90 0<br />
2<br />
Example(16):<br />
Convert the coordinates <strong>of</strong> the following points from Cartesian to cylindrical <strong>and</strong> spherical<br />
coordinates : a‐ P 1 (1,2,0) , b‐ P 2 (0,0,3) <strong>and</strong> c‐ P 3 (1,1,2)<br />
66
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Solution:<br />
In general the Cartesian coordinates are related to cylindrical <strong>and</strong> spherical coordinates by<br />
the following equations:<br />
<br />
r <br />
x<br />
x<br />
2<br />
2<br />
y<br />
y<br />
2<br />
2<br />
z<br />
2<br />
tan<br />
1<br />
tan<br />
y<br />
x<br />
1<br />
y<br />
x<br />
z z<br />
cos<br />
<strong>The</strong>refore these points in cylindrical coordinates are given as follows:<br />
1<br />
z<br />
r<br />
x<br />
(a).<br />
<br />
P1<br />
P1<br />
1<br />
x<br />
2<br />
P1<br />
y<br />
y<br />
P1<br />
2<br />
P1<br />
2<br />
5<br />
<br />
P1<br />
<strong>and</strong><br />
tan<br />
1<br />
y<br />
x<br />
P1<br />
P1<br />
z<br />
P1<br />
0<br />
tan<br />
1<br />
2 <br />
63<br />
1<br />
<strong>and</strong><br />
z<br />
P1<br />
0<br />
P 1(<br />
<br />
5,63 ,0)<br />
x<br />
P2<br />
(b).<br />
<br />
0<br />
P2<br />
<br />
x<br />
2<br />
P2<br />
y<br />
y<br />
P2<br />
2<br />
P2<br />
0<br />
0<br />
<br />
P2<br />
<strong>and</strong><br />
tan<br />
1<br />
y<br />
x<br />
P2<br />
P2<br />
z<br />
P2<br />
3<br />
tan<br />
1<br />
0 <br />
90<br />
0<br />
<strong>and</strong><br />
z<br />
P2<br />
3<br />
P<br />
2<br />
(0,90<br />
<br />
,3)<br />
x<br />
P3<br />
(c).<br />
<br />
1<br />
P3<br />
<br />
x<br />
2<br />
P3<br />
y<br />
P3<br />
y<br />
1<br />
2<br />
P3<br />
<br />
2<br />
<br />
<strong>and</strong><br />
P3<br />
tan<br />
1<br />
z<br />
y<br />
x<br />
P3<br />
P3<br />
P3<br />
2<br />
tan<br />
1<br />
1<br />
45<br />
1<br />
<br />
<strong>and</strong><br />
z<br />
P3<br />
2<br />
P 3(<br />
<br />
2,45 ,2)<br />
Also these points in spherical coordinates are given as follows:<br />
(a).<br />
x<br />
r<br />
P1<br />
P1<br />
1<br />
<br />
x<br />
2<br />
P1<br />
y<br />
y<br />
2<br />
P1<br />
P1<br />
z<br />
2<br />
2<br />
P1<br />
<br />
5<br />
<strong>and</strong><br />
tan<br />
P1<br />
1<br />
y<br />
x<br />
z<br />
P1<br />
P1<br />
P1<br />
0<br />
tan<br />
1<br />
2 <br />
63<br />
1<br />
<strong>and</strong><br />
<br />
P1<br />
cos<br />
1<br />
0<br />
90<br />
5<br />
<br />
P 1(<br />
<br />
5,63 ,90<br />
<br />
)<br />
xP2<br />
0<br />
(b).<br />
<br />
P2<br />
x<br />
2<br />
P2<br />
y<br />
y<br />
P2<br />
2<br />
P2<br />
0<br />
z<br />
2<br />
P2<br />
3<br />
<strong>and</strong><br />
<br />
P2<br />
z<br />
tan<br />
P2<br />
1<br />
3<br />
y<br />
x<br />
P2<br />
P2<br />
tan<br />
1<br />
0 <br />
90<br />
0<br />
<strong>and</strong><br />
<br />
P2<br />
cos<br />
1<br />
3 <br />
0<br />
3<br />
P<br />
2<br />
(3,90<br />
<br />
,0<br />
<br />
)<br />
67
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
(c).<br />
x<br />
P3<br />
<br />
1<br />
P3<br />
<br />
x<br />
2<br />
P3<br />
y<br />
P3<br />
y<br />
1<br />
2<br />
P3<br />
z<br />
2<br />
P3<br />
<br />
6<br />
<strong>and</strong><br />
<br />
P3<br />
z<br />
P3<br />
tan<br />
2<br />
1<br />
y<br />
x<br />
P3<br />
P3<br />
tan<br />
1<br />
1<br />
45<br />
1<br />
<br />
<strong>and</strong><br />
<br />
P3<br />
cos<br />
1<br />
2<br />
35.26<br />
6<br />
P<br />
3 (<br />
<br />
6,45 ,35.26 )<br />
Example(17):<br />
Express <strong>vector</strong> <br />
in Cartesian coordinates.<br />
Solution:<br />
<br />
<br />
<br />
<br />
0<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
1<br />
But: ; <br />
<br />
sin <br />
cos <br />
<br />
sin <br />
<br />
cos <br />
<br />
<br />
<br />
<br />
; tan <br />
<br />
10 <br />
∙ <br />
<br />
<br />
<br />
<br />
10 <br />
∙ <br />
<br />
<br />
<br />
10<br />
<br />
<br />
<br />
<br />
Example(18):<br />
If ∅ evaluate ∮ . around the path shown in Figure:<br />
Solution:<br />
<br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
1‐ ∙ <br />
<br />
<br />
<br />
2| √3 1<br />
68
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
<br />
<br />
<br />
<br />
2‐ ∙ 30 <br />
<br />
<br />
3‐ ∙ <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
5| <br />
4‐ ∙ 60 <br />
<br />
<br />
√<br />
<br />
√3 1<br />
<br />
<br />
<br />
∙ √3 1 21√3<br />
4 5√3<br />
2 5 2 21<br />
4 27 √3 1<br />
4<br />
Example(19):<br />
Show that the scalar field 10 is not harmonic.<br />
Solution:<br />
10 <br />
1 <br />
<br />
1<br />
<br />
<br />
<br />
1 <br />
10 1<br />
<br />
20 <br />
<br />
202<br />
<br />
<br />
<br />
1<br />
<br />
<br />
10 2 22 2 1<br />
<br />
10 1 22<br />
<br />
∎ T0 <strong>The</strong> function is not harmonic.<br />
<br />
102 10 <br />
<br />
102<br />
<br />
10<br />
<br />
Example(20):<br />
Obtain the spherical coordinates <strong>of</strong> 10 at the point 3, 2, 4.<br />
Solution:<br />
Giving <strong>vector</strong> is in Cartesian system say 10 <br />
∙ 10 ∙ 10sincos<br />
∙ 10 ∙ 10coscos<br />
∙ 10 ∙ 10sin<br />
3 2 4 √29<br />
tan tan 2 3 33.69<br />
cos cos 4<br />
√29 42.03<br />
69
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
But, is negative <strong>and</strong> is positive hence must be between 90 <strong>and</strong> 180 , so add 180 to<br />
:<br />
33.69 180 146.31 <br />
10sin42.03 cos146.31<br />
10cos42.03 cos146.31<br />
10sin146.31<br />
5.57 6.18 5.547 <br />
Example(21):<br />
A particular <strong>vector</strong> field cos sin , is in the cylindrical system. Find the<br />
flux due to this field over a close surface <strong>of</strong> the cylinder 4 , 01 , verify the<br />
divergence theorem.<br />
Solution:<br />
z<br />
(1)<br />
(3)<br />
(2)<br />
y<br />
Surface (1):<br />
Surface (2):<br />
Surface (3):<br />
∙ cos sin ∙ 0<br />
∙ cos sin ∙ 0<br />
∙ cos sin ∙ <br />
<br />
cos <br />
<br />
<br />
<br />
4 1cos2 <br />
<br />
sin 2<br />
32 <br />
2 64<br />
<br />
<strong>The</strong> divergence theorem state:<br />
∙ ∙ <br />
∙ 1 <br />
1 <br />
x<br />
<br />
<br />
<br />
1 <br />
<br />
cos 1 <br />
<br />
<br />
<br />
<br />
sin 0<br />
<br />
70
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
3cos cos <br />
∙ 3 cos cos <br />
<br />
:<br />
<br />
<br />
<br />
<br />
3 cos cos <br />
<br />
<br />
cos cos <br />
<br />
<br />
<br />
<br />
<br />
64 cos 4cos <br />
<br />
<br />
64 cos 2 cos <br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
<br />
64 cos 2cos <br />
321cos2 2cos <br />
<br />
<br />
sin 2<br />
32 2sin 64<br />
2<br />
<br />
∙ ∙ & .<br />
Example(22):<br />
Given that calculate the circulation <strong>of</strong> around the closed path<br />
shown in Figure.<br />
Solution:<br />
Line (1):<br />
∙ <br />
<br />
<br />
0 , 0 , <br />
∙ <br />
<br />
<br />
∙ <br />
71
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Line (2):<br />
Line (3):<br />
Line (4):<br />
<br />
∙ <br />
<br />
<br />
<br />
3 1 3<br />
<br />
0 , 0 , <br />
∙ 0<br />
∙ 0<br />
1 , , <br />
∙ <br />
<br />
∙ 1<br />
<br />
<br />
<br />
3 2 3<br />
<br />
1 , , <br />
∙ <br />
<br />
∙ <br />
<br />
<br />
2 <br />
<br />
3 5 6<br />
<br />
∙ 1 2 02 3 5 6 1 6<br />
Example(23):<br />
For an harmonic scalar function sin 4 cos 3. Find <strong>and</strong> .<br />
Solution:<br />
<strong>The</strong> curl <strong>of</strong> grad is always zero:<br />
Since the function is harmonic:<br />
∴ 0<br />
∴ 0<br />
Example(24):<br />
<br />
transform into spherical coordinate <strong>and</strong> find its magnitude at point 3, 4, 0.<br />
∙ ∙ ∙ ∙ <br />
∙ ∙ ∙ ∙ <br />
∙ ∙ ∙ ∙ <br />
<br />
∙ ∙ ∙ <br />
∙ ∙ ∙ <br />
∙ ∙ ∙ <br />
<br />
72
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
0 <br />
0 <br />
<br />
0 1 0 <br />
<br />
<br />
<br />
<br />
<br />
<br />
√9165<br />
√25 5<br />
0 5 90<br />
4<br />
3 53.13<br />
5sin90 cos53.13 5 0 cos90 sin53.13 <br />
5cos90 cos53.13 5 0 sin 90 sin 53.13 . <br />
3 0 0.018 <br />
Example(25):<br />
Show that if the scalar function , is harmonic at point √3, ,0 .<br />
∂<br />
r sinθ ∂θ<br />
H 1 ∂<br />
r ∂r r sin θ e 1<br />
H 1 ∂ ∂H<br />
r r<br />
∂r ∂r 1<br />
∂H<br />
sinθ<br />
∂θ 1<br />
∂<br />
∂ H<br />
r sin θ ∂φ <br />
r sinθ ∂θ sinθ2 sin θ cosθ e <br />
H sin θ<br />
r r e 2r e 2 e<br />
r sinθ sin θ2sinθ cos θ <br />
H sin θ<br />
r<br />
e r2 2 e<br />
r 2 cos θ sin θ<br />
At point √3, ,0, H0 ⟹ is not harmonic.<br />
1<br />
r sin θ 0<br />
Home Work:<br />
<br />
<br />
Q 1 / Prove that: ( V A ) V<br />
A AV<br />
, where V is a scalar field <strong>and</strong> A is a <strong>vector</strong> field ?<br />
73
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
Q 2 / prove that:<br />
<br />
( V A)<br />
V<br />
A V<br />
A , where V is a scalar field <strong>and</strong> A is a <strong>vector</strong><br />
field?<br />
Q 3 / Show that:<br />
Q 4 / Given <strong><strong>vector</strong>s</strong><br />
<br />
<br />
<br />
2<br />
2<br />
( A B)<br />
( A B)<br />
( A B<br />
<br />
A<br />
aˆ<br />
x<br />
2aˆ<br />
y<br />
<br />
3aˆ<br />
z<br />
2<br />
) .<br />
<br />
, B 3aˆ<br />
x<br />
4aˆ<br />
y<br />
<strong>and</strong><br />
<br />
C 3aˆ<br />
y<br />
4aˆ<br />
z<br />
, find the<br />
following:<br />
<br />
<br />
a. A <strong>and</strong> aˆ<br />
A<br />
, b. the component <strong>of</strong> B along C , c. <br />
AC<br />
, d. A<br />
C<br />
<br />
,<br />
<br />
<br />
<br />
e. A ( B C)<br />
, f. A ( B C)<br />
, g. aˆ x<br />
B , h. ( A<br />
aˆ<br />
y<br />
). aˆ<br />
z<br />
.<br />
Q 5 / Express the unit <strong>vector</strong> directed toward the point ( 0,0, h ) from an arbitrary point in the<br />
plane z 2<br />
. Explain the result as h 2<br />
?<br />
x aˆ<br />
ˆ<br />
x<br />
y a<br />
y<br />
Ans.<br />
( h 2) aˆ<br />
z<br />
2 2<br />
2<br />
x y ( h 2)<br />
<br />
Q 6 / Given A 4aˆ<br />
10aˆ<br />
<strong>and</strong><br />
<br />
B 2aˆ<br />
3aˆ<br />
, find the magnitude <strong>and</strong> <strong>vector</strong><br />
y<br />
z<br />
y<br />
x<br />
<br />
components <strong>of</strong> A on B ?<br />
<br />
<br />
Q 7 / Find the angle between A 10aˆ<br />
2aˆ<br />
, <strong>and</strong> B 4aˆ<br />
0.5aˆ<br />
, using both dot <strong>and</strong><br />
cross product.<br />
<br />
Q 8 / Given A aˆ<br />
aˆ<br />
x<br />
y<br />
,<br />
<br />
B 2aˆ<br />
z<br />
<strong>and</strong><br />
z<br />
y<br />
<br />
C aˆ<br />
x<br />
3aˆ<br />
y<br />
y<br />
, find both<br />
<br />
A B C <strong>and</strong> ( A B)<br />
C ? Ans. 4 , 8<br />
âz<br />
.<br />
<br />
Q 9 / Given A ( y 1)<br />
aˆ<br />
2 x aˆ<br />
, find the <strong>vector</strong> at point ( 2,2,1)<br />
<strong>and</strong> its projection on <strong>vector</strong><br />
Q 10 / If<br />
B <br />
, where B 5aˆ<br />
aˆ<br />
2aˆ<br />
?<br />
x<br />
x<br />
x<br />
y<br />
<br />
F F x<br />
aˆ<br />
, what can be said about F if ;<br />
1. F 0 every where ,<br />
2. F 0 every where ,<br />
y<br />
z<br />
z<br />
3. F 0 <strong>and</strong> F 0 every where?<br />
<br />
2<br />
3<br />
3<br />
Q 11 / Given field A 3 x y z aˆ<br />
x z aˆ<br />
( x y 2 z)<br />
aˆ<br />
x<br />
y<br />
z<br />
, it can be said that A is :<br />
a. Harmonic, b. Divergenceless, c. Solenoid, d. Rotational, e. Conservative.<br />
Q 12 / If a <strong>vector</strong> field A is solenoid, which <strong>of</strong> the following is true:<br />
<br />
a. A dl 0 , b. A ds 0 , c. A 0 , d. A 2<br />
0 , e. A 0 .<br />
L<br />
<br />
S<br />
Q 13 / If ( r <br />
) is the position <strong>vector</strong> <strong>of</strong> point (x,y,z) <strong>and</strong> r r , then prove that :<br />
74
Electromagnetic <strong>The</strong>orem<br />
(Dr. Omed Ghareb Abdullah) University <strong>of</strong> Sulaimani –College <strong>of</strong> Science – Physics Department<br />
aˆr<br />
a. (ln r)<br />
<strong>and</strong> b. <br />
2 (ln r)<br />
<br />
1<br />
2<br />
r<br />
r<br />
<br />
3 2 2<br />
Q 14 / Let G x y z aˆ<br />
, then calculate: a. G <br />
, b. G <br />
, c. G <br />
, d.<br />
G x<br />
x<br />
Q 15 / Evaluate<br />
V , V<br />
, <strong>and</strong> V<br />
if :<br />
Q 16 / If<br />
2<br />
a. V 3 x y x z , b. V z cos<br />
, c. V 4r<br />
2 cos sin<br />
.<br />
<br />
r x aˆ<br />
x<br />
y aˆ<br />
y<br />
z aˆ<br />
z<br />
<br />
is the position <strong>vector</strong> <strong>of</strong> point (x,y,z) <strong>and</strong> r r , then which <strong>of</strong><br />
<br />
r<br />
the following is incorrect: a. r , b. r 1 , c. <br />
2 ( r r<br />
) 6<br />
r<br />
, d. r 0 .<br />
Q 17 / Determine which <strong>of</strong> the following <strong>vector</strong> fields is harmonic, solenoid or conservative ;<br />
<br />
<br />
<br />
2<br />
2 2<br />
<br />
a. A 2 x y aˆ<br />
x<br />
y aˆ<br />
y<br />
, b. B x aˆ<br />
x<br />
y aˆ<br />
y<br />
2 z aˆ<br />
z<br />
, c. C ( 3 ) aˆ<br />
z aˆ<br />
z<br />
1<br />
<br />
1<br />
<br />
r<br />
d. D aˆ<br />
r<br />
, e. E r ar<br />
r<br />
e ˆ<br />
sin<br />
cos<br />
, f. F aˆ<br />
<br />
aˆ<br />
2 2 <br />
<br />
75