on carleman estimates for elliptic and parabolic operators ...
on carleman estimates for elliptic and parabolic operators ...
on carleman estimates for elliptic and parabolic operators ...
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
10 JÉRÔME LE ROUSSEAU AND GILLES LEBEAU<br />
S<br />
f (x) = f (x 0 )<br />
Ω ε<br />
W<br />
∇ f<br />
x 0<br />
ϕ(x) = ϕ(x 0 ) − ε<br />
∇ϕ<br />
B 0<br />
V ′′ ϕ(x) = ϕ(x 0 )<br />
V<br />
V ′<br />
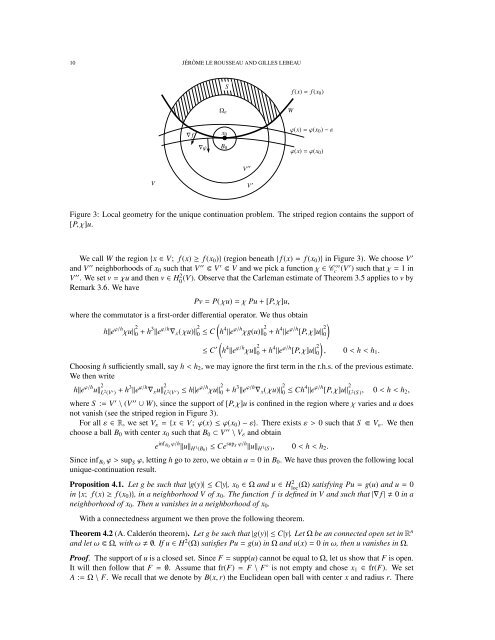
Figure 3: Local geometry <strong>for</strong> the unique c<strong>on</strong>tinuati<strong>on</strong> problem. The striped regi<strong>on</strong> c<strong>on</strong>tains the support of<br />
[P, χ]u.<br />
We call W the regi<strong>on</strong> {x ∈ V; f (x) ≥ f (x 0 )} (regi<strong>on</strong> beneath { f (x) = f (x 0 )} in Figure 3). We choose V ′<br />
<strong>and</strong> V ′′ neighborhoods of x 0 such that V ′′ ⋐ V ′ ⋐ V <strong>and</strong> we pick a functi<strong>on</strong> χ ∈ Cc ∞ (V ′ ) such that χ = 1 in<br />
V ′′ . We set v = χu <strong>and</strong> then v ∈ H0 2 (V). Observe that the Carleman estimate of Theorem 3.5 applies to v by<br />
Remark 3.6. We have<br />
Pv = P( χu) = χ Pu + [P, χ]u,<br />
where the commutator is a first-order differential operator. We thus obtain<br />
(<br />
)<br />
h‖e ϕ/h χu‖ 2 0 + h 3 ‖e ϕ/h ∇ x ( χu)‖ 2 0 ≤ C h 4 ‖e ϕ/h χg(u)‖ 2 0 + h 4 ‖e ϕ/h [P, χ]u‖ 2 0<br />
)<br />
≤ C<br />
(h ′ 4 ‖e ϕ/h χu‖ 2 0 + h 4 ‖e ϕ/h [P, χ]u‖ 2 0 , 0 < h < h 1 .<br />
Choosing h sufficiently small, say h < h 2 , we may ignore the first term in the r.h.s. of the previous estimate.<br />
We then write<br />
h‖e ϕ/h u‖ 2 L 2 (V ′ ) + h 3 ‖e ϕ/h ∇ x u‖ 2 L 2 (V ′ ) ≤ h‖e ϕ/h χu‖ 2 0 + h 3 ‖e ϕ/h ∇ x ( χu)‖ 2 0 ≤ Ch 4 ‖e ϕ/h [P, χ]u‖ 2 L 2 (S ), 0 < h < h 2 ,<br />
where S := V ′ \ (V ′′ ∪ W), since the support of [P, χ]u is c<strong>on</strong>fined in the regi<strong>on</strong> where χ varies <strong>and</strong> u does<br />
not vanish (see the striped regi<strong>on</strong> in Figure 3).<br />
For all ε ∈ R, we set V ε = {x ∈ V; ϕ(x) ≤ ϕ(x 0 ) − ε}. There exists ε > 0 such that S ⋐ V ε . We then<br />
choose a ball B 0 with center x 0 such that B 0 ⊂ V ′′ \ V ε <strong>and</strong> obtain<br />
e inf B 0 ϕ/h ‖u‖ H 1 (B 0 ) ≤ Ce sup S ϕ/h ‖u‖ H 1 (S ), 0 < h < h 2 .<br />
Since inf B0 ϕ > sup S ϕ, letting h go to zero, we obtain u = 0 in B 0 . We have thus proven the following local<br />
unique-c<strong>on</strong>tinuati<strong>on</strong> result.<br />
Propositi<strong>on</strong> 4.1. Let g be such that |g(y)| ≤ C|y|, x 0 ∈ Ω <strong>and</strong> u ∈ H 2 loc<br />
(Ω) satisfying Pu = g(u) <strong>and</strong> u = 0<br />
in {x; f (x) ≥ f (x 0 )}, in a neighborhood V of x 0 . The functi<strong>on</strong> f is defined in V <strong>and</strong> such that |∇ f | 0 in a<br />
neighborhood of x 0 . Then u vanishes in a neighborhood of x 0 .<br />
With a c<strong>on</strong>nectedness argument we then prove the following theorem.<br />
Theorem 4.2 (A. Calderón theorem). Let g be such that |g(y)| ≤ C|y|. Let Ω be an c<strong>on</strong>nected open set in R n<br />
<strong>and</strong> let ω ⋐ Ω, with ω ∅. If u ∈ H 2 (Ω) satisfies Pu = g(u) in Ω <strong>and</strong> u(x) = 0 in ω, then u vanishes in Ω.<br />
Proof. The support of u is a closed set. Since F = supp(u) cannot be equal to Ω, let us show that F is open.<br />
It will then follow that F = ∅. Assume that fr(F) = F \ F ◦ is not empty <strong>and</strong> chose x 1 ∈ fr(F). We set<br />
A := Ω \ F. We recall that we denote by B(x, r) the Euclidean open ball with center x <strong>and</strong> radius r. There