THÈSE DE DOCTORAT ELLOUMI Mounir Espaces duaux de ...

44 Dual topology of the motion groups SO(n) ⋉ R n
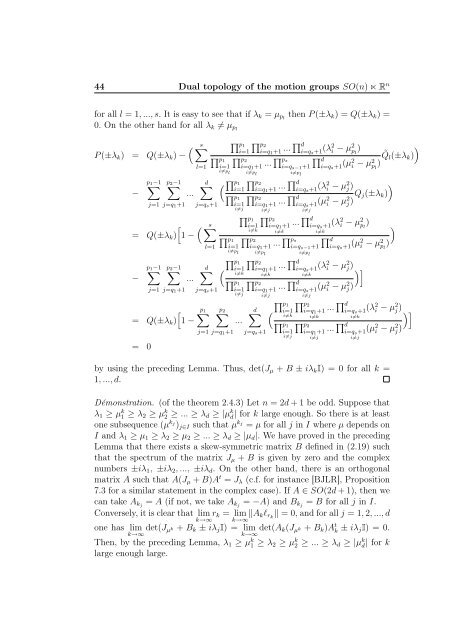
for all l = 1, ..., s. It is easy to see that if λk = µpl then P (±λk) = Q(±λk) =
0. On the other hand for all λk �= µpl
� �s
P (±λk) = Q(±λk) −
−
� � �s
= Q(±λk) 1 −
−
� � p1 p2
i=1 i=q1+1
l=1
... �d i=qs+1 (λ2i − µ 2 pl )
� � p1 p2
i=1 i=q1+1 ...
i�=pl i�=pl � � ps
d
i=qs−1+1 i=qs+1
i�=pl (µ2i − µ2pl )
p1−1 p2−1 � � d� �
...
j=1 j=q1+1 j=qs+1
� � p1 p2
i=1 i=q1+1 ... �d i=qs+1 (λ2i − µ 2 j)
� � p1 p2
i=1 i=q1+1 ...
i�=j i�=j
�d i=qs+1(µ
i�=j
2 i − µ2j )Qj(±λk)
�
� � p1 p2
i=1 i=q1+1 ...
i�=k i�=k
l=1
�d i=qs+1(λ
i�=k
2 i − µ 2 pl )
� � p1 p2
i=1 i=q1+1 ...
i�=pl i�=pl � � ps
d
i=qs−1+1 i=qs+1
i�=pl (µ2i − µ2pl )
�
� � p1 p2
p1−1 p2−1 � � d� � i=1 i=q1+1 ...
i�=k i�=k
...
j=1 j=q1+1 j=qs+1
�d i=qs+1(λ
i�=k
2 i − µ 2 j)
� � p1 p2
i=1 i=q1+1 ...
i�=j i�=j
�d i=qs+1(µ
i�=j
2 i − µ2j )
��
� � p1 p2
p1 p2 � � d� � i=1 i=q1+1 ...
i�=k i�=k
...
j=1 j=q1+1 j=qs+1
�d i=qs+1
i�=k
� � p1 p2
i=1 i=q1+1 ...
i�=j i�=j
�d i=qs+1(µ
i�=j
2 i − µ2j )
�
= Q(±λk) 1 −
= 0
�
˜Ql(±λk)
(λ2 i − µ 2 j) ��
by using the preceding Lemma. Thus, det(Jµ + B ± iλkI) = 0 for all k =
1, ..., d.
Démonstration. (of the theorem 2.4.3) Let n = 2d + 1 be odd. Suppose that
λ1 ≥ µ k 1 ≥ λ2 ≥ µ k 2 ≥ ... ≥ λd ≥ |µ k d | for k large enough. So there is at least
one subsequence (µ kj )j∈I such that µ kj = µ for all j in I where µ depends on
I and λ1 ≥ µ1 ≥ λ2 ≥ µ2 ≥ ... ≥ λd ≥ |µd|. We have proved in the preceding
Lemma that there exists a skew-symmetric matrix B defined in (2.19) such
that the spectrum of the matrix Jµ + B is given by zero and the complex
numbers ±iλ1, ±iλ2, ..., ±iλd. On the other hand, there is an orthogonal
matrix A such that A(Jµ + B)At = Jλ (c.f. for instance [BJLR], Proposition
7.3 for a similar statement in the complex case). If A ∈ SO(2d + 1), then we
can take Akj = A (if not, we take Akj = −A) and Bkj = B for all j in I.
Conversely, it is clear that lim rk = lim �Akℓrk� = 0, and for all j = 1, 2, ..., d
k→∞ k→∞
one has lim
k→∞ det(J µ k + Bk ± iλjI) = lim
k→∞ det(Ak(J µ k + Bk)A t k ± iλjI) = 0.
Then, by the preceding Lemma, λ1 ≥ µ k 1 ≥ λ2 ≥ µ k 2 ≥ ... ≥ λd ≥ |µ k d | for k
large enough large.