Dispense - Dipartimento di Matematica e Informatica

CAPITOLO 3. CURVE ALGEBRICHE PIANE 46
3.3 Risultante di due polinomi
Ricordiamo rapidamente la definizione e le principali proprietà del risultante di due
polinomi.
Sia D un dominio a fattorizzazione unica, e sia D[T ] l’anello dei polinomi su D. Siano
f(T ) = a0 +a1T +. . .+anT n e g(T ) = b0 +b1T +. . .+bmT m due polinomi a coefficienti
in D di grado rispettivo n e m (ovvero supponiamo che anbm = 0). Si definisce
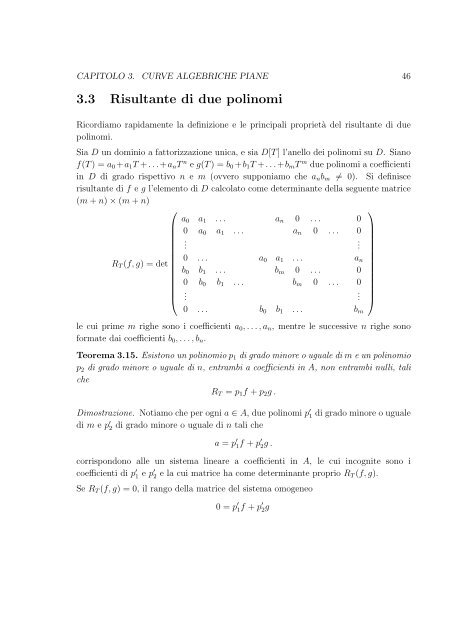
risultante di f e g l’elemento di D calcolato come determinante della seguente matrice
(m + n) × (m + n)
⎛
a0
⎜ 0
⎜ .
⎜ 0
RT (f, g) = det ⎜ b0
⎜
0
⎜
⎝ .
a1
a0
. . .
b1
b0
. . .
a1
. . .
b1
. . .
. . .
a0
an
a1
bm
0
an
. . .
0
bm
. . .
0
. . .
0
. . .
. . .
0
0
.
an
0
0
.
⎞
⎟
⎠
0 . . . b0 b1 . . . bm
le cui prime m righe sono i coefficienti a0, . . . , an, mentre le successive n righe sono
formate dai coefficienti b0, . . . , bn.
Teorema 3.15. Esistono un polinomio p1 di grado minore o uguale di m e un polinomio
p2 di grado minore o uguale di n, entrambi a coefficienti in A, non entrambi nulli, tali
che
RT = p1f + p2g .
Dimostrazione. Notiamo che per ogni a ∈ A, due polinomi p ′ 1 di grado minore o uguale
di m e p ′ 2 di grado minore o uguale di n tali che
a = p ′ 1f + p ′ 2g .
corrispondono alle un sistema lineare a coefficienti in A, le cui incognite sono i
coefficienti di p ′ 1 e p ′ 2 e la cui matrice ha come determinante proprio RT (f, g).
Se RT (f, g) = 0, il rango della matrice del sistema omogeneo
0 = p ′ 1f + p ′ 2g