Chapter 6 Partial Differential Equations
Chapter 6 Partial Differential Equations
Chapter 6 Partial Differential Equations
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
6.1. INTRODUCTION 7<br />
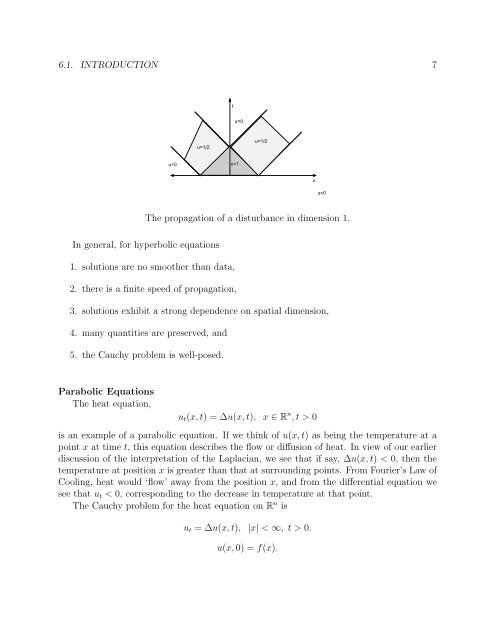
t<br />
u=0<br />
u=1/2<br />
u=1/2<br />
u=0 u=1<br />
x<br />
u=0<br />
The propagation of a disturbance in dimension 1.<br />
In general, for hyperbolic equations<br />
1. solutions are no smoother than data,<br />
2. there is a finite speed of propagation,<br />
3. solutions exhibit a strong dependence on spatial dimension,<br />
4. many quantities are preserved, and<br />
5. the Cauchy problem is well-posed.<br />
Parabolic <strong>Equations</strong><br />
The heat equation,<br />
u t (x, t) =∆u(x, t), x ∈ R n ,t>0<br />
is an example of a parabolic equation. If we think of u(x, t) as being the temperature at a<br />
point x at time t, this equation describes the flow or diffusion of heat. In view of our earlier<br />
discussion of the interpretation of the Laplacian, we see that if say, ∆u(x, t) < 0, then the<br />
temperature at position x is greater than that at surrounding points. From Fourier’s Law of<br />
Cooling, heat would ‘flow’ away from the position x, and from the differential equation we<br />
see that u t < 0, corresponding to the decrease in temperature at that point.<br />
The Cauchy problem for the heat equation on R n is<br />
u t =∆u(x, t), |x| < ∞, t>0.<br />
u(x, 0) = f(x).