Analysis for buckling and vibrations of composite ... - ResearchGate

More documents

Recommendations

Info

362 R. Rikards et al. / Composite Structures 51 (2001) 361±370 There is extensive literature for analysis of sti€ened plates and shells. For example, review and analysis of buckling problems of sti€ened plates are presented in [16]. The eciency of the ®nite element method in comparison with other methods for analysis of sti€ened structures was shown. In the present paper, the equivalent layer theory based on the ®rst-order shear deformation theory including extension of normal line (FOSDT-6) is employed in order to develop the ®nite element for analysis of laminated sti€ened shells. Previously, similar ®nite element was developed and applied for analysis of smooth shells [17,18]. 2. Kinematics of shell Kinematic equations for the ®rst-order shear deformation theory (FOSDT) including extension of the normal line can be obtained from the 3D equations of the theory of elasticity by using a well-known ®rst-order approximation of a vector function with respect to the coordinate x 3 . Further a well-known geometric relations for the shell in normal coordinates (see, for example, [14]) are used. 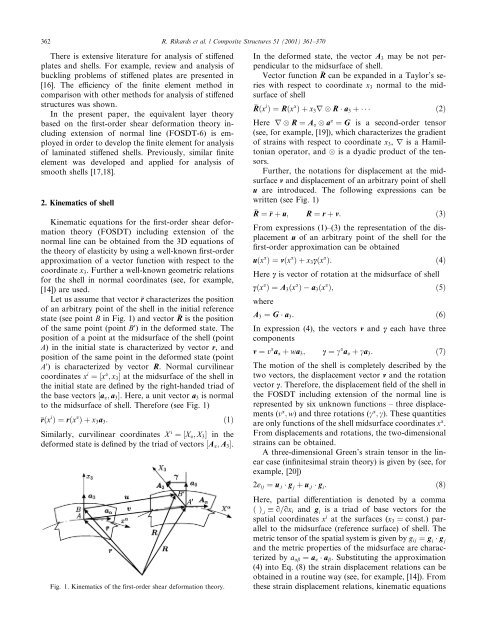Let us assume that vector r characterizes the position of an arbitrary point of the shell in the initial reference state (see point B in Fig. 1) and vector R is the position of the same point (point B 0 ) in the deformed state. The position of a point at the midsurface of the shell (point A) in the initial state is characterized by vector r, and position of the same point in the deformed state (point A 0 ) is characterized by vector R. Normal curvilinear coordinates x i ˆ‰x a ; x 3 Š at the midsurface of the shell in the initial state are de®ned by the right-handed triad of the base vectors ‰a a ; a 3 Š. Here, a unit vector a 3 is normal to the midsurface of shell. Therefore (see Fig. 1) r…x i †ˆr…x a †‡x 3 a 3 : …1† Similarly, curvilinear coordinates X i ˆ‰X a ; X 3 Š in the deformed state is de®ned by the triad of vectors ‰A a ; A 3 Š. Fig. 1. Kinematics of the ®rst-order shear deformation theory. In the deformed state, the vector A 3 may be not perpendicular to the midsurface of shell. Vector function R can be expanded in a Taylor's series with respect to coordinate x 3 normal to the midsurface of shell R…x i †ˆR…x a †‡x 3 rR a 3 ‡ …2† Here rR ˆ A a a a ˆ G is a second-order tensor (see, for example, [19]), which characterizes the gradient of strains with respect to coordinate x 3 , r is a Hamiltonian operator, and is a dyadic product of the tensors. Further, the notations for displacement at the midsurface v and displacement of an arbitrary point of shell u are introduced. The following expressions can be written (see Fig. 1) R ˆ r ‡ u; R ˆ r ‡ v: …3† From expressions (1)±(3) the representation of the displacement u of an arbitrary point of the shell for the ®rst-order approximation can be obtained u…x a †ˆv…x a †‡x 3 c…x a †: …4† Here c is vector of rotation at the midsurface of shell c…x a †ˆA 3 …x a †a 3 …x a †; …5† where A 3 ˆ G a 3 : …6† In expression (4), the vectors v and c each have three components v ˆ v a a a ‡ wa 3 ; c ˆ c a a a ‡ ca 3 : …7† The motion of the shell is completely described by the two vectors, the displacement vector v and the rotation vector c. Therefore, the displacement ®eld of the shell in the FOSDT including extension of the normal line is represented by six unknown functions ± three displacements (v a ; w) and three rotations (c a ; c). These quantities are only functions of the shell midsurface coordinates x a . From displacements and rotations, the two-dimensional strains can be obtained. A three-dimensional Green's strain tensor in the linear case (in®nitesimal strain theory) is given by (see, for example, [20]) 2e ij ˆ u ;i g j ‡ u ;j g i : …8† Here, partial di€erentiation is denoted by a comma …† ;i o=ox i and g i is a triad of base vectors for the spatial coordinates x i at the surfaces (x 3 ˆ const:) parallel to the midsurface (reference surface) of shell. The metric tensor of the spatial system is given by g ij ˆ g i g j and the metric properties of the midsurface are characterized by a ab ˆ a a a b . Substituting the approximation (4) into Eq. (8) the strain displacement relations can be obtained in a routine way (see, for example, [14]). From these strain displacement relations, kinematic equations
in di€erent curvilinear coordinates can be obtained and written through the physical components in the matrix form ˆ Lw: …9† Here w is physical components of vectors of displacement v and rotation c w T ˆ‰u; v; w; c …1† ; c …2† ; cŠ: R. Rikards et al. / Composite Structures 51 (2001) 361±370 363 …10† The shell theory outlined above is the so-called ®rstorder shear deformation theory with six degrees of freedom (FOSDT-6). Note, that in the present theory the normal line undergoes a small rotation relative to the reference surface and also a small, uniform extension. The third component of the vector c, i.e., the function c ˆ c…x a † characterizes the deformation in the direction of normal line. Often this deformation is neglected c ˆ 0. Thus, the ®rst-order shear deformation theory with ®ve degrees of freedom (FOSDT-5) can be obtained. In the case of ¯exural deformation of plates with symmetric layer stacking sequence the in-plane displacements u ˆ 0 and v ˆ 0. Therefore, for analysis of such plates the ®rst-order shear deformation theory with three degrees of freedom (FOSDT-3) can be employed. 3. Strain energy and sti€ness of laminated shell The strain energy U of the shell represented as a three-dimensional body is given by the expression, where in curvilinear coordinates the contravariant stress tensor r ij is contracted with the covariant strain tensor e ij U ˆ 1 Z r ij e ij dV ; 2 V where dV is a volume element of the shell dV ˆ dA ˆ p g dx 1 dx 2 dx 3 ˆ p a dx 1 dx 2 : r g dx 3 dA; a …11† …12† Here dA is the shell midsurface element and a determinants of the metric tensors are given by a ˆ det…a ab † and g ˆ det…g ij †. In the laminated structures, the sti€ness properties are function of the normal coordinate. In Fig. 2, a crosssection of laminated shell composed of K layers is presented. The thickness of the kth layer is h k , the inner coordinate of the ®rst layer is x …0† 3 , the coordinate at the interface of the ®rst and the second layer is denoted by x …1† 3 , etc. A linear elastic properties of the anisotropic layers are characterized by the tensor of elasticity A. This tensor is function of the coordinate x 3 , i.e., A ˆ A…x 3 †. Fig. 2. Cross-section of laminated shell composed of K layers. In many applications, it can be assumed that calculations of shell sti€ness properties can be performed neglecting the di€erences in spatial and shell midsurface metrics, i.e., a ab g ab . In this case, the Hooke's law for each layer can be written by r ij ˆ A ijkl e kl : …13† Here A ijkl is the component of the elasticity tensor in the midsurface metrics. Substituting Eq. (13) into (11) and taking into account the relations (12) the strain energy U can be expressed by Z Z h=2 U ˆ A ijkl e ij e kl dx 3 dA: …14† A h=2 After integration, throughout the thickness, the strain energy can be obtained in terms of shell quantities: stress resultants and couples and laminated shell sti€ness characteristics …Q abcd ; B abcd ; D abcd †ˆ Z h=2 h=2 A abcd …1; x 3 ; x 2 3 † dx 3; Z h=2 Q a3b3 ˆ c …a3† A a3b3 dx 3 : h=2 …15† Here c …a3† are shear correction coecients. These shear correction coecients must be used for laminated shells for more correct representation of the transverse shear sti€ness properties. Calculation of the shear correction coecients can be performed by using various methods. Some approaches have been discussed in [21±23]. Further in numerical examples the matrix notations for the shear correction coecients are used. k 2 4 ˆ c …23†; k 2 5 ˆ c …13†: Since the elastic properties are to be constant throughout the layer thickness the expressions (15) can be easily integrated
Page 1: Composite Structures 51 (2001) 361
Page 5 and 6: R. Rikards et al. / Composite Struc

Analysis for buckling and vibrations of composite ... - ResearchGate

Create successful ePaper yourself

Delete template?

Save as template?