Analysis for buckling and vibrations of composite ... - ResearchGate

366 R. Rikards et al. / Composite Structures 51 (2001) 361±370
Fig. 7. Geometry of the single ribbed plate.
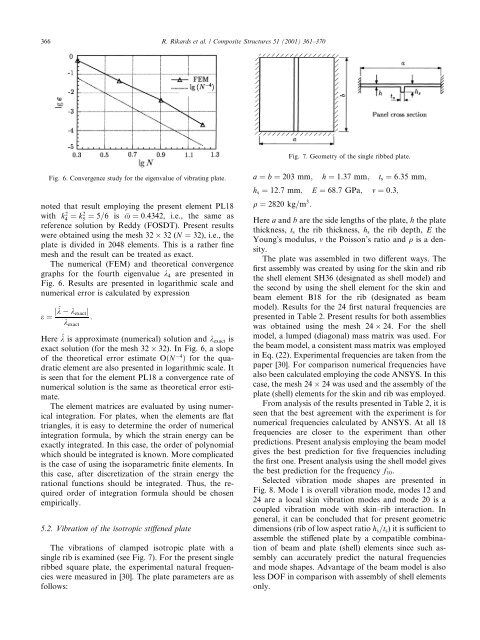
Fig. 6. Convergence study for the eigenvalue of vibrating plate.
noted that result employing the present element PL18
with k4 2 ˆ k2 5 ˆ 5=6 is x ˆ 0:4342, i.e., the same as
reference solution by Reddy (FOSDT). Present results
were obtained using the mesh 32 32 (N ˆ 32), i.e., the
plate is divided in 2048 elements. This is a rather ®ne
mesh and the result can be treated as exact.
The numerical (FEM) and theoretical convergence
graphs for the fourth eigenvalue k 4 are presented in
Fig. 6. Results are presented in logarithmic scale and
numerical error is calculated by expression
e ˆ j^k k exact j
k exact
:
Here ^k is approximate (numerical) solution and k exact is
exact solution (for the mesh 32 32). In Fig. 6, a slope
of the theoretical error estimate O…N 4 † for the quadratic
element are also presented in logarithmic scale. It
is seen that for the element PL18 a convergence rate of
numerical solution is the same as theoretical error estimate.
The element matrices are evaluated by using numerical
integration. For plates, when the elements are ¯at
triangles, it is easy to determine the order of numerical
integration formula, by which the strain energy can be
exactly integrated. In this case, the order of polynomial
which should be integrated is known. More complicated
is the case of using the isoparametric ®nite elements. In
this case, after discretization of the strain energy the
rational functions should be integrated. Thus, the required
order of integration formula should be chosen
empirically.
5.2. Vibration of the isotropic sti€ened plate
The vibrations of clamped isotropic plate with a
single rib is examined (see Fig. 7). For the present single
ribbed square plate, the experimental natural frequencies
were measured in [30]. The plate parameters are as
follows:
a ˆ b ˆ 203 mm; h ˆ 1:37 mm; t s ˆ 6:35 mm;
h s ˆ 12:7 mm; E ˆ 68:7 GPa; m ˆ 0:3;
q ˆ 2820 kg=m 3 :
Here a and b are the side lengths of the plate, h the plate
thickness, t s the rib thickness, h s the rib depth, E the
Young's modulus, m the Poisson's ratio and q is a density.
The plate was assembled in two di€erent ways. The
®rst assembly was created by using for the skin and rib
the shell element SH36 (designated as shell model) and
the second by using the shell element for the skin and
beam element B18 for the rib (designated as beam
model). Results for the 24 ®rst natural frequencies are
presented in Table 2. Present results for both assemblies
was obtained using the mesh 24 24. For the shell
model, a lumped (diagonal) mass matrix was used. For
the beam model, a consistent mass matrix was employed
in Eq. (22). Experimental frequencies are taken from the
paper [30]. For comparison numerical frequencies have
also been calculated employing the code ANSYS. In this
case, the mesh 24 24 was used and the assembly of the
plate (shell) elements for the skin and rib was employed.
From analysis of the results presented in Table 2, it is
seen that the best agreement with the experiment is for
numerical frequencies calculated by ANSYS. At all 18
frequencies are closer to the experiment than other
predictions. Present analysis employing the beam model
gives the best prediction for ®ve frequencies including
the ®rst one. Present analysis using the shell model gives
the best prediction for the frequency f 10 .
Selected vibration mode shapes are presented in
Fig. 8. Mode 1 is overall vibration mode, modes 12 and
24 are a local skin vibration modes and mode 20 is a
coupled vibration mode with skin±rib interaction. In
general, it can be concluded that for present geometric
dimensions (rib of low aspect ratio h s =t s ) it is sucient to
assemble the sti€ened plate by a compatible combination
of beam and plate (shell) elements since such assembly
can accurately predict the natural frequencies
and mode shapes. Advantage of the beam model is also
less DOF in comparison with assembly of shell elements
only.