Analysis for buckling and vibrations of composite ... - ResearchGate

More documents

Recommendations

Info

364 R. Rikards et al. / Composite Structures 51 (2001) 361±370 Q abcd ˆ XK B abcd ˆ 1 2 kˆ1 X K kˆ1 A abcd …k† ‰x 3…k† x 3…k1† Š; A abcd …k† ‰x 2 3…k† x2 3…k1† Š; D abcd ˆ 1 X K A abcd …k† ‰x 3 3…k† 3 x3 3…k1† Š; kˆ1 Q a3b3 ˆ c …a3† X K Here A ijkl …k† kth layer. kˆ1 4. Finite elements A a3b3 …k† ‰x 3…k† x 3…k1† Š: …16† are components of the elasticity tensor of the In [17] for the shell analysis, it was proposed to use two ®nite elements based on FOSDT-5 (see Fig. 3). The ®rst element with six nodes called SH30 is based on the second-order approximation of displacements. The second element with 10 nodes called SH50 is based on the third-order approximation of displacements. Since the element SH50 has internal node and is too complicated, further only the quadratic element was developed [18]. However, the cubic element has higher convergence rate [17]. For sti€ened shells, two di€erent shell surfaces should be assembled. In this case, in order to obtain a continuous displacement and rotation ®elds the FOSDT-6 should be used. The displacements (10) are approximated by the second-order polynomial shape functions (see [1]) w T ˆ‰N 1 I; N 2 I; ...; N 6 IŠv e ˆ Nv e : …17† Here I is a 6 6 unity matrix for the FOSDT-6, a 5 5 unity matrix for the FOSDT-5 and a 3 3 unity matrix for the FOSDT-3. For the FOSDT-6, the element displacement vector v e is given by v T e ˆ‰ve 1 ; ve 2 ; ...; ve 6 Š; v eT i ˆ‰u i ; v i ; w i ; c i …1† ; ci …2† ; c iŠ: …18† Here v e i …i ˆ 1; 2; ...; 6† are displacements for the ith node. For the FOSDT-5, the element displacement vector in Eq. (17) is given by v T e ˆ ve 1 ; ve 2 ; ...; h i ve 6 ; v eT i ˆ u i ; v i ; w i ; c i …1† ; ci …2† : …19† For the FOSDT-3, the element displacement vector in Eq. (17) is given by v T e ˆ ve 1 ; ve 2 ; ...; h i ve 6 ; v eT i ˆ w i ; c i …1† ; ci …2† : …20† Therefore, at all three triangular ®nite elements for analysis of shells and plates are considered. 1. The ®nite element SH36 based on second-order approximation of displacements according the FOS- DT-6. 2. The ®nite element SH30 based on second-order approximation of displacements according the FOS- DT-5. 3. The ®nite element PL18 based on second-order approximation of displacements according the FOS- DT-3. Here, the plate element PL18 is considered only for the convergence studies. For discretization of the sti€ened structures, several idealizations can be used in which the assemblies can be treated as collections of the shell, plate and beam elements. The sti€eners depending on dimensions can be idealized as a shell or beam elements. For light sti€eners, the overall (global) buckling or vibration modes occur and in this case the beam elements can be used. If the sti€ener is ¯exurally and rotationally sti€ and skin of the shell is thin, then the local mode of the skin is dominant. In this case for sti€eners also the beam elements can be used. In the case of torsionally weak sti€ener, a local buckling occurs in the sti€ener. In this case, the sti€ener should be idealized by using the shell (plate) elements. Therefore, the idealization depends upon the geometric characteristics of the attached sti€ener. In order to reduce the degrees of freedom of assembled structure for some geometric dimensions of sti€ener a spatial beam elements can be used. Let us consider the spatial beam element compatible with the shell ®nite element SH36. So, for the beam a second-order approximation of displacements and rotations should be used. A beam element with three nodes is presented in Fig. 4. For the present element a Timoshenko's beam theory is used to derive the element matrices. In addition a torsional deformation of the beam is taken into Fig. 3. Isoparametric triangular ®nite elements with six (a) and 10 (b) nodes. Fig. 4. Spatial Timoshenko's beam ®nite element B18.
R. Rikards et al. / Composite Structures 51 (2001) 361±370 365 account. So, the beam displacement vector is the same as for shell Eq. (10) and the element displacement vector is given by v T e ˆ‰ve 1 ; ve 2 ; ve 3 Š; h veT i ˆ u i ; v i ; w i ; c i …1† ; ci …2† ; c i i : …21† At all for the present beam element there are 18 DOF and the element is designated as B18. Similar Timoshenko's beam ®nite element employing the third-order approximations was developed in [24]. The ®nite elements SH36 and B18 can be used to assemble the sti€ened shells of an arbitrary shape since for both isoparametric elements the mapping technique is applied. The locking phenomenon for these elements can be avoided by using a selective integration technique [25]. 5. Numerical examples Further the vibration and buckling problems of di€erent structures are analyzed. In order to evaluate the natural frequencies a linear eigenvalue problem is solved Ku kMu ˆ 0: …22† Here K and M is a global sti€ness and mass matrices, u is global displacement vector and k ˆ x 2 , where x ˆ 2pf is a circular frequency and f is a frequency. The subspace iteration method [26], which is widely used for large scale ®nite element calculations, is employed to solve Eq. (22). Di€erent methods are employed to estimate buckling loads. 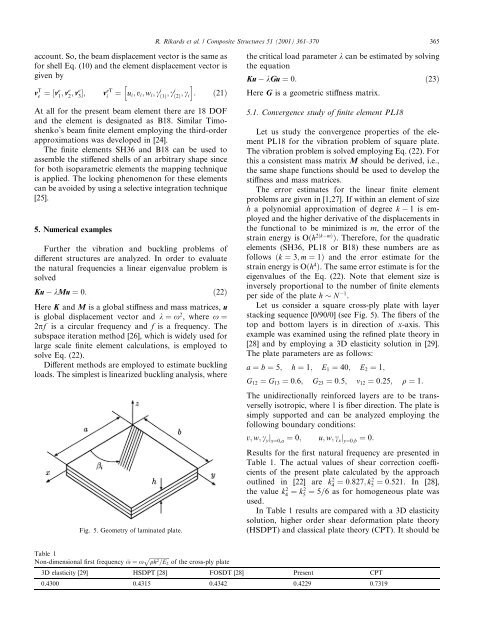The simplest is linearized buckling analysis, where Fig. 5. Geometry of laminated plate. the critical load parameter k can be estimated by solving the equation Ku kGu ˆ 0: …23† Here G is a geometric sti€ness matrix. 5.1. Convergence study of ®nite element PL18 Let us study the convergence properties of the element PL18 for the vibration problem of square plate. The vibration problem is solved employing Eq. (22). For this a consistent mass matrix M should be derived, i.e., the same shape functions should be used to develop the sti€ness and mass matrices. The error estimates for the linear ®nite element problems are given in [1,27]. If within an element of size h a polynomial approximation of degree k 1 is employed and the higher derivative of the displacements in the functional to be minimized is m, the error of the strain energy is O…h 2…km† †. Therefore, for the quadratic elements (SH36, PL18 or B18) these numbers are as follows …k ˆ 3; m ˆ 1† and the error estimate for the strain energy is O…h 4 †. The same error estimate is for the eigenvalues of the Eq. (22). Note that element size is inversely proportional to the number of ®nite elements per side of the plate h N 1 . Let us consider a square cross-ply plate with layer stacking sequence [0/90/0] (see Fig. 5). The ®bers of the top and bottom layers is in direction of x-axis. This example was examined using the re®ned plate theory in [28] and by employing a 3D elasticity solution in [29]. The plate parameters are as follows: a ˆ b ˆ 5; h ˆ 1; E 1 ˆ 40; E 2 ˆ 1; G 12 ˆ G 13 ˆ 0:6; G 23 ˆ 0:5; m 12 ˆ 0:25; q ˆ 1: The unidirectionally reinforced layers are to be transverselly isotropic, where 1 is ®ber direction. The plate is simply supported and can be analyzed employing the following boundary conditions: v; w; c y j xˆ0;a ˆ 0; u; w; c x j yˆ0;b ˆ 0: Results for the ®rst natural frequency are presented in Table 1. The actual values of shear correction coecients of the present plate calculated by the approach outlined in [22] are k4 2 ˆ 0:827; k2 5 ˆ 0:521. In [28], the value k4 2 ˆ k2 5 ˆ 5=6 as for homogeneous plate was used. In Table 1 results are compared with a 3D elasticity solution, higher order shear deformation plate theory (HSDPT) and classical plate theory (CPT). It should be Table 1 p Non-dimensional ®rst frequency x ˆ x qh 2 =E 2 of the cross-ply plate 3D elasticity [29] HSDPT [28] FOSDT [28] Present CPT 0.4300 0.4315 0.4342 0.4229 0.7319
Page 1 and 2: Composite Structures 51 (2001) 361
Page 3: in di€erent curvilinear coordinat
Page 7 and 8: R. Rikards et al. / Composite Struc
Page 9 and 10: R. Rikards et al. / Composite Struc

Analysis for buckling and vibrations of composite ... - ResearchGate

Create successful ePaper yourself

Delete template?

Save as template?