EQUATIONS OF ELASTIC HYPERSURFACES

More documents

Recommendations

Info

88 SHELLS where I denotes the identity. The incisive step in the direction of identifying all such operators is the observation that any other operator of the type is a linear combination of these two. Namely, we have the following. Proposition 3.25 Consider a linear operator T, as in (3.86), such that the isotropy condition (3.97) holds. Then T satisfies the first equality in (3.91) if and only if it satisfies the second equality in (3.91). Furthermore, any linear operator T which satisfies (3.97) along with either the first or the second equality in (3.91) has the form for some constants λ, µ ∈ R. TA = λ (Tr A)I + µ (A + A ⊤ ), A ∈ M n,n (R), (3.99) Proof: Let us first show that any linear operator (3.86) satisfying (3.91), (3.97) is represented in the form (3.99). By the previous discussion (cf. (3.99)), it suffices to prove that the space of linear operators (3.86) satisfying (3.91), (3.97) has dimension two. 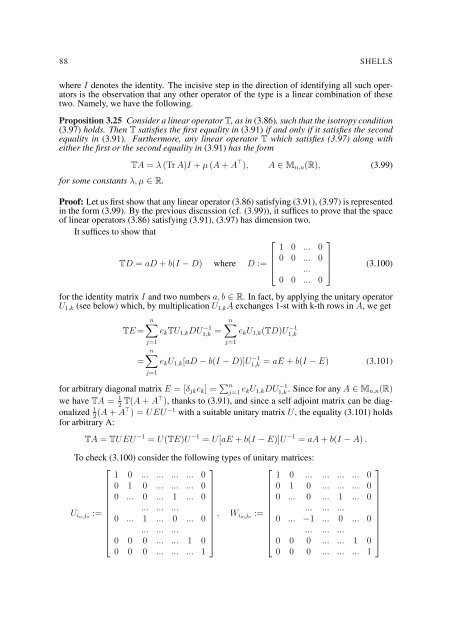It suffices to show that TD = aD + b(I − D) where D := ⎡ ⎢ ⎣ 1 0 ... 0 0 0 ... 0 ... 0 0 ... 0 ⎤ ⎥ ⎦ (3.100) for the identity matrix I and two numbers a, b ∈ R. In fact, by applying the unitary operator U 1,k (see below) which, by multiplication U 1,k A exchanges 1-st with k-th rows in A, we get TE = = n∑ n∑ e k TU 1,k DU −1 1,k = j=1 n∑ j=1 j=1 e k U 1,k [aD − b(I − D)]U −1 1,k e k U 1,k (TD)U −1 1,k = aE + b(I − E) (3.101) for arbitrary diagonal matrix E = [δ jk e k ] = ∑ n j=1 e kU 1,k DU −1 1,k . Since for any A ∈ M n,n(R) we have TA = 1 2 T(A + A⊤ ), thanks to (3.91), and since a self adjoint matrix can be diagonalized 1 2 (A + A⊤ ) = UEU −1 with a suitable unitary matrix U, the equality (3.101) holds for arbitrary A: TA = TUEU −1 = U(TE)U −1 = U[aE + b(I − E)]U −1 = aA + b(I − A) . To check (3.100) consider the following types of unitary matrices: ⎡ ⎤ ⎡ ⎤ 1 0 ... ... ... ... 0 1 0 ... ... ... ... 0 0 1 0 ... ... ... 0 0 1 0 ... ... ... 0 0 ... 0 ... 1 ... 0 0 ... 0 ... 1 ... 0 U io ,j o := ... ... ... 0 ... 1 ... 0 ... 0 , W io ,j o := ... ... ... 0 ... −1 ... 0 ... 0 ⎢ ... ... ... ⎥ ⎢ ... ... ... ⎥ ⎣ 0 0 0 ... ... 1 0 ⎦ ⎣ 0 0 0 ... ... 1 0 ⎦ 0 0 0 ... ... ... 1 0 0 0 ... ... ... 1
3. CALCULUS <strong>OF</strong> DIFFERENTIAL OPERATORS ON <strong>HYPERSURFACES</strong> 89 where the only non-zero, off the diagonal entries are at (i o , j o ) and (j o , i o ). Next, set A := TD, A = [ a ij ]1≤i,j≤n and observe that D is invariant under conjugation by W io ,j o , i.e. W io ,j o DW ⊤ i o ,j o = D, as long as i o ≠ 1 and j o ≠ 1. Thus, by (3.97), the same is true for A = TD which, in turn, eventually implies that a io i o = a jo j o , ∀ i o , j o ≠ 1. (3.102) The next observation is that D is invariant under conjugation by the product U io j o W io ,j o , i.e. U io j o W io ,j o DWi ⊤ o ,j o Ui ⊤ o j o = D, this time for every 1 ≤ i o ≠ j o ≤ n. Hence, by (3.97), the same holds for A = TD, which ultimately implies that a io j o = −a jo i o for every pair of indices 1 ≤ i o ≠ j o ≤ n. Consequently, a io j o = 0, for every 1 ≤ i o ≠ j o ≤ n. (3.103) Under the current assumptions, i.e. (3.97), the first condition in (3.91), the desired conclusion, i.e. that TD has the two-parameter diagonal form indicated above, now follows readily from (3.102) and (3.103). There remains to analyze the case when the linear operator T satisfies (3.97) along with the second condition in (3.91). In this situation, it can be readily checked that T ∗ , the adjoint of T with respect to the inner product (3.87), satisfies (3.97) and the first condition in (3.91), so the previous reasoning applies. Consequently, T ∗ can be represented in the form (3.99), which is invariant under adjunction. Hence T can be written in the form (3.99) also. In particular, (3.99) holds in this case as well, and this finishes the proof of the proposition. Remark 3.26 A posteriori, the conditions (3.91) and (3.97) imply that the linear operator (3.86) has the form (3.99) and, in particular, is self adjoint, i.e., imply the condition (3.89). Remark 3.27 The above proof can be modified to hold in the case when (3.97) is (seemingly) weakened to allow only orientation preserving unitary matrices U. All one has to do in this later case is to employ the invariance of D under conjugation by U ko l o U io j o W io ,j o (with k o , l o ≠ 1), in place of conjugation by (the inversion) U io j o W io ,j o as in the original proof. We are now ready to derive the Lamé equations of elasticity on a hypersurface. Theorem 3.28 On a smooth hypersurface S in R n , modeling a homogeneous, linear, isotropic, elastic medium, the Lamé operator L S is given by L S = −λ ∇ S Div S + 2µ Def ∗ S Def S = λ Div ∗ S Div S + 2µ Def ∗ S Def S . (3.104) In particular, L S is a formally self-adjoint differential operator of second order. Proof: According to the discussion in the first part of this section, the elasticity tensor in the case of linear, isotropic, elastic medium is given by (3.99), where λ, µ are the Lamé moduli. Applying the following properties of the trace Tr(A + B) = Tr(A) + Tr(B) , 〈A + A ⊤ , A〉 = T r [ (A + A ⊤ )A ⊤] = 1 2 T r(A + A⊤ ) 2 , (3.105)
Page 1 and 2:
Grant GNSF/ST06/3-001 of the Georgi
Page 3 and 4:
1. INTRODUCTION 3 1 INTRODUCTION Pa
Page 5 and 6:
1. INTRODUCTION 5 defines the canon
Page 7 and 8:
2. OUTLINE OF DIFFERENTIAL GEOMETRY
Page 9 and 10:
Page 11 and 12:
Page 13 and 14:
Page 15 and 16:
Page 17 and 18:
Page 19 and 20:
Page 21 and 22:
Page 23 and 24:
Page 25 and 26:
Page 27 and 28:
Page 29 and 30:
Page 31 and 32:
Page 33 and 34:
Page 35 and 36:
Page 37 and 38: 2. OUTLINE OF DIFFERENTIAL GEOMETRY
Page 65 and 66: 3. CALCULUS OF DIFFERENTIAL OPERATO
Page 87: 3. CALCULUS OF DIFFERENTIAL OPERATO
Page 101 and 102: 4. BOUNDARY VALUE PROBLEMS: GENERAL
Page 139 and 140:
4. BOUNDARY VALUE PROBLEMS: GENERAL
Page 141 and 142:
Page 143 and 144:
Page 145 and 146:
Page 147 and 148:
5. BOUNDARY VALUE PROBLEMS: EXAMPLE
Page 149 and 150:
Page 151 and 152:
Page 153 and 154:
Page 155 and 156:
Page 157 and 158:
Page 159 and 160:
Page 161 and 162:
Page 163 and 164:
Page 165 and 166:
Page 167 and 168:
Page 169 and 170:
Page 171 and 172:
Page 173 and 174:
Page 175 and 176:
Page 177 and 178:
Page 179 and 180:
Page 181 and 182:
BIBLIOGRAPHY 181 [CD1] O.Chkadua, R
Page 183 and 184:
BIBLIOGRAPHY 183 [Hr1] L. Hörmande
Page 185:
BIBLIOGRAPHY 185 [Si1] I. Simonenko
show all

EQUATIONS OF ELASTIC HYPERSURFACES

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?