Liquid interfaces in viscous straining flows ... - Itai Cohen Group

More documents

Recommendations

Info

Liquid interfaces in viscous straining flows 179Therefore, u 2 , the disturbance velocity field in the lower layer, satisfies∇·σ 2 = −∇p 2 + μ∇ 2 u 2 = 0, ∇·u 2 = 0, (3.4)where p 2 is the pressure field in the lower layer and σ 2 thefluidstressfieldinthelower layer.Since the flow dynamics in both layers are described by the linear Stokes equations,we can represent the velocity field as an integral over a suitably defined closedsurface, as discussed in earlier works and in textbooks on viscous flow (Lorentz1907; Ladyzhenskaya 1963; Pozrikidis 1992). The key result of the boundary-integralformulation is that a Stokes velocity field u at a point x is given by an integral overany closed surface S enclosing x. The surface integral has the form∫∫u(x) = J(r) · [n · σ ( y)] dS y + n · K(r) · u( y) dS y , (3.5)Swhere y is the point on the surface that is being integrated over and n is an outwardpointingsurface normal. The tensors J and K are defined as:J(r) = 1 ( 18πμ r + rr ), K(r) =− 3 rrr(r = x − y).r 3 4π r 5Physically, (3.5) indicates that the velocity at the interior point x can be expressed asa sum over two different kinds of contributions over the closed surface S. The firstterm on the right-hand side of (3.5) corresponds to the fluid stress exerted by flowspast the enclosing surface. The second term describes contributions corresponding toflows into and along the closed surface S. If the point x lies outside the enclosedvolume, the contributions over the closed surface cancel, so that the right-hand sideof (3.5) sums to 0. A point on the closed surface S is a special case. When the closedsurface S is continuous and smoothly varying, the velocity at x is simply an averageof the contribution from S to x if it is in the exterior and the contribution from S tox if it is an interior point. As a result, the velocity for a point x on the surface S canbe written as∫1u(x) = 2SS∫J(r) · [n · σ ( y)] dS y + n · K(r) · u( y) dS y . (3.6)SThese results allow us to derive an integral equation for the time-evolution of theinterface in our idealized withdrawal problem. We define the surface S ∞ , which hasthe shape of a hemispherical shell with radius R ∞ such that R ∞ goes to infinity.We then divide the liquid interface into two portions, an inner portion, denoted byS I , corresponding to the interface within a radial distance a of the origin, and anouter portion, denoted by S O , corresponding to the rest of the interface. We thenassume that the sink height is always much smaller than a so that contributionsfrom the integral over S O are small compared with contribution from S I , and entirelydiscard the contribution from S O . Our numerical results show that this is a reasonableprocedure as long as the sink height ˜S is smaller than a (§ 4.2). In that regime, thehump height and radius of curvature are little influenced by the errors incurredin neglecting S O . Starting from (3.5) and neglecting the contribution from S O ,thedisturbance velocity u 1 in the upper layer can be written as a sum of integrals over S Iand S ∞ .Sinceu 1 decays rapidly to 0 at infinity, the contributions from the integralsover the shell S ∞ approach 0 as R ∞ is taken to infinity, so that only the contribution
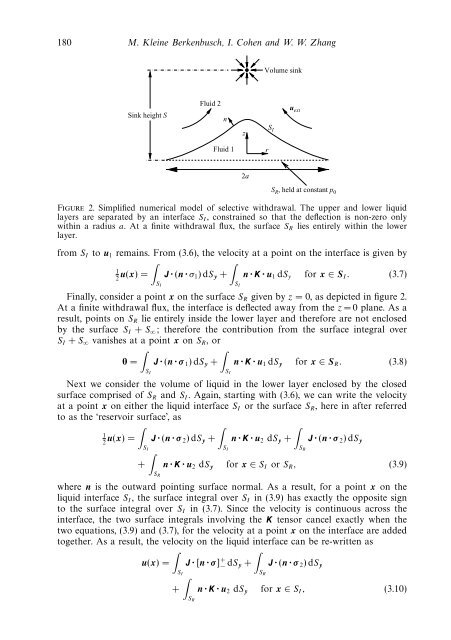
180 M. Kleine Berkenbusch, I. Cohen and W. W. ZhangVolume sinkSink height SFluid 2nFluid 1zrS Iu ext2aS R , held at constant p 0Figure 2. Simplified numerical model of selective withdrawal. The upper and lower liquidlayers are separated by an interface S I , constrained so that the deflection is non-zero onlywithin a radius a. At a finite withdrawal flux, the surface S R lies entirely within the lowerlayer.from S I to u 1 remains. From (3.6), the velocity at a point on the interface is given by∫1u(x) = J · (n · σ2 1 )dS y + n · K · u 1 dS y for x ∈ S I . (3.7)∫S I S IFinally, consider a point x on the surface S R given by z = 0, as depicted in figure 2.At a finite withdrawal flux, the interface is deflected away from the z = 0 plane. As aresult, points on S R lie entirely inside the lower layer and therefore are not enclosedby the surface S I + S ∞ ; therefore the contribution from the surface integral overS I + S ∞ vanishes at a point x on S R ,or∫∫0 = J · (n · σ 1 )dS y + n · K · u 1 dS y for x ∈ S R . (3.8)S I S INext we consider the volume of liquid in the lower layer enclosed by the closedsurface comprised of S R and S I . Again, starting with (3.6), we can write the velocityat a point x on either the liquid interface S I or the surface S R , here in after referredto as the ‘reservoir surface’, as∫∫1u(x) = J · (n · σ2 2 )dS y + n · K · u 2 dS y + J · (n · σ 2 )dS y∫S I S I S∫R+ n · K · u 2 dS y for x ∈ S I or S R , (3.9)S Rwhere n is the outward pointing surface normal. As a result, for a point x on theliquid interface S I , the surface integral over S I in (3.9) has exactly the opposite signto the surface integral over S I in (3.7). Since the velocity is continuous across theinterface, the two surface integrals involving the K tensor cancel exactly when thetwo equations, (3.9) and (3.7), for the velocity at a point x on the interface are addedtogether. As a result, the velocity on the liquid interface can be re-written as∫∫u(x) = J · [n · σ ] + − dS y + J · (n · σ 2 )dS yS I S∫R+ n · K · u 2 dS y for x ∈ S I , (3.10)S R
Page 6 and 7: Liquid interfaces in viscous strain
Page 26 and 27: 10 0Liquid interfaces in viscous st
Page 34: Liquid interfaces in viscous strain

Liquid interfaces in viscous straining flows ... - Itai Cohen Group

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?