Continuidad de funciones de varias variables.

Solución
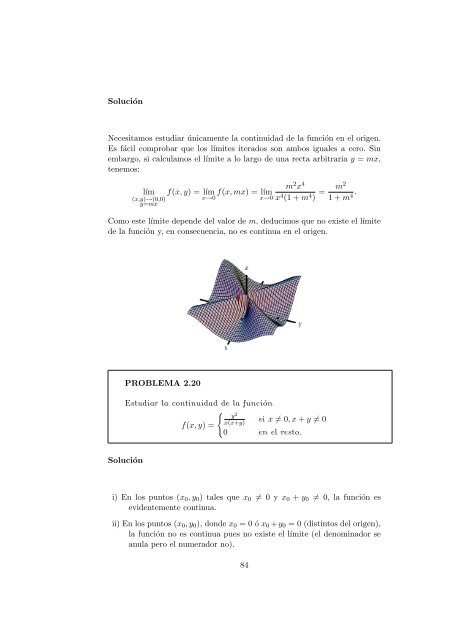
Necesitamos estudiar únicamente la continuidad de la función en el origen.
Es fácil comprobar que los límites iterados son ambos iguales a cero. Sin
embargo, si calculamos el límite a lo largo de una recta arbitraria y = mx,
tenemos:
lím
(x,y)→(0,0)
y=mx
f(x, y) = lím
x→0 f(x, mx) = lím
x→0
m2x4 x4 (1 + m4 m2
= .
) 1 + m4 Como este límite depende del valor de m, deducimos que no existe el límite
de la función y, en consecuencia, no es continua en el origen.
PROBLEMA 2.20
Estudiar la continuidad de la función
y2 x(x+y) si x = 0, x + y = 0
f(x, y) =
0 en el resto.
Solución
i) En los puntos (x0, y0) tales que x0 = 0 y x0 + y0 = 0, la función es
evidentemente continua.
ii) En los puntos (x0, y0), donde x0 = 0 ó x0 + y0 = 0 (distintos del origen),
la función no es continua pues no existe el límite (el denominador se
anula pero el numerador no).
84