LR Rabiner and RW Schafer, June 3

DRAFT: L. R. Rabiner and R. W. Schafer, June 3, 2009
8.5. HOMOMORPHIC FILTERING OF NATURAL SPEECH 467
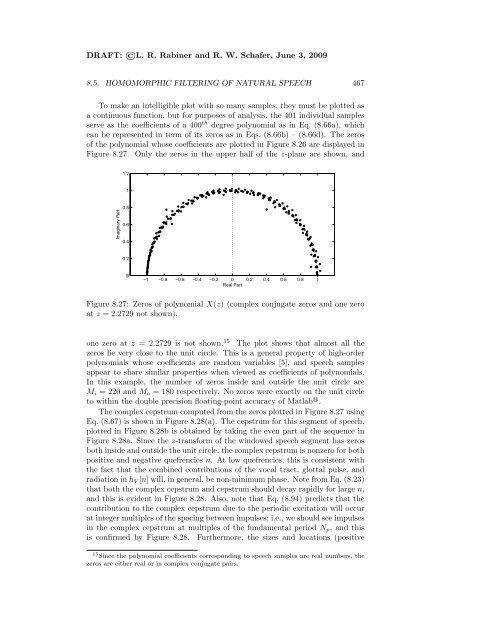
To make an intelligible plot with so many samples, they must be plotted as
a continuous function, but for purposes of analysis, the 401 individual samples
serve as the coefficients of a 400 th degree polynomial as in Eq. (8.66a), which
can be represented in term of its zeros as in Eqs. (8.66b) – (8.66d). The zeros
of the polynomial whose coefficients are plotted in Figure 8.26 are displayed in
Figure 8.27. Only the zeros in the upper half of the z-plane are shown, and
Imaginary Part
1.2
1
0.8
0.6
0.4
0.2
0
−1 −0.8 −0.6 −0.4 −0.2 0
Real Part
0.2 0.4 0.6 0.8 1
Figure 8.27: Zeros of polynomial X(z) (complex conjugate zeros and one zero
at z = 2.2729 not shown).
one zero at z = 2.2729 is not shown. 15 The plot shows that almost all the
zeros lie very close to the unit circle. This is a general property of high-order
polynomials whose coefficients are random variables [5], and speech samples
appear to share similar properties when viewed as coefficients of polynomials.
In this example, the number of zeros inside and outside the unit circle are
Mi = 220 and Mo = 180 respectively. No zeros were exactly on the unit circle
to within the double precision floating-point accuracy of Matlab .
The complex cepstrum computed from the zeros plotted in Figure 8.27 using
Eq. (8.67) is shown in Figure 8.28(a). The cepstrum for this segment of speech,
plotted in Figure 8.28b is obtained by taking the even part of the sequence in
Figure 8.28a. Since the z-transform of the windowed speech segment has zeros
both inside and outside the unit circle, the complex cepstrum is nonzero for both
positive and negative quefrencies n. At low quefrencies, this is consistent with
the fact that the combined contributions of the vocal tract, glottal pulse, and
radiation in hV [n] will, in general, be non-minimum phase. Note from Eq. (8.23)
that both the complex cepstrum and cepstrum should decay rapidly for large n,
and this is evident in Figure 8.28. Also, note that Eq. (8.94) predicts that the
contribution to the complex cepstrum due to the periodic excitation will occur
at integer multiples of the spacing between impulses; i.e., we should see impulses
in the complex cepstrum at multiples of the fundamental period Np, and this
is confirmed by Figure 8.28. Furthermore, the sizes and locations (positive
15 Since the polynomial coefficients corresponding to speech samples are real numbers, the
zeros are either real or in complex conjugate pairs.