LR Rabiner and RW Schafer, June 3

DRAFT: L. R. Rabiner and R. W. Schafer, June 3, 2009
8.2. HOMOMORPHIC SYSTEMS FOR CONVOLUTION 429
*
H { }
*
y[ n]
= H { x[
n]
}
x ∗ H { x n]
} ∗H
{ x [ n]
}
x[ n]
1[
n]
x2[
n]
1[
2
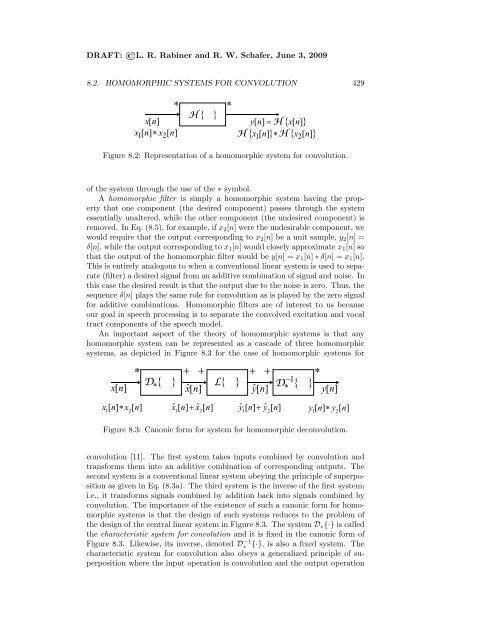
Figure 8.2: Representation of a homomorphic system for convolution.
of the system through the use of the ∗ symbol.
A homomorphic filter is simply a homomorphic system having the property
that one component (the desired component) passes through the system
essentially unaltered, while the other component (the undesired component) is
removed. In Eq. (8.5), for example, if x2[n] were the undesirable component, we
would require that the output corresponding to x2[n] be a unit sample, y2[n] =
δ[n], while the output corresponding to x1[n] would closely approximate x1[n] so
that the output of the homomorphic filter would be y[n] = x1[n] ∗ δ[n] = x1[n].
This is entirely analogous to when a conventional linear system is used to separate
(filter) a desired signal from an additive combination of signal and noise. In
this case the desired result is that the output due to the noise is zero. Thus, the
sequence δ[n] plays the same role for convolution as is played by the zero signal
for additive combinations. Homomorphic filters are of interest to us because
our goal in speech processing is to separate the convolved excitation and vocal
tract components of the speech model.
An important aspect of the theory of homomorphic systems is that any
homomorphic system can be represented as a cascade of three homomorphic
systems, as depicted in Figure 8.3 for the case of homomorphic systems for
x[ n]
x n x n
* + + + + *
D ∗{
} L{
}
−1
D { }
xn ˆ[ ]
yn ˆ[ ] ∗ yn [ ]
ˆ ˆ
ˆ ˆ
1[ ] ∗ 2[
] x1[ n] + x2[ n]
y1[ n] + y2[ n]
1 ∗ 2
y[ n] y [ n]
Figure 8.3: Canonic form for system for homomorphic deconvolution.
convolution [11]. The first system takes inputs combined by convolution and
transforms them into an additive combination of corresponding outputs. The
second system is a conventional linear system obeying the principle of superposition
as given in Eq. (8.3a). The third system is the inverse of the first system;
i.e., it transforms signals combined by addition back into signals combined by
convolution. The importance of the existence of such a canonic form for homomorphic
systems is that the design of such systems reduces to the problem of
the design of the central linear system in Figure 8.3. The system D∗{·} is called
the characteristic system for convolution and it is fixed in the canonic form of
Figure 8.3. Likewise, its inverse, denoted D −1
∗ {·}, is also a fixed system. The
characteristic system for convolution also obeys a generalized principle of superposition
where the input operation is convolution and the output operation