LR Rabiner and RW Schafer, June 3

DRAFT: L. R. Rabiner and R. W. Schafer, June 3, 2009
8.2. HOMOMORPHIC SYSTEMS FOR CONVOLUTION 431
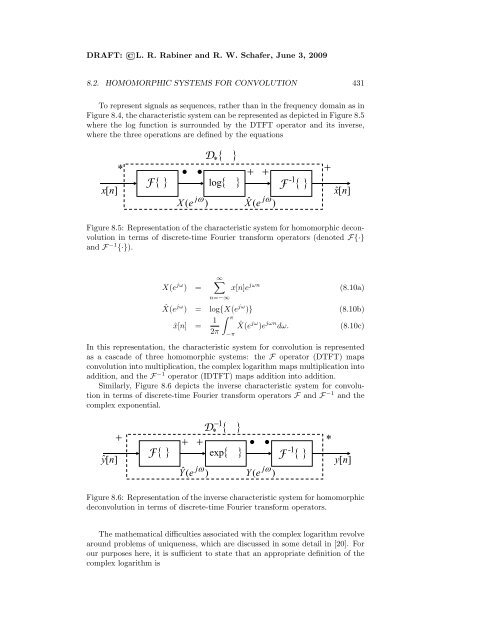
To represent signals as sequences, rather than in the frequency domain as in
Figure 8.4, the characteristic system can be represented as depicted in Figure 8.5
where the log function is surrounded by the DTFT operator and its inverse,
where the three operations are defined by the equations
D∗{
}
* • • + + +
F {} log{
}
-1
F { }
( )
ω j
x [n]
xˆ
[ n]
X e
ˆ jω
X ( e )
Figure 8.5: Representation of the characteristic system for homomorphic deconvolution
in terms of discrete-time Fourier transform operators (denoted F{·}
and F −1 {·}).
X(e jω ) =
∞
x[n]e jωn
(8.10a)
ˆX(e
n=−∞
jω ) = log{X(e jω )} (8.10b)
ˆx[n] = 1
2π
π
−π
ˆX(e jω )e jωn dω. (8.10c)
In this representation, the characteristic system for convolution is represented
as a cascade of three homomorphic systems: the F operator (DTFT) maps
convolution into multiplication, the complex logarithm maps multiplication into
addition, and the F −1 operator (IDTFT) maps addition into addition.
Similarly, Figure 8.6 depicts the inverse characteristic system for convolution
in terms of discrete-time Fourier transform operators F and F −1 and the
complex exponential.
+
yn ˆ[ ]
F {}
+
−1
D∗
{
+
exp{
}
}
• •
-1
F { }
*
yn [ ]
ˆ jω
Y ( e ) ( )
ω j
Y e
Figure 8.6: Representation of the inverse characteristic system for homomorphic
deconvolution in terms of discrete-time Fourier transform operators.
The mathematical difficulties associated with the complex logarithm revolve
around problems of uniqueness, which are discussed in some detail in [20]. For
our purposes here, it is sufficient to state that an appropriate definition of the
complex logarithm is