NEAR OPTIMAL BOUNDS IN FREIMAN'S THEOREM

More documents

Recommendations

Info

HOLOMORPHIC OPEN BOOK DECOMPOSITIONS 37 Definition 2.1 Let θ ∈ S 1 = R/2πZ denote polar coordinates on the unit disk D ⊂ R 2 by (r, φ), and let γ1,γ2 :[0, +∞) → R be smooth functions. A 1-form λ = γ1(r) dθ + γ2(r) dφ is called a local model near the binding if the following conditions are satisfied: (1) the functions γ1,γ2,andγ2(r)/r 2 are smooth if considered as functions on the disk D (in particular, γ ′ 1 (0) = γ ′ 2 (0) = γ2(0) = 0); (2) µ(r) := γ1(r)γ ′ 2 (r) − γ ′ 1 (r)γ2(r) > 0 if r>0; (3) γ1(0) > 0 and γ ′ 1 (r) < 0 if r>0; (4) limr→0(µ(r)/r) = γ1(0)γ ′′ 2 (0) > 0; (5) κ := (γ ′′ ′′ 1 (0)/γ 2 (0)) /∈ Z and κ ≤−1/2; (6) A(r) = (1/µ 2 (r))(γ ′′ 2 (r)γ ′ ′′ 1 (r) − γ 1 (r)γ ′ 2 We explain some of the conditions above. First, since (r)) is of order r for small r>0. λ ∧ dλ = µ(r)dθ ∧ dr ∧ dφ = µ(r) dθ ∧ dx ∧ dy, r the form λ is a contact form on S 1 × D. The Reeb vector field is given by X(θ,r,φ) = γ ′ 2 (r) µ(r) ∂ ∂θ − γ ′ 1 (r) µ(r) ∂ ∂φ The trajectories of X all lie on tori Tr = S 1 × ∂Dr: We compute and =: α(r) ∂ ∂θ + β(r) ∂ ∂φ . θ(t) = θ0 + α(r) t, φ(t) = φ0 + β(r) t. (2.1) γ lim α(r) = lim r→0 r→0 ′′ 2 (r) µ ′ (r) γ lim β(r) =−lim r→0 r→0 ′′ 1 (r) µ ′ (r) = γ ′′ 2 (0) Recalling that ∂ ∂ ∂ = x − y , we obtain for r = 0 ∂φ ∂y ∂x X = 1 ∂ γ1(0) ∂θ , γ1(0)γ ′′ 1 = (0) γ1(0) 2 =− γ ′′ 1 (0) γ1(0)γ ′′ 2 (0).
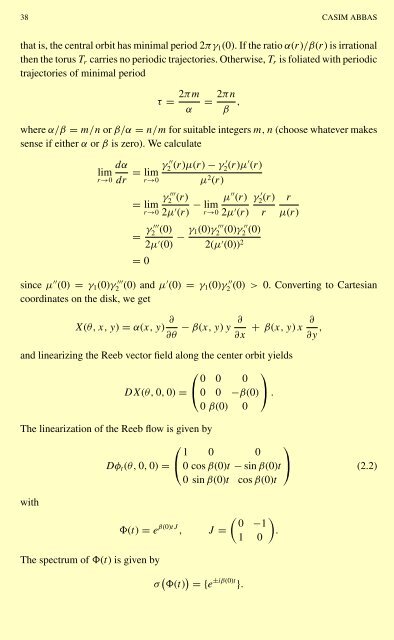
38 CASIM ABBAS that is, the central orbit has minimal period 2πγ1(0). If the ratio α(r)/β(r) is irrational then the torus Tr carries no periodic trajectories. Otherwise, Tr is foliated with periodic trajectories of minimal period τ = 2πm α = 2πn β , where α/β = m/n or β/α = n/m for suitable integers m, n (choose whatever makes sense if either α or β is zero). We calculate dα lim r→0 dr γ = lim r→0 ′′ 2 (r)µ(r) − γ ′ 2 (r)µ′ (r) µ 2 (r) γ = lim r→0 ′′′ 2 (r) 2µ ′ − lim (r) r→0 = γ ′′′ = 0 since µ ′′ (0) = γ1(0)γ ′′′ coordinates on the disk, we get 2 (0) 2µ ′ (0) µ ′′ (r) 2µ ′ γ (r) ′ 2 (r) r ′′′ ′′ γ1(0)γ 2 (0)γ 2 − (0) 2(µ ′ (0)) 2 2 (0) and µ′ (0) = γ1(0)γ ′′ 2 X(θ,x,y) = α(x,y) ∂ ∂θ − β(x,y) y ∂ ∂x r µ(r) (0) > 0. Converting to Cartesian + β(x,y) x ∂ ∂y , and linearizing the Reeb vector field along the center orbit yields ⎛ 0 0 0 ⎞ DX(θ,0, 0) = ⎝ 0 0 −β(0) ⎠ . 0 β(0) 0 The linearization of the Reeb flow is given by ⎛ 1 0 0 ⎞ Dφt(θ,0, 0) = ⎝ 0 cos β(0)t − sin β(0)t ⎠ (2.2) 0 sin β(0)t cos β(0)t with The spectrum of (t) is given by (t) = e β(0)tJ , J = σ (t) ={e ±iβ(0)t }. 0 −1 . 1 0
Page 1 and 2: NEAR OPTIMAL BOUNDS IN FREIMAN’S
Page 13 and 14: SUR LA NON-DENSITÉ DES POINTS ENTI
Page 29 and 30: 30 CASIM ABBAS Recall that the Reeb
Page 31 and 32: 32 CASIM ABBAS that Here the energy
Page 33 and 34: 34 CASIM ABBAS • uτ ( ˙S) ∩ u
Page 35: 36 CASIM ABBAS punctured surfaces w
Page 39 and 40: 40 CASIM ABBAS even have A(r) ≡ 0
Page 41 and 42: 42 CASIM ABBAS are contact forms on
Page 43 and 44: 44 CASIM ABBAS the standard complex
Page 45 and 46: 46 CASIM ABBAS and Jδε2 = xγ1(r)
Page 47 and 48: 48 CASIM ABBAS (λ0,J0) with vanish
Page 49 and 50: 50 CASIM ABBAS defined as H 1 j (S)
Page 51 and 52: 52 CASIM ABBAS and denoting the cor
Page 53 and 54: 54 CASIM ABBAS formula (2.2) for th
Page 55 and 56: 56 CASIM ABBAS Restricting any of t
Page 57 and 58: 58 CASIM ABBAS where fτ (∞) = li
Page 59 and 60: 60 CASIM ABBAS for τ
Page 61 and 62: 62 CASIM ABBAS Recalling our origin
Page 63 and 64: 64 CASIM ABBAS THEOREM 3.7 Let µ,
Page 65 and 66: 66 CASIM ABBAS Since w2 − w1C 1,
Page 67 and 68: 68 CASIM ABBAS be the conformal tra
Page 69 and 70: 70 CASIM ABBAS so that, for z ∈ B
Page 71 and 72: 72 CASIM ABBAS and, for every R>0,
Page 73 and 74: 74 CASIM ABBAS W 1,p loc (C) since
Page 75 and 76: 76 CASIM ABBAS (follows from 0 =
Page 77 and 78: 78 CASIM ABBAS is finite, the image
Page 79 and 80: 80 CASIM ABBAS Converting from coor
Page 81 and 82: 82 CASIM ABBAS [27] D. MCDUFF and D
Page 83 and 84: 84 GUILLARMOU and TZOU where ∂ν
Page 85 and 86: 86 GUILLARMOU and TZOU Carleman est
Page 87 and 88:
88 GUILLARMOU and TZOU where F ⊂
Page 89 and 90:
90 GUILLARMOU and TZOU LEMMA 2.2.4
Page 91 and 92:
92 GUILLARMOU and TZOU THEOREM 2.3.
Page 93 and 94:
94 GUILLARMOU and TZOU At a point (
Page 95 and 96:
96 GUILLARMOU and TZOU enough, we h
Page 97 and 98:
98 GUILLARMOU and TZOU Using the fa
Page 99 and 100:
100 GUILLARMOU and TZOU certain exp
Page 101 and 102:
102 GUILLARMOU and TZOU −1 where
Page 103 and 104:
104 GUILLARMOU and TZOU factor, or
Page 105 and 106:
106 GUILLARMOU and TZOU Proof of Pr
Page 107 and 108:
108 GUILLARMOU and TZOU where I1 =
Page 109 and 110:
110 GUILLARMOU and TZOU Proof of Le
Page 111 and 112:
112 GUILLARMOU and TZOU COROLLARY 6
Page 113 and 114:
114 GUILLARMOU and TZOU in x−k−
Page 115 and 116:
116 GUILLARMOU and TZOU Therefore,
Page 117 and 118:
118 GUILLARMOU and TZOU Proof of Pr
Page 119 and 120:
120 GUILLARMOU and TZOU [27] R. B.
Page 121 and 122:
122 MARGULIS and MOHAMMADI multiple
Page 123 and 124:
124 MARGULIS and MOHAMMADI As in [E
Page 125 and 126:
126 MARGULIS and MOHAMMADI planes
Page 127 and 128:
128 MARGULIS and MOHAMMADI α ∈ R
Page 129 and 130:
130 MARGULIS and MOHAMMADI We fix s
Page 131 and 132:
132 MARGULIS and MOHAMMADI Hence, i
Page 133 and 134:
134 MARGULIS and MOHAMMADI Hence th
Page 135 and 136:
136 MARGULIS and MOHAMMADI Indeed,
Page 137 and 138:
138 MARGULIS and MOHAMMADI For simp
Page 139 and 140:
140 MARGULIS and MOHAMMADI Note tha
Page 141 and 142:
142 MARGULIS and MOHAMMADI (ii) The
Page 143 and 144:
144 MARGULIS and MOHAMMADI needs to
Page 145 and 146:
146 MARGULIS and MOHAMMADI Proof Le
Page 147 and 148:
148 MARGULIS and MOHAMMADI all j>s,
Page 149 and 150:
150 MARGULIS and MOHAMMADI 0 c
Page 151 and 152:
152 MARGULIS and MOHAMMADI Some imp
Page 153 and 154:
154 MARGULIS and MOHAMMADI of G, an
Page 155 and 156:
156 MARGULIS and MOHAMMADI (ii) for
Page 157 and 158:
158 MARGULIS and MOHAMMADI PROPOSIT
Page 159:
160 MARGULIS and MOHAMMADI [EMM2]
show all

NEAR OPTIMAL BOUNDS IN FREIMAN'S THEOREM

Create successful ePaper yourself

Delete template?

Save as template?