1 GRADE 11 MATHEMATICS PAPER 3 GEOMETRY ... - AdMaths

GRADE 11 MATHEMATICS PAPER 3 GEOMETRY
LESSON 5
5.1 SIMILARITY
1 Polygons
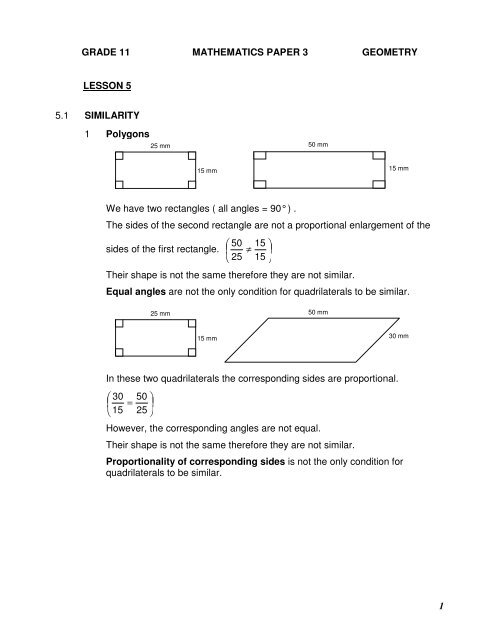
We have two rectangles ( all angles = 90° ) .
The sides of the second rectangle are not a proportional enlargement of the
⎛ 50 15 ⎞
sides of the first rectangle. ⎜ ≠ ⎟
⎝ 25 15 ⎠
Their shape is not the same therefore they are not similar.
Equal angles are not the only condition for quadrilaterals to be similar.
In these two quadrilaterals the corresponding sides are proportional.
⎛ 30 50 ⎞
⎜ = ⎟
⎝15
25 ⎠
25 mm
25 mm
15 mm
15 mm
50 mm
50 mm
However, the corresponding angles are not equal.
Their shape is not the same therefore they are not similar.
Proportionality of corresponding sides is not the only condition for
quadrilaterals to be similar.
15 mm
30 mm
1

25 mm
15 mm
40 mm
In the two rectangles above the corresponding angles are equal.
⎛ 40 24 8 ⎞
The corresponding sides are proportional. ⎜ = = ⎟
⎝ 25 15 5 ⎠
The shapes are similar. The second rectangle is an enlargement of the first.
These rectangles fulfil the conditions for two quadrilaterals to be similar.
The same conditions are necessary for the similarity of pentagons, etc.
Polygons are similar only if the are equiangular
and their corresponding sides are in proportion.
24 mm
The two conditions for polygons to be similar are dependent on each other.
2

GRADE 11 MATHEMATICS PAPER 3 GEOMETRY
2 Triangles
Our main interest is in the conditions under which two triangles are similar.
If two triangles are equiangular,
then their corresponding sides are in
proportion
and the two triangles are therefore similar.
If two triangles have proportional corresponding sides,
then the triangles are equiangular
and the triangles are therefore similar.
It is interesting that the conditions of equiangularity and proportionality of
corresponding sides in triangles are independent of each other.
Congruent triangles are always similar.
• The symbol for is similar to is
• Do not confuse with ≡ , which is the symbol for is congruent to.
• If ∆ ABC is similar to ∆ PQR , we write
∆ ABC
∆PQR
• In naming similar triangles it is advisable to follow the same convention
as that used for naming congruent triangle, i.e. the letters indicating
corresponding angles should be written in the same order for all
triangles.
3

If in ∆ ABC and ∆ PQR
Rˆ Â =
Pˆ Bˆ =
Qˆ Ĉ =
Then ∆ ABC ∆RPQ
It is now easy to write down the ratios which are equal.
AB
RP
B C
=
BC
PQ
=
AC
RQ
A
Notice that AB , BC and AC ( the numerators ) are the sides of one triangle
and RP , PQ and RQ ( the denominators ) are the sides of the other triangle.
P
Q R
4

GRADE 11 MATHEMATICS PAPER 3 GEOMETRY
5.2 The proofs of the following theorems must be known for exam purposes
Theorem 6 : When two triangles are equiangular, the corresponding sides
are proportional.
Given : ∆ ABC and ∆ PQR with Qˆ Bˆ P and ˆ Â = = .
Reqd. :
A
B C
Prove that
PQ
AB
=
QR
BC
=
RP
AC
Constr. : Mark off points S and T on PQ and PR respectively
such that PS = AB and PT = AC . Draw ST .
Proof : ∆PST ≡ ∆ABC ( S ∠ S )
Hence Qˆ Bˆ Sˆ 1 = = ( given )
So that ST QR ( corresponding angles equal )
Hence
And
PQ
=
PS
PR
PT
PQ PR
= ( PS = AB , PT = AC , construction )
AB AC
By marking points D and E on QP and QR respectively,
so that QD = BA and QE = BC , it can be shown that
PQ QR
= .
AB BC
hence
PQ
AB
=
QR
BC
=
RP
AC
P
1
S T
Q R
5

Conclusion
Equiangular triangles are similar.
A
B C
R . ˆ Q en Ĉ ˆ Bˆ P, ˆ Â = = =
Hence, ∆ ABC ∆PQR
Q R
Theorem 7 : If the corresponding sides of two triangles are
proportional, then the two triangles are equiangular.
( converse of theorem 6 )
B
Given :
A
∆ ABC and ∆ PQR with
AB
PQ
BC AC
= = .
QR PR
Reqd. : Prove that 1 R1 ˆ
Q en Ĉ ˆ Bˆ P, ˆ Â = = =
C
Constr. : Construct ∆ QSR on QR so that Bˆ Qˆ 2 = and Ĉ Rˆ 2 = ,
with S and P on opposite sides of QR .
Q
P
1
1
2 2
S
R
P
6

Proof :
∆ ABC and ∆ SQR are equiangular ( construction )
Hence
AB
=
SQ
BC
QR
Hence SQ = PQ .
AB
= ( given )
PQ
In the same way, SR = PR .
Hence ∆SQR ≡ ∆PQR ( S S S )
Hence Aˆ
= Sˆ
= Pˆ
Bˆ
= Qˆ
2 = Qˆ
1
and also Cˆ
= Rˆ
2 = Rˆ
1
Conclusion If the corresponding sides of two triangles are proportional,
then the triangles are similar.
AB
PQ
=
A
B C
BC
QR
=
AC
PR
Hence ∆ ABC ∆PQR
P
Q R
7

GRADE 11 MATHEMATICS PAPER 3 GEOMETRY
5.3 Useful hints for solving problems on similarity.
1 Always write the names of the triangles so that the equal angles occur in the
same order. Then it is not necessary to look at the figures to write down the
proportionality.
2 If two triangles, say ∆ AED and ∆ PRS , have been proved equiangular, the
ratios of the corresponding sides can be written down immediately.
∆ AED
AE
PR
=
ED
RS
∆PRS
3 It is advisable to write down all three ratios and then select the two ratios
which will give the required result.
If in point 2 above we were asked to prove that AE.PS = PR.AD , we could use
the first and third ratios and by cross-multiplication obtain the required result.
4 If two triangles are to be proved equiangular, it is only necessary to show that
two angles of the one triangle are equal to the two corresponding angles of the
other triangle. Often a common angle can be found.
5 Make separate sketches of the two triangles to be proved equiangular and
mark off the equal angles. ( This is especially useful when many facts are
given. )
Useful hints continues ….
=
AD
PS
8

GRADE 11 MATHEMATICS PAPER 3 GEOMETRY
6 If we have to prove the product of two line segments equal to the product of
two other line segments, the implication is that we have to prove two triangles
equiangular, and hence similar.
It is often difficult to recognise the two triangles.
We proceed as follows :
Write down the two equal products as a proportion.
We use an example to illustrate this.
B
Reqd. : Prove that BQ.RP = AP.RQ .
Analysing the problem
Two proportionals equivalent to BQ.RP = AP.RQ are
A
BQ AP BQ RQ
= and =
RQ RP AP RP
In the second proportional (but not in the first)
the numerators are sides of the first triangle and the denominators are
sides of the second triangle.
We have therefore to prove that ∆ BQR and ∆ APR are equiangular.
7 BD 2 = BD.BD
If the square of a line segment (say BD 2 ) is equal to the product of two other
line segments (say AD.DC), then BD is a common side of the two triangles
which have to be proved similar.
B
R
Q
A
P
D
C
C
9

5.4 EXAMPLES
Example 1
If we have to prove that BD 2 = AD.DC, we must prove ∆ ABD similar to
∆ BCD ( BD is the common side ) .
Name the similar triangles in the following figures and calculate the values of
c m
a , b , x , y , and .
d n
a) b)
c) d)
L
Solution
A
24
B
a) ∆ AEB ∆DEC ( Â = alternate Dˆ and Bˆ = alternate Ĉ )
Hence
18
E
d
15 30
K
AE
DE
18
30
=
=
a
c
N
EB
EC
15
a
=
C
D
=
b
7,5 P 17,5 S
M
m
M
n
AB
DC
24
b
Hence 18a = 15 × 30 and 18b = 30 × 24
a = 25 b = 40
16
Q
S
24
P
36
x
P
30
21
R
T
y
32
R
T
10

)
∆ PST ∆PQR ( PST ˆ = corresponding Qˆ and Pˆ is common )
Hence
PS
PQ
24
40
=
ST
QR
=
PT
PR
36 30
= = ( cancel where possible )
x 30 + y
Hence 3x = 5 × 36 and 3(30 + y) = 5 × 30
x = 60 y = 20
c) ∆ MPN ∆MLK ( Mˆ is common, NPM ˆ = corresponding Lˆ )
Thus
MP
ML
=
17,5
=
25
c
=
d
PN
LK
c
d
7
10
=
MN
MK
d) ∆ SMP ∆STR ( Sˆ is common, SMP ˆ = corresponding Tˆ )
2.1
Thus
Thus
SM
=
ST
MP
TR
m
=
m + n
21
32
32m = 21(m + n)
32m = 21m + 21n
11m = 21n
m
=
n
21
11
Example 2
In ∆ ACE , FC ˆ BK A ˆ A = and BF CE and ACˆ
K = AFˆ
B .
Proof that :
AB
=
AF
AK
AC
11

2.2
Solution
AC AK.AE
2 =
2.1 In ∆ ABK and ∆ AFC
1. Â is common
2. FC ˆ BK A ˆ A = ( given )
∴ ∆ ABK ∆AFC ( ∠ ∠ ∠ )
∴
AB
=
AF
AK
AC
2.2 In ∆ ACK and ∆ ACE
1. Â is common
2. FB ˆ A ĈK
= A ( given )
= Ê ( corresponding, BF CE )
∴ ∆ ACK ∆AEC ( ∠ ∠ ∠ )
∴
AC
=
AE
E
C
AK
AC
∴ AC AK.AE
2 =
K
F
B
A
12

5.5
Exercise 5
1
2
3
In ∆ PST , TS ⊥ PS en RQ ⊥ PT .
Prove :
1.1 ∆ PRQ ||| ∆ PST
1.2 RQ : PQ = ST : PT
1.3 PR . PT = PQ . PS
In the diagram, PA ║ BC and B 1
∧
Prove that :
2.1 ∆ PAB ||| ∆ ABC
2.2 PA : AB = PB : AC
2.3 AB² = PA . BC
Q
P
= ∧
C .
PQRS is a parallelogram and all the lines are straight lines.
Prove that :
3.1 ∆ TVQ ||| ∆ PVS
PV QV
3.2 =
VU VS
3.3 PV² = TV .VU
P
P
V
R
1 2
B C
1
R
U
2
T
1
2
2
1 1
Q
S
A
T
S
13

4
In the figure, BD ⊥ AC and AB ⊥ BC .
 = 60º .
If AB = 2 units and DC = 3 units,
determine the lengths of AD and BC .
Copyright Mr V Page 14 12/07/2010
A
60°
D
B C
14