Self-Consistent Field Theory and Its Applications by M. W. Matsen

10 1 Self-consistent field theory and its applications
0.3
16
p m
(r) a 3
0.2
0.1
8
m=4
0.0
0.0 0.5 1.0 1.5
r/a
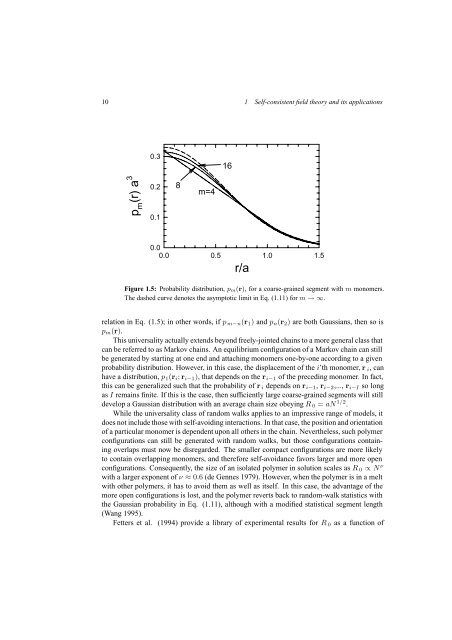
Figure 1.5: Probability distribution, p m(r), for a coarse-grained segment with m monomers.
The dashed curve denotes the asymptotic limit in Eq. (1.11) for m →∞.
relation in Eq. (1.5); in other words, if p m−n (r 1 ) and p n (r 2 ) are both Gaussians, then so is
p m (r).
This universality actually extends beyond freely-jointed chains to a more general class that
can be referred to as Markov chains. An equilibrium configuration of a Markov chain can still
be generated by starting at one end and attaching monomers one-by-one according to a given
probability distribution. However, in this case, the displacement of the i’th monomer, r i , can
have a distribution, p 1 (r i ; r i−1 ), that depends on the r i−1 of the preceding monomer. In fact,
this can be generalized such that the probability of r i depends on r i−1 , r i−2 ,..., r i−I so long
as I remains finite. If this is the case, then sufficiently large coarse-grained segments will still
develop a Gaussian distribution with an average chain size obeying R 0 = aN 1/2 .
While the universality class of random walks applies to an impressive range of models, it
does not include those with self-avoiding interactions. In that case, the position and orientation
of a particular monomer is dependent upon all others in the chain. Nevertheless, such polymer
configurations can still be generated with random walks, but those configurations containing
overlaps must now be disregarded. The smaller compact configurations are more likely
to contain overlapping monomers, and therefore self-avoidance favors larger and more open
configurations. Consequently, the size of an isolated polymer in solution scales as R 0 ∝ N ν
with a larger exponent of ν ≈ 0.6 (de Gennes 1979). However, when the polymer is in a melt
with other polymers, it has to avoid them as well as itself. In this case, the advantage of the
more open configurations is lost, and the polymer reverts back to random-walk statistics with
the Gaussian probability in Eq. (1.11), although with a modified statistical segment length
(Wang 1995).
Fetters et al. (1994) provide a library of experimental results for R 0 as a function of