Self-Consistent Field Theory and Its Applications by M. W. Matsen
Self-Consistent Field Theory and Its Applications by M. W. Matsen
Self-Consistent Field Theory and Its Applications by M. W. Matsen
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
8 1 <strong>Self</strong>-consistent field theory <strong>and</strong> its applications<br />
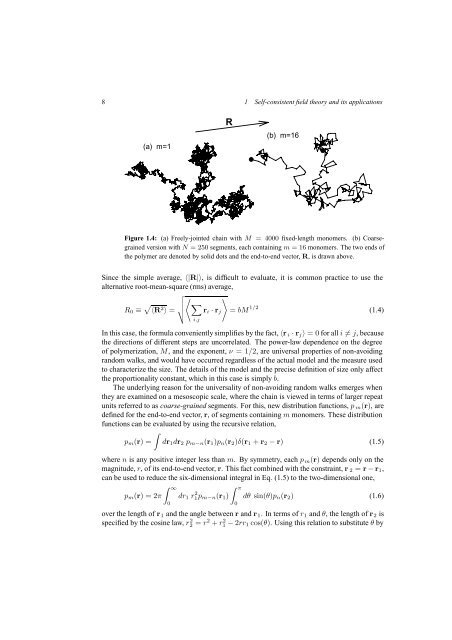
(a) m=1<br />
R<br />
(b) m=16<br />
Figure 1.4: (a) Freely-jointed chain with M = 4000 fixed-length monomers. (b) Coarsegrained<br />
version with N = 250 segments, each containing m =16monomers. The two ends of<br />
the polymer are denoted <strong>by</strong> solid dots <strong>and</strong> the end-to-end vector, R, is drawn above.<br />
Since the simple average, 〈|R|〉, is difficult to evaluate, it is common practice to use the<br />
alternative root-mean-square (rms) average,<br />
R 0 ≡ √ 〈 〉 ∑<br />
〈R 2 〉 = √ r i · r j = bM 1/2 (1.4)<br />
i,j<br />
In this case, the formula conveniently simplifies <strong>by</strong> the fact, 〈r i · r j 〉 =0for all i ≠ j, because<br />
the directions of different steps are uncorrelated. The power-law dependence on the degree<br />
of polymerization, M, <strong>and</strong> the exponent, ν =1/2, are universal properties of non-avoiding<br />
r<strong>and</strong>om walks, <strong>and</strong> would have occurred regardless of the actual model <strong>and</strong> the measure used<br />
to characterize the size. The details of the model <strong>and</strong> the precise definition of size only affect<br />
the proportionality constant, which in this case is simply b.<br />
The underlying reason for the universality of non-avoiding r<strong>and</strong>om walks emerges when<br />
they are examined on a mesoscopic scale, where the chain is viewed in terms of larger repeat<br />
units referred to as coarse-grained segments. For this, new distribution functions, p m (r), are<br />
defined for the end-to-end vector, r, of segments containing m monomers. These distribution<br />
functions can be evaluated <strong>by</strong> using the recursive relation,<br />
∫<br />
p m (r) = dr 1 dr 2 p m−n (r 1 )p n (r 2 )δ(r 1 + r 2 − r) (1.5)<br />
where n is any positive integer less than m. By symmetry, each p m (r) depends only on the<br />
magnitude, r, of its end-to-end vector, r. This fact combined with the constraint, r 2 = r − r 1 ,<br />
can be used to reduce the six-dimensional integral in Eq. (1.5) to the two-dimensional one,<br />
p m (r) =2π<br />
∫ ∞<br />
0<br />
dr 1 r 2 1 p m−n(r 1 )<br />
∫ π<br />
0<br />
dθ sin(θ)p n (r 2 ) (1.6)<br />
over the length of r 1 <strong>and</strong> the angle between r <strong>and</strong> r 1 . In terms of r 1 <strong>and</strong> θ, the length of r 2 is<br />
specified <strong>by</strong> the cosine law, r 2 2 = r2 + r 2 1 − 2rr 1 cos(θ). Using this relation to substitute θ <strong>by</strong>